Un poco de programar videojuegos

Las matemáticas no sirven para nada y tal…

«https://www.raywenderlich.com/35866/trigonometry-for-game-programming-part-1

Trigonometry for Game Programming: Part 1/2

 

 

Does the thought of doing mathematics give you cold sweats? Are you ready to give up on your career as a budding game developer because the math just doesn’t make any sense to you?

Don’t fret – math can be fun, and this cool 2-part game tutorial will back up that claim!

Here’s a little secret: as an app developer, you don’t really need to know a lot of math. If you can add or multiply two numbers together, you’re already halfway there. Most of the computations that we do in our professional lives don’t go much beyond basic arithmetic.

That said, for making games it is useful to have a few more math skills in your toolbox. You don’t need to become as smart as Archimedes or Einstein, but a basic understanding of trigonometry, combined with some common sense, will take you a long way.

In this tutorial, you will learn about some important trigonometric functions and how you can use them in your games. Then you’ll get some practice applying the theories by developing a simple spaces shooter iPhone game that requires a lot of trigonometry, using the Cocos2D game framework.

Don’t worry if you’ve never used Cocos2D before or are going to use a different framework for your game – the mathematics we’ll cover in this tutorial will apply to your engine no matter what. And you don’t need any prior experience, as I’ll walk through the process step-by-step.

If you supply the common sense, this tutorial will get you up to speed on the trig, so let’s get started!

Getting Started: It’s All About Triangles

It sounds like a mouthful, but trigonometry simply means calculations with triangles (that’s where the tricomes from).

You may not have realized it until now, but games are full of triangles. For example, imagine you have a spaceship game, and you want to calculate the distance between these ships:

You have X and Y position of each ship, but how can you find the length of that line?

Well, you can simply draw a line from the center point of each ship to form a triangle like this:

Then, since you know the X and Y coordinates of each ship, you can compute the length of each of the new lines. Now that you know the lengths of two sides of the triangle, you can use some Trigonometry to compute the length of the diagonal line – the distance between the ships.

Note that one of the corners of this triangle has an angle of 90 degrees. This is also known as a right triangle, and that’s the sort of triangle you’ll be dealing with in this tutorial.

Any time you can express something in your game as a triangle with a 90-degree right angle – such as the spatial relationship between the two sprites in the picture – you can use trigonometric functions to do calculations on them.

So in summary, Trigonometry is the mathematics that you use to calculate the lengths of the sides of these triangles, as well as the angles between those sides. And that comes in handy more often that you might think.

For example, in this space ship game you might want to:

• Have one ship shoot a laser in the direction of the other ship
• Have one ship start moving in the direction of another ship to chase
• Play a warning sound effect if an enemy ship is getting too close

All of this and more you can do with the power of Trigonometry!

Your Arsenal of Functions

First, let’s get the theory out of the way. Don’t worry, I’ll keep it short so you can get to the fun coding bits as quickly as possible.

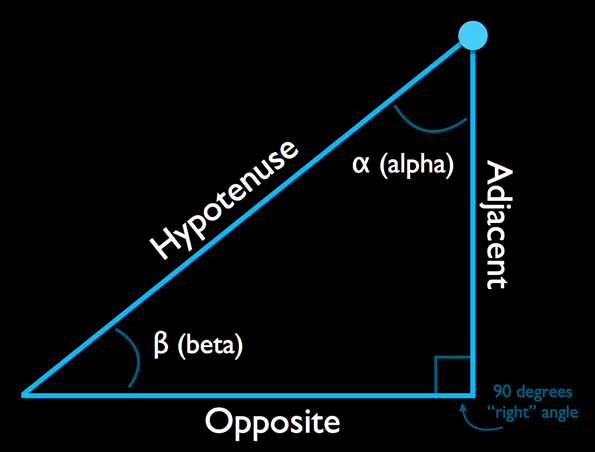
These are the parts that make up a right triangle:

In the picture above, the slanted side is called the hypotenuse. It always sits across from the corner with the 90-degree angle (also called a right angle), and it is always the longest of the three sides.

The two remaining sides are called the adjacent and the opposite, as seen from one particular corner of the triangle, the bottom-left corner in this case.

If you look at the triangle from the other corner (top-right), then the adjacent and opposite change places:

Alpha (α) and beta (β) are the names of the two other angles. You can call these angles anything you want (as long as it sounds Greek!) but usually alpha is the angle in the corner of interest and beta is the angle in the opposing corner. In other words, you label your opposite and adjacent sides with respect to alpha.

The cool thing is that if you only know two of these things, trig allows you to find out all the others using the sine, cosine and tangent functions. For example, if you know an angle and the length of one of the sides, then the sine, cosine and tangent functions can tell you the length of the other sides:

Think of the sin, cos, and tan functions as “black boxes” – you plug in numbers and get back results. They are pre-written functions you can call from almost any programming language.

Know Angle and Length, Need Sides

Let’s consider an example. Say you know the alpha angle between the ships is 45 degrees, and the length between the ships (the hypotenuse) is 10 points long. You can then plug these values into the formula:

sin(45) = opposite / 10

To solve this for the hypotenuse, you shift things around a bit:

opposite = sin(45) * 10

If you call the built-in sin function, you’ll find the sine of 45 degrees is 0.707 (rounded off), and filling that in that gives you the result:

opposite = 0.707 * 10 = 7.07

There is a handy mnemonic for remembering what these functions do that you may remember from high school: SOH-CAH-TOA (where SOH stands for Sine is Opposite over Hypotenuse, and so on), or if you need something more catchy: Some Old Hippy / Caught Another Hippy / Tripping On Acid. (That hippy was probably a mathematician who did a little too much trig.) :]

Know 2 Sides, Need Angle

The above formulas are useful when you already know an angle, but that is not always the case – sometimes it is the angle you are looking for. Then you need to know the lengths of at least two of the sides and plug these into the inverse functions:

• angle = arcsin(opposite/hypotenuse)
• angle = arccos(adjacent/hypotenuse)
• angle = arctan(opposite/adjacent)

In other words, if sin(a) = b, then it is also true that arcsin(b) = a. Of these inverse functions, you will use the arc tangent (arctan) the most in practice. Sometimes these functions are also notated as sin^-1, cos^-1, and tan^-1, so don’t let that fool you.

Is any of this sinking in or sounding familiar? Good, but you’re not done yet with the theory lesson – there is still more that you can calculate with triangles.

Know 2 Sides, Need Remaining Side

Sometimes you may know the length of two of the sides and need to know the length of the third, like in the example at the beginning of this tutorial where you wanted to find the distance between the two space ships.

This is where Trigonometry’s Pythagorean Theorem comes to the rescue. Even if you forgot everything else about math, this is probably the one formula you do remember:

a² + b² = c²

Or, put in terms of the triangle that you saw earlier:

opposite² + adjacent² = hypotenuse²

If you know any two of these sides, then calculating the third is simply a matter of filling in the formula and taking the square root. This is a very common thing to do in games and you’ll be seeing it several times in this tutorial.

Note: Want to drill this formula into your head while having a great laugh at the same time? Search YouTube for “Pythagoras song” – it’s an inspiration for many!

Have Angle, Need Other Angle

Lastly, the angles. If you know one of the non-right angles from the triangle, then figuring out the other ones is a piece of cake. In a triangle, all angles always add up to a total of 180 degrees. Because this is a right triangle, you already know that one of the angles is 90 degrees. That leaves:

alpha + beta + 90 = 180

Or simply:

alpha + beta = 90

The remaining two angles must add up to 90 degrees. So if you know alpha, you also know beta, and vice-versa.

And those are all the formulas you need to know! Which one to use in practice depends on the pieces that you already have. Usually you either have the angle and the length of at least one of the sides, or you don’t have the angle but you do have two of the sides.

Enough theory. Let’s put this stuff into practice.

To Skip, or Not to Skip?

In the next few sections, you will be setting up a basic Cocos2D project with a space ship that can move around the screen using the accelerometer. This won’t involve any trigonometry (yet), so if you already know Cocos2D and feel like this guy:

Then feel free to skip ahead to the Begin the Trigonometry! section below – I have a starter project waiting for you there.

But if you’re the type who likes to code everything from scratch, keep reading! :]

Getting Started

To get started, download the latest version of the Cocos2D v2.1 branch. At the time of writing, this is the “unstable” version cocos2d-iphone-2.1-rc1.

After Cocos2D is done downloading and unzipping, you want to install the project templates. Open a Terminal window to the directory where you downloaded Cocos2D and enter the following command:

./install-templates.sh -f

You should see “Installing cocos2d templates” and a bunch of messages. Now you’re ready to work with Cocos2D.

Fire up Xcode and create a new application from the “cocos2d iOS” template you just installed.

Name the project TrigBlaster and set the device family to iPhone. Build and run the template as-is. If all works OK, you should see the following:

You are going to use ARC in this project, but Cocos2D projects have it disabled by default. Luckily, enabling ARC is really easy. Just go to Edit\Refactor\Convert to Objective-C ARC. Expand the drop-down and select only the last four files (main.m, AppDelegate.m, HelloWorldLayer.m, and IntroLayer.m), then click Check and finish the steps in the wizard.

Note: When you convert a project to ARC or make other large changes, Xcode will prompt you to enable snapshots for the current project. A snapshot saves the state of the entire project as if you copied and pasted the project into another directory. You can learn more about snapshots over here. For the purposes of this tutorial, select disable.

Next download the resources for this tutorial. This file contains the images for the sprites and the sound effects. Unzip it and drag the Images and Sounds folders into Xcode to add them to the project. In the import dialog, make sure Copy items into destination group’s folder is checked.

Great, the preliminaries are over and done with – now let’s get coding for real.

Steering with Accelerometers

Because this is a simple game, you will be doing all your work inside a single class: the HelloWorldLayer. Right now, this layer contains a bunch of stuff that you don’t need.

Replace the contents of HelloWorldLayer.h with:

#import "cocos2d.h"   @interface HelloWorldLayer : CCLayerColor   + (CCScene *)scene;   @end

This game doesn’t use GameKit, so you got rid of that. You also changed HelloWorldLayer from a plain CCLayerto a CCLayerColor so that you can have a background color other than just black.

There is also a bunch of code in the .m file that you don’t need for this game. Replace HelloWorldLayer.m with:

#import "HelloWorldLayer.h" #import "SimpleAudioEngine.h"   @implementation HelloWorldLayer   + (CCScene *)scene { CCScene *scene = [CCScene node]; HelloWorldLayer *layer = [HelloWorldLayer node]; [scene addChild:layer]; return scene; }   - (id)init { if ((self = [super initWithColor:ccc4(94, 63, 107, 255)])) { } return self; }   @end

The scene method is unchanged from the template, but init now calls [super initWithColor] to set a purple background color on the layer.

Build and run, and you should see nothing but purple:

Let’s make things a bit more exciting by adding a spaceship to the scene. You will need some instance variables for this, so modify the @implementation line in HelloWorldLayer.m as follows:

@implementation HelloWorldLayer { CGSize _winSize; CCSprite *_playerSprite; }

The _winSize variable stores the dimensions of the screen, which is useful because you will be referring to that quite often. The _playerSprite variable holds the spaceship sprite.

Now change init to the following:

- (id)init { if ((self = [super initWithColor:ccc4(94, 63, 107, 255)])) { _winSize = [CCDirector sharedDirector].winSize;   _playerSprite = [CCSprite spriteWithFile:@"Player.png"]; _playerSprite.position = ccp(_winSize.width - 50.0f, 50.0f); [self addChild:_playerSprite]; } return self; }

This is all pretty basic if you have worked with Cocos2D before. The player sprite is positioned in the bottom-right corner of the screen. Remember that with Cocos2D, it is the bottom of the screen that has y-coordinate 0, unlike in UIKit, where y = 0 points to the top of the screen.

Build and run to try it out, and you should see the following:

To move the spaceship, this game uses the iPhone’s built-in accelerometers. Unfortunately, the iOS Simulator cannot simulate the accelerometers. That means that from now on, you will need to run the app on your device to test it.

Note: If you are unsure how to put the app on your device, check out this extensive tutorial that explains how to obtain and install the certificates and provisioning profiles that allow Xcode to run apps on your device. It’s not as intimidating as it looks, but you do need to sign up for the paid Apple developer program.

To move the spaceship with the accelerometers, you’ll obviously be rocking your device from side to side. During testing I found that this sometimes caused the screen to autorotate from landscape right to landscape left, which is really annoying when you’re in the middle of a heated battle.

To prevent that from happening, go into the Target Settings screen and in the Summary tab under Supported Interface Orientations, de-select all options except for Landscape Right.

Using the accelerometers from code is pretty straightforward. You first ask Cocos2D to activate the accelerometers. Once enabled, it sends you periodic updates with the values of the three accelerometers that are built into the iPhone. You then use these values to change the speed of the spaceship.

First, add new instance variables to keep track of the accelerometer values. You only store the values for two of the accelerometers; the “Z” one isn’t used by this game.

@implementation HelloWorldLayer { . . . UIAccelerationValue _accelerometerX; UIAccelerationValue _accelerometerY; }

A good place to activate the accelerometers is inside init. Add the following line to it:

self.accelerometerEnabled = YES;

Finally, add the method that receives the accelerometer values:

- (void)accelerometer:(UIAccelerometer *)accelerometer didAccelerate:(UIAcceleration *)acceleration { const double FilteringFactor = 0.75;   _accelerometerX = acceleration.x * FilteringFactor + _accelerometerX * (1.0 - FilteringFactor); _accelerometerY = acceleration.y * FilteringFactor + _accelerometerY * (1.0 - FilteringFactor); }

This bit of logic is necessary to filter – or “smoothen” – the data that you get back from the accelerometers so that it appears less jittery.

Note: An accelerometer records how much gravity currently pulls on it. Because the user is holding the iPhone in her hands, and hands are never completely steady, there are a lot of tiny fluctuations in this gravity value. We are not so much interested in these unsteady motions as in the larger changes in orientation that the user makes to the device. By applying this simple low-pass filter, you retain this orientation information but filter out the less important fluctuations.

Now that you have a stable measurement of the device’s orientation, how can you use this to make the player’s spaceship move?

Movement in games often works like this:

1. Set the acceleration based on some form of user input, in this case from the accelerometer values.
2. Add the new acceleration to the spaceship’s current speed. This makes the object speed up or slow down, depending on the direction of the acceleration.
3. Add the new speed to the spaceship’s position to make it move.

Of course, you have a great mathematician to thank for these equations: Sir Isaac Newton!

You need some more instance variables to pull this off. There is no need to keep track of the player’s position because the CCSprite already does that for you, but acceleration and speed are your own responsibility.

Add these instance variables:

@implementation HelloWorldLayer { . . . float _playerAccelX; float _playerAccelY; float _playerSpeedX; float _playerSpeedY; }

It’s good to set some bounds on how fast the spaceship can travel or it would be pretty hard to maneuver. Infinite acceleration sounds like a good idea in theory but in practice it doesn’t work out so well (besides, even Einstein thinks there are limits to how fast you can go).

Add the following lines directly below the #import statement:

const float MaxPlayerAccel = 400.0f; const float MaxPlayerSpeed = 200.0f;

This defines two constants, the maximum acceleration (400 points per second squared) and the maximum speed (200 points per second).

Now add the following bit of logic to the bottom of accelerometer:didAccelerate::

if (_accelerometerY > 0.05) { _playerAccelX = -MaxPlayerAccel; } else if (_accelerometerY < -0.05) { _playerAccelX = MaxPlayerAccel; } if (_accelerometerX < -0.05) { _playerAccelY = -MaxPlayerAccel; } else if (_accelerometerX > 0.05) { _playerAccelY = MaxPlayerAccel; }

This is a basic technique for controlling a sprite using the accelerometers. When the device is tilted to the left, you give the player maximum acceleration to the left. Conversely, when the device is tilted to the right, you give the player maximum acceleration to the right. Likewise for the up and down directions.

Note: You’re using the _accelerometerY value for the x-direction. That’s as it should be. Remember that this game is in landscape, so the Y-accelerometer runs from left to right in this orientation, and the X-accelerometer from top to bottom.

You’re almost there. The last step is applying the _playerAccelX and Y values to the speed and position of the spaceship. You will do this in the game’s update method. This method is called 60 times per second, so it’s the natural place to perform all of the game logic.

Add updatePlayer to the class:

- (void)updatePlayer:(ccTime)dt { // 1 _playerSpeedX += _playerAccelX * dt; _playerSpeedY += _playerAccelY * dt;   // 2 _playerSpeedX = fmaxf(fminf(_playerSpeedX, MaxPlayerSpeed), -MaxPlayerSpeed); _playerSpeedY = fmaxf(fminf(_playerSpeedY, MaxPlayerSpeed), -MaxPlayerSpeed);   // 3 float newX = _playerSprite.position.x + _playerSpeedX*dt; float newY = _playerSprite.position.y + _playerSpeedY*dt;   // 4 newX = MIN(_winSize.width, MAX(newX, 0)); newY = MIN(_winSize.height, MAX(newY, 0));   _playerSprite.position = ccp(newX, newY); }

If you’ve programmed games before (or studied physics), then this should look very familiar. Here’s how it works:

1. Add the current acceleration to the speed.The acceleration is expressed in points per second (actually, per second squared, but don’t worry about that) but update: is performed a lot more often than once per second. To compensate for this difference, you multiply the acceleration by the elapsed or “delta” time, dt. Without this, the spaceship would move about sixty times faster than it should!
2. Clamp the speed so that it doesn’t go faster than MaxPlayerSpeed if it is positive or -MaxPlayerSpeed if it is negative. You could write this using an if statement instead:

   if (_playerSpeedX < -MaxPlayerSpeed) { _playerSpeedX = -MaxPlayerSpeed; } else if (_playerSpeedX > MaxPlayerSpeed) { _playerSpeedX = MaxPlayerSpeed; }

   However, I like the succinctness of the one-line version. fminf() makes sure the speed doesn’t become larger than MaxPlayerSpeed because it always picks the smallest of the two, while fmaxf() makes sure the speed doesn’t drop below -MaxPlayerSpeed because it always picks the largest value. It’s a neat little trick.

3. Add the current speed to the sprite’s position. Again, speed is measured in points per second, so you need to multiply it by the delta time to make it work correctly.
4. Clamp the new position to the sides of the screen. You don’t want the player’s spaceship to go offscreen, never to be found again!

Finally, add the following line to init to make update active:

[self scheduleUpdate];

Then add update itself:

- (void)update:(ccTime)dt { [self updatePlayer:dt]; }

That should do it. Build and run the game. You can now control the spaceship by tilting the device.

Begin the Trigonometry!

If you skipped ahead to this section, here is the starter project at this point. Build and run it on your device – you’ll see there’s a spaceship that you can move around with the accelerometer.

You haven’t seen any trigonometry yet, so let’s put some into action.

Note: If you’ve used Cocos2D before, you might know Cocos2D comes with handy built-in methods like ccpLength, ccpAngle, and more that are wrappers over the raw trigonometry functions for ease of use. In this tutorial, you will not be using these functions – instead you will be going low level and issuing the raw trigonometry calls yourself, for the learning experience. In an actual game, you might want to use the wrapper methods however.

It would be cool – and much less confusing to the player! – to rotate the spaceship in the direction it is currently moving rather than having it always pointing upward.

To rotate the spaceship, you need to know the angle to rotate it. But you don’t know what that is, so how can you figure that out?

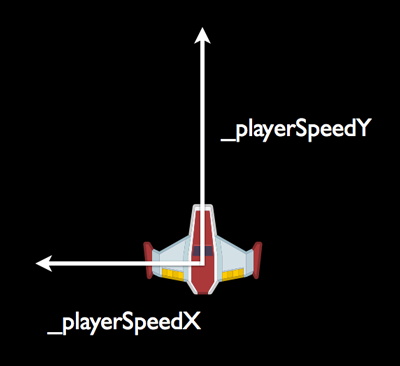
Let’s think about what you do know. You do have the player’s speed, which consists of two components: a speed in the x-direction and a speed in the y-direction:

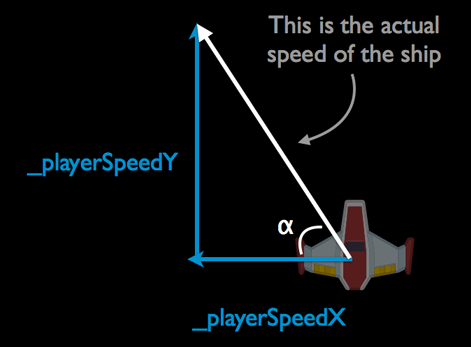
If you rearrange these a little, you can see that they form a triangle:

Here you know the lengths of the adjacent (_playerSpeedX) and the opposite (_playerSpeedY) sides.

So basically, you know 2 sides of a right triangle, so you are in the Know 2 Sides, Need Angle case mentioned in the beginning of this tutorial. That means you need to use one of the inverse functions: arcsin, arccos or arctan.

You know the opposite and adjacent sides, so you want to use the arctan function to find the angle to rotate the ship. Remember, that looks like the following:

angle = arctan(opposite / adjacent)

The Objective-C math library comes with the atan() function that computes the arc tangent, but it has one big issue: what if the x-speed is 0? In that case, the adjacent is 0 and dividing by 0 is mathematically undefined. Your app might crash or just behave weirdly when that happens.

Instead of using atan(), it is better to use the function atan2(), which takes two parameters and correctly handles the division-by-zero scenario without you having to worry about it:

angle = atan2(opposite, adjacent)

Important: The angle that atan2f() gives you is not the inner angle inside the triangle, but the angle that the hypotenuse makes with that 0-degree line:

This is another reason why atan2() is a lot more useful than plain, old atan().

So let’s give that a shot. Add the following two lines to the bottom of updatePlayer:

float angle = atan2f(_playerSpeedY, _playerSpeedX); _playerSprite.rotation = angle;

Note that the arctan function you are using is actually called atan2f() instead of just atan2(). More about that in a moment. Also notice that the y-coordinate goes first. A common mistake is to write atan2f(x, y) but that’s the wrong way around. Remember the first parameter is the opposite, and in this case the Y coordinate is the opposite.

Build and run the app to try it out:

Hmm, this doesn’t seem to be working quite right. What is wrong here?

Radians vs. Degrees

Normal human beings tend to think of angles as values between 0 and 360. Mathematicians, however, measure angles in radians, which are expressed in terms of π (the Greek letter Pi, which sounds like pie but doesn’t taste as good).

It’s not essential to understand, but if you’re curious, one radian is the angle you get when you travel the distance of the radius along the arc of the circle. You can do that 2π times (roughly 6.28 times) before you end up at the beginning of the circle again.

So while you may think of angles as values from 0 to 360, a mathematician sees values from 0 to 2π. And that’s where your problem lies. atan2f() returns a value in radians but Cocos2D’s sprite rotation property expects degrees. That’s why you only saw the sprite rotate a little: angles measured in radians are much smaller than angles measured in degrees.

Fortunately, it is easy to convert from one to the other. Change the line that sets _playerSprite.rotation to:

_playerSprite.rotation = CC_RADIANS_TO_DEGREES(angle);

The CC_RADIANS_TO_DEGREES macro is Cocos2D’s way of doing the conversion but you can easily write your own function or macro to do this. I don’t have these formulas memorized but they are easy enough to derive yourself when you realize than an angle of 360 degrees corresponds to 2π:

Angle in degrees / 360 degrees = Angle in radians / 2π radians

To go from radians to degrees, the formula becomes:

Angle in degrees = (Angle in radians / 2π) * 360

And the other way around, from degrees to radians:

Angle in radians = (Angle in degrees / 360) * 2π

I point this out because forgetting to convert between radians and degrees is probably the most common mistake programmers make when they are dealing with trigonometry! So if you don’t see the rotation you expected, make sure you’re not mixing up your degrees and radians…

So what the @#! is π? Pi is the ratio of the circumference of a circle to its diameter. In other words, if you measure the outside of any circle and divide it by its diameter (which is twice the radius), you get the number 3.141592… and that is what we call π. It takes 2π radians to go all the way around a circle, which is why 360 degrees corresponds to 2π. Pi is a very important concept in mathematics that shows up all the time, especially with anything that is cyclical.

By the way, not everyone likes π. Some people believe that it would have been better to choose the ratio of the circumference to the radius, instead of to the diameter. This number is called tau (τ) and is equal to 2π, thus simplifying many of the calculations. You can read more about tau at tauday.com.

Anyway, back to the game. Build and run to see some glorious rotation in action.

Whoops, something is still not right. The spaceship certainly rotates but it seems to be pointing in the direction opposite to where it’s flying!

Here’s what’s happening: the sprite for the spaceship points straight up, which corresponds to the default rotation value of 0 degrees. But in mathematics, an angle of 0 degrees (or radians) doesn’t point upward, but to the right:

And that’s not the only problem: in Cocos2D, rotation happens in a clockwise direction, but in mathematics it goes counterclockwise.

To overcome these differences, change the line to:

_playerSprite.rotation = 90.0f - CC_RADIANS_TO_DEGREES(angle);

This adds 90 degrees to make the sprite point to the right at an angle of 0 degrees, so that it lines up with the way atan2f() does things. Then it adds the negative angle – in other words, subtracts the angle – in order to rotate the proper way around.

Build and run once more to try it out. You’ll finally have a spaceship that has its head on straight!

Bouncing Off the Walls

You have a spaceship that you can move using the accelerometers and you’re using trig to make sure it points in the direction it’s flying. That’s a good start.

Unfortunately, having the spaceship get stuck on the edges of the screen isn’t very satisfying. You’re going to fix that by making it bounce off the screen borders instead.

First, comment these lines from updatePlayer:

//newX = MIN(_winSize.width, MAX(newX, 0)); //newY = MIN(_winSize.height, MAX(newY, 0));

Then add the following code to updatePlayer, just after the commented lines:

BOOL collidedWithVerticalBorder = NO; BOOL collidedWithHorizontalBorder = NO;   if (newX < 0.0f) { newX = 0.0f; collidedWithVerticalBorder = YES; } else if (newX > _winSize.width) { newX = _winSize.width; collidedWithVerticalBorder = YES; }   if (newY < 0.0f) { newY = 0.0f; collidedWithHorizontalBorder = YES; } else if (newY > _winSize.height) { newY = _winSize.height; collidedWithHorizontalBorder = YES; }

This checks whether the spaceship hit any of the screen borders and if so, sets a BOOL variable to YES. But what to do after such a collision takes place? To make the spaceship bounce off the border, you can simply reverse its speed and acceleration.

First, define a constant at the top of the file, above the @implementation line:

const float BorderCollisionDamping = 0.4f;

Add the following lines in updatePlayer, directly below the code you last added there:

if (collidedWithVerticalBorder) { _playerAccelX = -_playerAccelX * BorderCollisionDamping; _playerSpeedX = -_playerSpeedX * BorderCollisionDamping; _playerAccelY = _playerAccelY * BorderCollisionDamping; _playerSpeedY = _playerSpeedY * BorderCollisionDamping; }   if (collidedWithHorizontalBorder) { _playerAccelX = _playerAccelX * BorderCollisionDamping; _playerSpeedX = _playerSpeedX * BorderCollisionDamping; _playerAccelY = -_playerAccelY * BorderCollisionDamping; _playerSpeedY = -_playerSpeedY * BorderCollisionDamping; }

If a collision registered, you flip the acceleration and speed values around. Notice that this also multiplies the acceleration and speed by a damping value, BorderCollisionDamping.

As usually happens in a collision, some of the movement energy is dissipated by the impact. In this case, you make the spaceship retain only 40% of its speed after bumping into the screen edges.

Try it out. Smash the spaceship into the border and see what happens. Who said spaceships can’t bounce?

For fun, play with the value of BorderCollisionDamping to see the effect of different values for this constant. If you make it larger than 1.0f, the spaceship actually gains energy from the collision.

Note: Now why is there an f behind those numbers in the code: 0.4f, 0.1f, 0.0f, and so on? And why did you use atan2f() instead of just atan2()? When you write games, you want to work with floating point numbers as much as possible because, unlike integers, they allow for digits behind the decimal point. This allows you to be much more precise.

There are two types of floating point numbers: floats and doubles (there is also a “long double”, but that’s the same as a double on the iPhone). Doubles are more precise than floats but they also take up more memory and are slower in practice. When you don’t put the f behind the number and just use 0.4, 0.1, 0.0, or when you use the version of a math function without the f suffix, you are working with doubles and not floats.

It doesn’t really matter if you use a double here and there. For example, the time value that CACurrentMediaTime() returns is a double. However, if you’re doing hundreds of thousands of calculations every frame, you will notice the difference. I did a quick test on a couple of my devices and the same calculations using doubles were 1.5 to 2 times slower. So it’s a good habit to stick to regular floats where you can.

You may have noticed a slight problem. Keep the spaceship aimed at the bottom of the screen so that it continues smashing into the border over and over, and you’ll see a constant flicker between the up and down angles.

Using the arc tangent to find the angle between a pair of x- and y-components works quite well, but only if those x and y values are fairly large. In this case, the damping factor has reduced the speed to almost zero. When you apply atan2f() to very small values, even a tiny change in these values can result in a big change in the resulting angle.

One way to fix this is to not change the angle when the speed is very slow. That sounds like an excellent reason to give a call to our old friend, Pythagoras.

Right now you don’t have such a thing as “the ship’s speed”. Instead, you have two speeds: one in the x-direction and one in the y-direction. But in order to draw any conclusions about “the ship’s speed” – is it too slow to actually rotate the ship? – you need to combine these x and y speed values into one single value:

Here you are in the Know 2 Sides, Need Remaining Side case discussed earlier.

As you can see, the true speed of the spaceship, that is, how many points it moves across the screen in a second, is the hypotenuse of the triangle that is formed by the speed in the x-direction and the speed in the y-direction. Put in terms of the Pythagorean formula:

true speed² = _playerSpeedX² + _playerSpeedY²

To find the actual value, you need to take the square root:

true speed = √(_playerSpeedX² + _playerSpeedY²)

Add this code to updatePlayer. First remove this block of code:

float angle = atan2f(_playerSpeedY, _playerSpeedX); _playerSprite.rotation = 90.0f - CC_RADIANS_TO_DEGREES(angle);

And replace it with this block of code:

float speed = sqrtf(_playerSpeedX*_playerSpeedX + _playerSpeedY*_playerSpeedY); if (speed > 40.0f) { float angle = atan2f(_playerSpeedY, _playerSpeedX); _playerSprite.rotation = 90.0f - CC_RADIANS_TO_DEGREES(angle); }

Build and run. You’ll see the spaceship now acts a lot more stable at the edges of the screen. If you’re wondering where the value 40.0f came from, the answer is: experimentation. I put some NSLog() statements into the code to look at the speeds at which the craft typically hit the borders, and then I tweaked this value until it felt right.

Blending Angles for Smooth Rotation

Of course, fixing one thing always breaks something else. Try slowing down the spaceship until it has stopped, then flip the device so the spaceship has to turn around and fly the other way.

Previously, that happened with a nice animation where you actually saw the ship turning. But because you just added some code that prevents the ship from changing its angle at low speeds, the turn is now very abrupt. It’s only a small detail, but it is the details that make great products.

The fix is to not switch to the new angle immediately, but to gradually “blend” it with the previous angle over a series of successive frames. This re-introduces the turning animation and still prevents the ship from rotating when it is not moving fast enough.

Blending sounds fancy, but it’s actually quite easy to implement. It does require you to keep track of the spaceship’s angle between updates, so add a new instance variable for it in the implementation block in HelloWorldLayer.m:

@implementation HelloWorldLayer { ... float _playerAngle; }

Change the rotation code in updatePlayer from this:

float speed = sqrtf(_playerSpeedX*_playerSpeedX + _playerSpeedY*_playerSpeedY); if (speed > 40.0f) { float angle = atan2f(_playerSpeedY, _playerSpeedX); _playerSprite.rotation = 90.0f - CC_RADIANS_TO_DEGREES(angle); }

To this:

float speed = sqrtf(_playerSpeedX*_playerSpeedX + _playerSpeedY*_playerSpeedY); if (speed > 40.0f) { float angle = atan2f(_playerSpeedY, _playerSpeedX);   const float RotationBlendFactor = 0.2f; _playerAngle = angle * RotationBlendFactor + _playerAngle * (1.0f - RotationBlendFactor); }   _playerSprite.rotation = 90.0f - CC_RADIANS_TO_DEGREES(_playerAngle);

The _playerAngle variable combines the new angle and its own previous value by multiplying them with a blend factor. In human-speak, this means the new angle only counts for 20% towards the actual rotation that you set on the spaceship. Of course, over time more and more of the new angle gets added so that eventually the spaceship does point in the proper direction.

Note that the line that sets the _playerSprite’s rotation property is now outside the if statement.

Build and run to verify that there is no longer an abrupt change from one rotation angle to another.

Now try flying in a circle a couple of times, both clockwise and counterclockwise. You’ll notice that at some point in the turn the spaceship suddenly spins in the opposite direction. It always happens at the same point in the circle. What’s going on?

Well, there is something you should know about atan2f(). It does not return an angle in the convenient range of 0 to 360 degrees, but a value between +π and –π radians, or between +180 and -180 degrees to us non-mathematicians:

That means if you’re turning counterclockwise, at some point the angle will jump from +180 degrees to -180 degrees; or the other way around if you’re turning clockwise. And that’s where the weird spinning effect happens.

The problem is that when the new angle jumps from 180 degrees to -180 degrees, _playerAngle is still positive because it is trailing behind a bit. When you blend these two together, the spaceship actually starts turning the other way around. It took me a while to figure out what was causing this!

To fix it, you need to recognize when the angle makes that jump and adjust _playerAngle accordingly. Add a new instance variable at the bottom of the HelloWorldLayer implementation block:

@implementation HelloWorldLayer { ... float _lastAngle; }

And change the rotation code one more time from this:

float speed = sqrtf(_playerSpeedX*_playerSpeedX + _playerSpeedY*_playerSpeedY); if (speed > 40.0f) { float angle = atan2f(_playerSpeedY, _playerSpeedX);   const float RotationBlendFactor = 0.2f; _playerAngle = angle * RotationBlendFactor + _playerAngle * (1.0f - RotationBlendFactor); }   _playerSprite.rotation = 90.0f - CC_RADIANS_TO_DEGREES(_playerAngle);

To this:

float speed = sqrtf(_playerSpeedX*_playerSpeedX + _playerSpeedY*_playerSpeedY); if (speed > 40.0f) { float angle = atan2f(_playerSpeedY, _playerSpeedX);   // Did the angle flip from +Pi to -Pi, or -Pi to +Pi? if (_lastAngle < -3.0f && angle > 3.0f) { _playerAngle += M_PI * 2.0f; } else if (_lastAngle > 3.0f && angle < -3.0f) { _playerAngle -= M_PI * 2.0f; }   _lastAngle = angle; const float RotationBlendFactor = 0.2f; _playerAngle = angle * RotationBlendFactor + _playerAngle * (1.0f - RotationBlendFactor); }   _playerSprite.rotation = 90.0f - CC_RADIANS_TO_DEGREES(_playerAngle);

Build and run. That’ll fix things right up and you should have no more problems turning your spacecraft!

This may have seemed like an arcane little problem not worth so much time, but it’s good to be aware that atan2f() gives you angles from -180 degrees (or –π radians) to +180 degrees (or π radians). If you’re using arc tangent to calculate an angle and you get weird behavior like the spinning you saw here, then you might need to verify that your angles really are what you expect them to be.

Using Trig to Find Your Target

This is a great start – you have a spaceship moving along pretty smoothly! But so far the spaceship’s life is too easy and carefree. Let’s spice this up by adding an enemy: a big cannon!

Add two new instance variables in the implementation block in HelloWorldLayer.m:

@implementation HelloWorldLayer { ... CCSprite *_cannonSprite; CCSprite *_turretSprite; }

You’ll set these sprites up in init. Place this code before the creation of _playerSprite so that the spaceship always gets drawn “above” the cannon:

_cannonSprite = [CCSprite spriteWithFile:@"Cannon.png"]; _cannonSprite.position = ccp(_winSize.width/2.0f, _winSize.height/2.0f); [self addChild:_cannonSprite];   _turretSprite = [CCSprite spriteWithFile:@"Turret.png"]; _turretSprite.position = ccp(_winSize.width/2.0f, _winSize.height/2.0f); [self addChild:_turretSprite];

The cannon consists of two sprites: the fixed base and the turret that can rotate to take aim at the player. Build and run, and you should have a brand-new cannon sitting smack in the middle of the screen.

Now you’ll give the cannon a target to snipe at – I bet you know who!

Yep, its turret should point at the player at all times. To get this to work, you need to figure out the angle to rotate the turret so that it points toward the player.

Figuring this out will be very similar to how you figured out how to rotate the spaceship in order to point toward the direction it’s moving in. The difference is that this time, the triangle won’t be based on the speed of the spaceship. Instead it will be drawn between the center positions of the two sprites:

Again, you can use atan2f() to calculate this angle. Add the following method:

- (void)updateTurret:(ccTime)dt { float deltaX = _playerSprite.position.x - _turretSprite.position.x; float deltaY = _playerSprite.position.y - _turretSprite.position.y;   float angle = atan2f(deltaY, deltaX); _turretSprite.rotation = 90.0f - CC_RADIANS_TO_DEGREES(angle); }

The deltaX and deltaY variables measure the distance between the player sprite and the turret sprite. You plug these values into atan2f() to get angle of rotation.

As before, you need to convert this angle to degrees and adjust for the differences between mathematics and Cocos2D. Remember that atan2() always gives you the angle between the hypotenuse and the 0-degree line. It is not the angle inside the triangle.

Don’t forget to call this new updateTurret: method from update, or nothing much will happen:

- (void)update:(ccTime)dt { [self updatePlayer:dt]; [self updateTurret:dt]; }

Build and run. The cannon is now always pointing at the spaceship. See how easy that was? That’s the power of trig for you!

Challenge: It is unlikely that a real cannon would be able to act so instantaneously – it would have to be able to predict exactly where the target was going. Instead, a real cannon would always be playing catch up.

You can accomplish this by “blending” the old angle with the new one, just like you did earlier. The smaller the blend factor, the more time the turret needs to catch up with the spaceship. See if you can implement this on your own.

Adding Health Bars… That Move!

Soon the player will be able to fire missiles at the cannon, and the cannon will be able to inflict damage on the player. To show the amount of hit points each object has remaining, you will add health bars to the scene using CCDrawNode, a new feature of Cocos2D v2.1.

First, you have to do some prep. Add a couple of new constants to the top of the file:

const int MaxHP = 100; const float HealthBarWidth = 40.0f; const float HealthBarHeight = 4.0f;

Also add a couple of new instance variables in the implementation block:

@implementation HelloWorldLayer { ... int _playerHP; int _cannonHP; CCDrawNode *_playerHealthBar; CCDrawNode *_cannonHealthBar; }

Add the following to the bottom of init to set up these new variables:

_playerHealthBar = [[CCDrawNode alloc] init]; _playerHealthBar.contentSize = CGSizeMake(HealthBarWidth, HealthBarHeight); [self addChild:_playerHealthBar];   _cannonHealthBar = [[CCDrawNode alloc] init]; _cannonHealthBar.contentSize = CGSizeMake(HealthBarWidth, HealthBarHeight); [self addChild:_cannonHealthBar];   _cannonHealthBar.position = ccp( _cannonSprite.position.x - HealthBarWidth/2.0f + 0.5f, _cannonSprite.position.y - _cannonSprite.contentSize.height/2.0f - 10.0f + 0.5f);   _playerHP = MaxHP; _cannonHP = MaxHP;

The _playerHealthBar and _cannonHealthBar objects are instances of CCDrawNode, which is like a sprite except that you can draw arbitrary shapes into it. That is ideal for your health bars, which consist of a border and a filled rectangle.

Note that you placed the _cannonHealthBar sprite slightly below the cannon, but didn’t assign a position to the _playerHealthBar yet. That’s because the cannon never moves, so you can simply set the position of its health bar once and forget about it.

However, whenever the spaceship moves, you have to adjust the position of the _playerHealthBar as well. That happens in updatePlayer. Add the following lines to the bottom of that method:

_playerHealthBar.position = ccp( _playerSprite.position.x - HealthBarWidth/2.0f + 0.5f, _playerSprite.position.y - _playerSprite.contentSize.height/2.0f - 15.0f + 0.5f);

Now all that’s left is drawing the bars themselves. Add this new method to the bottom of the file:

- (void)drawHealthBar:(CCDrawNode *)node hp:(int)hp { [node clear];   CGPoint verts[4]; verts[0] = ccp(0.0f, 0.0f); verts[1] = ccp(0.0f, HealthBarHeight - 1.0f); verts[2] = ccp(HealthBarWidth - 1.0f, HealthBarHeight - 1.0f); verts[3] = ccp(HealthBarWidth - 1.0f, 0.0f);   ccColor4F clearColor = ccc4f(0.0f, 0.0f, 0.0f, 0.0f); ccColor4F fillColor = ccc4f(113.0f/255.0f, 202.0f/255.0f, 53.0f/255.0f, 1.0f); ccColor4F borderColor = ccc4f(35.0f/255.0f, 28.0f/255.0f, 40.0f/255.0f, 1.0f);   [node drawPolyWithVerts:verts count:4 fillColor:clearColor borderWidth:1.0f borderColor:borderColor];   verts[0].x += 0.5f; verts[0].y += 0.5f; verts[1].x += 0.5f; verts[1].y -= 0.5f; verts[2].x = (HealthBarWidth - 2.0f)*hp/MaxHP + 0.5f; verts[2].y -= 0.5f; verts[3].x = verts[2].x; verts[3].y += 0.5f;   [node drawPolyWithVerts:verts count:4 fillColor:fillColor borderWidth:0.0f borderColor:clearColor]; }

This sets up an array of points for the corners of the rectangle that you’ll be drawing, then draws it twice: once for the border, which always has the same size, and once for the amount of health, which changes depending on the number of remaining hit points.

Of course, you need to call this method, once for the player and once for the cannon. Add these two lines to update:

[self drawHealthBar:_playerHealthBar hp:_playerHP]; [self drawHealthBar:_cannonHealthBar hp:_cannonHP];

Build and run. Now both the player and the enemy have health bars:

Using Trig for Collision Detection

Right now, the spaceship can fly directly over the cannon without consequence. It would be more fun if it didn’t have it so easy and instead suffered damage for colliding with the cannon. This is where this tutorial enters the sphere of collision detection (don’t miss that pun).

At this point, a lot of game devs think, “I need a physics engine!” and reach for Box2D. While it’s certainly true that you can use Box2D for this, it’s not that hard to do collision detection yourself, especially when you can use circles.

Detecting whether two circles intersect is a piece of cake: all you have to do is look at the distance between them (*cough* Pythagoras) and see if it is smaller than the radius of both circles.

Add two new constants to the top of HelloWorldLayer.m:

const float CannonCollisionRadius = 20.0f; const float PlayerCollisionRadius = 10.0f;

These are the sizes of the collision circles around the cannon and the player. Looking at the sprite, you’ll see that the radius of the cannon is slightly larger at 25 points, but it’s nice to have a bit of wiggle room. You don’t want your games to be too unforgiving or players will think it is unfair.

The fact that the spaceship isn’t circular at all shouldn’t deter you. Often a circle is a good enough approximation of the shape of your sprite, and it has the big advantage that it makes it easy to do the necessary calculations. In this case, the body of the ship is roughly 20 points in diameter (remember, the diameter is twice the radius).

Add a new method that does the collision detection:

- (void)checkCollisionOfPlayerWithCannon { float deltaX = _playerSprite.position.x - _turretSprite.position.x; float deltaY = _playerSprite.position.y - _turretSprite.position.y;   float distance = sqrtf(deltaX*deltaX + deltaY*deltaY);   if (distance <= CannonCollisionRadius + PlayerCollisionRadius) { [[SimpleAudioEngine sharedEngine] playEffect:@"Collision.wav"]; } }

You’ve seen how this works before: first you calculate the distance between the x-positions of the two sprites, then the y-distance. With these two values, you can calculate the hypotenuse, which is the true distance between these sprites. If that distance is smaller than the collision circles, a sound effect is played.

In update, add a call to this new method, above the calls to drawHealthBar:

[self checkCollisionOfPlayerWithCannon];

Also add this line to init to pre-load the sound effect:

[[SimpleAudioEngine sharedEngine] preloadEffect:@"Collision.wav"];

Give it a whirl and drive the spaceship into the cannon.

Notice that the sound effect is a little odd. It’s because while the spaceship flies over the cannon, the game registers repeated collisions, one after another. There isn’t just one collision, there are many, and it plays the sound effect for every one of them.

Collision detection is only the first step. The second step is collision response. Not only do you want to play a sound effect, but you also want the spaceship to bounce off the cannon.

Add these lines inside the if statement in checkCollisionOfPlayerWithCannon:

const float CannonCollisionDamping = 0.8f; _playerAccelX = -_playerAccelX * CannonCollisionDamping; _playerSpeedX = -_playerSpeedX * CannonCollisionDamping; _playerAccelY = -_playerAccelY * CannonCollisionDamping; _playerSpeedY = -_playerSpeedY * CannonCollisionDamping;

This is similar to what you did for making the spaceship bounce off the screen borders. Build and run to see how it works.

It works pretty well when the spaceship is going fast when it hits the cannon. But if it’s moving too slow, then even after reversing the speed, the ship stays within the collision radius and never makes its way out of it. So this solution has some problems.

Instead of bouncing the ship off the cannon by reversing its existing speed, you will simply expel the ship with a fixed speed so that it always moves outside of the collision area, no matter how fast or slow it was flying when it hit the cannon.

This requires you to add a new constant at the top of HelloWorldLayer.m:

const float CannonCollisionSpeed = 200.0f;

Remove those lines you just added in checkCollisionOfPlayerWithCannon and replace them with the following:

float angle = atan2f(deltaY, deltaX);   _playerSpeedX = cosf(angle) * CannonCollisionSpeed; _playerSpeedY = sinf(angle) * CannonCollisionSpeed; _playerAccelX = 0.0f; _playerAccelY = 0.0f;   _playerHP = MAX(0, _playerHP - 20); _cannonHP = MAX(0, _cannonHP - 5);

That is the speed you want the spaceship to have after it collides with the cannon. You need to calculate how much of that speed goes in the x-direction and how much in the y-direction, based on the angle of impact:

You can calculate the angle using arctan because you know the current speed of the player. To calculate how fast the player bounces off the cannon in the x- and y-directions, you already have two pieces of information: the angle that you just calculated, and the new speed, CannonCollisionSpeed, which is the hypotenuse of the triangle.

If you look back at the theory section, you will see that you can calculate the new x-component of the speed using the cosine function and the y-component using the sine function:

• new x-speed = cos(angle) * hypotenuse
• new y-speed = sin(angle) * hypotenuse

And that’s exactly what you’re doing in the code above. Build and run, and you’ll see the spaceship now bounces properly off the cannon and loses some hit points in the process.

Adding Some Spin

For an additional effect, you can add spin to the spaceship after a collision. This is additional rotation that doesn’t influence the flight direction. It just makes the effect of the collision more profound (and the pilot dizzy). Add a new instance variable in the implementation block:

@implementation HelloWorldLayer { ... float _playerSpin; }

In checkCollisionOfPlayerWithCannon, add the following line inside the collision detection block:

if (distance <= CannonCollisionRadius + PlayerCollisionRadius) { ... _playerSpin = 180.0f * 3.0f; }

This sets the amount of spin to a circle and a half, which I think looks pretty good. Now at the bottom of updatePlayer, add the following code to add spin to the rotation:

_playerSprite.rotation += _playerSpin; if (_playerSpin > 0.0f) { _playerSpin -= 2.0f * 360.0f * dt; if (_playerSpin < 0.0f) { _playerSpin = 0.0f; } }

The amount of spin quickly decreases over time – I chose a speed of 720 degrees per second. Once the spin has reached 0, it stops.

Build and run and set that ship spinning!

Where to Go from Here?

Here is the full example project from the tutorial up to this point.

Triangles are everywhere! You’ve seen how you can use this fact to breathe life into your sprites with the various trigonometric functions.

You have to admit, it wasn’t that hard to follow along, was it? Math doesn’t have to be boring if you can apply it to fun projects, such as making games.

But there’s more to come: in Part 2 of this Trigonometry for Game Programming series, you’ll add missiles to the game, learn more about sine and cosine, and see some other useful ways to put the power of trig to work in your games.

In the meantime, drop by the forums to share how it’s going for you so far! Or if you really can’t wait until part 2, why not brush up on some more Cocos2D with some of our other tutorials. Alternatively, if you want to start diving into more game development why not take a look at the games starter kits available on this site.

Credits: The graphics for this game are based on a free sprite set by Kenney Vleugels. The sound effects are based on samples from freesound.org.

This is a post by iOS Tutorial Team member Matthijs Hollemans, an experienced iOS developer and designer. You can find him on Google+ and Twitter.»

MINUTOS MUSICALES

Una de esas bandas de los 80 y 90 que hay que recordar:

11 SIMPÁTICAS VIÑETAS DE CÓMO SON LAS ENTREVISTAS DE TRABAJO EN LAS COMPAÑÍAS MÁS IMPORTANTES DEL MUNDO

http://culturainquieta.com/es/lifestyle/item/11004-11-simpaticas-vinetas-de-como-son-las-entrevistas-de-trabajo-en-las-companias-mas-importantes-del-mundo.html

Casi todas las grandes compañías tienen sus estereotipos. Ya sea escribir nombres en vasos (Starbucks), etiquetar todo (Instagram) o perder cosas (servicios postales), todas las compañías los tienen. El artista conceptual e ilustrador de Kazajstan, Leonid Khan, ha dibujado 12 divertidas ilustraciones para Bright Side mostrando cómo serían las entrevistas de trabajo en las compañías famosas basándose en sus estereotipos. ¿Cuál os parece más real?

 

1. IKEA

 

2. APPLE

 

3. CUALQUIER TRABAJO

 

4. STARBUCKS

 

5. SAMSUMG

 

6. INSTAGRAM

 

7. CORREOS

 

8. MCDONALD`S

 

9. OLD SPICE

 

10. VOLVO

 

11. GOOGLE

via brightside

 

 

Así es como los lobbies han arruinado tu alimentación

http://blogs.elconfidencial.com/mercados/perlas-de-kike/2016-11-22/obesiad-diabetes-lobbies-arruinado-alimentacion_1293210/

¿Crees que debes reducir grasas y hacer más ejercicio para adelgazar? Quizá te interese saber que eso es un mito de la industria para no señalar a los verdaderos culpables

Actualmente muere más gente por obesidad que por hambre. Un tercio de la población mundial sufre, como mínimo, sobrepeso (se considera sobrepeso contar con un IMC > 25). Ningún gobierno del mundo ha conseguido reducir esta lacra en las últimas 3 décadas y, según McKinsey, en el 2030 a la mitad de la población mundial le sobrarán kilos. La obesidad es, de hecho, uno de los principales costes de nuestros sistemas sanitarios y no para de aumentar. Da igual que hablemos de países desarrollados o emergentes, estamos ante una epidemia silenciosa y global. ¿Qué está pasando?

Puede que el primer culpable en nuestra mente sea el sedentarismo pero, paradójicamente, hacemos más deporte y vamos más al gimnasio que nunca. Puede que el segundo teórico culpable sea la ingesta excesiva de grasa, al fin y al cabo cuando miramos nuestros michelines es lo que vemos, y si está ahí será porque antes la hemos comido. O quizá culpemos al exceso de calorías en nuestra dieta diaria: si comemos más calorías de las que necesitamos la diferencia se acumula en grasa, o eso dicen las creencias populares.

Yo también lo creía. Creía que el sobrepeso y la obesidad eran por no cuidarse lo suficiente, y que, cuando alguien afirmaba seguir una dieta relativamente saludable y ganar peso de igual forma, simplemente no estaba diciendo toda la verdad. Como a mí no me pasaba, creía simplemente que otros no hacían lo suficiente. Pero me equivocaba, como así pude comprobar tras meses y meses leyendo estudios científicos sobre nutrición. Mi conclusión actual es que casi todo lo que creemos saber sobre cómo alimentarnos no es correcto y, además, sí existen unos culpables claros en la epidemia de la obesidad.

Este médico ha desvelado el código de la obesidad (y sabe cómo debes adelgazar)

MIGUEL AYUSO

Como cuenta Jason Fung en su nuevo y revelador libro, ‘The obesity code’, seguir una dieta saludable no es suficiente para perder peso. Esto es lo que debes hacer

Puede parecer extraño tratar un tema así en una sección de economía, pero el hecho es que me siento en la obligación de contar lo aprendido, aun siendo una ‘verdad incómoda’. Además, no podríamos estar ante un tema más económico pues, si las cosas son como son es, seguramente, por la fuerte presión que han ejercido los lobbies de la industria alimentaria en las últimas décadas. Si a eso le añadimos a gobernantes que han preferido velar por ciertos intereses privadosen lugar de por la salud pública, y científicos que venden su voz a cambio de considerables sumas de dinero, ya tenemos el guion de esta historia.

Lo que la evidencia científica independiente parece decir en los últimos años es que el gran problema son el azúcar y las harinas refinadas. Cuando tomamos una dosis de azúcar añadido (o de sucrosa, o fructosa, o glucosa, o dextroxa, o lactosa, o maltosa, o jarabe de maíz, o maltodextrina… o alguno de los cientos de nombres que existen para que no sepas lo que estás tomando cuando compras un producto procesado) o de harina blanca refinada (productos procesados, pan, arroz, pasta y cereales no integrales) lo que provocamos en realidad en el cuerpo es una reacción en forma de insulina, derivada del alto índice glucémico y alta carga glucémicade estos alimentos.

Cuando obtenemos energía por medio de las proteínas y las grasas no se produce este efecto, obteniendo una liberación más progresiva y que, por tanto, compensamos con nuestra actividad diaria. Lo mismo ocurre con los hidratos de carbono con bajo IG. Sin embargo, en el caso de los azúcares y las harinas refinadas, el proceso es tan rápido que el efecto es una transformación rápida en grasa abdominal (con el incremento de riesgo para nuestra salud que eso conlleva: enfermedades cardiovasculares, diabetes e incluso cáncer).

Casi uno de cada tres españoles será obeso en 2025, asegura The Lancet

ACYV

Se ha triplicado el número de hombres con problemas de sobrepeso y multiplicado el de mujeres en los últimos 40 años, y la cosa sólo marcha hacia peor

Por ello, aunque parezca contraintuitivo, tendremos menos grasa tomándola directamente que ingiriendo azúcar o harinas refinadas. De hecho, solo las grasas ‘trans’ son claramente negativas, no existiendo evidencias en las demás (o incluso considerándose positivas, recordemos por ejemplo el aceite de oliva).

Existen polémicas sobre innumerables cuestiones: sobre los productos integrales, sobre los lácteos, sobre la carne roja, sobre los edulcorantes, sobre tomar más o menos hidratos de carbono… Sin embargo, por lo que respecta a nuestros ‘amigos blancos’, cada estudio o libro publicado en los últimos meses no financiado por la industria es claro: el azúcar blanco y las harinas refinadas son un culpable claro de la epidemia de la obesidad. Si la evidencia parece tan rotunda, ¿por qué los gobiernos no toman cartas en el asunto? ¿Por qué no se modifican los patrones recomendados de las ‘dietas saludables’? ¿Por qué la población no sabe cómo cuidar su alimentación?

Voy a contar una breve historia que quizá ayude a entenderlo. A partir de los años 50, en Estados Unidos empezaron a publicarse estudios señalando al azúcar como un elemento nocivo para la salud, algo que inquietó a la industria: su reducción en la dieta diaria podría perjudicar sus beneficios. Por ello se ofrecieron a ‘colaborar’. Por ejemplo cuando el azúcar se señaló como culpable de la caries, la industria ‘colaboró’ con el Insituto Nacional para la Investigación Dental, quién aceptó el 78% de sus propuestas y abandonó toda intención de limitar el azúcar.

Cuando las evidencias se hicieron aun más fuertes, y los estudios comenzaron a relacionar la ingesta con la obesidad, los lobbies ‘promocionaron’ su propia teoría: el culpable de la obesidad no era tanto el azúcar como las grasas saturadas. Hasta hoy, y eso que no existe evidencia en contra de las grasas saturadas. Así, los gobiernos aceptaron la hipótesis y se estableció que lo correcto era reducir las calorías de los alimentos y reducir sus grasas, aun a costa de aumentar el azúcar añadido. Algo que perdura hoy en día, puesto que detrás de cada alimento ‘bajo en grasa’, existe en realidad uno ‘alto en azúcar’ (o cualquier otro sinónimo), nada recomendable para bajar de peso, por cierto.

El autor de dietas más exitoso te explica cómo vencer a la diabetes y adelgazar

MIGUEL AYUSO

Michael Mosley, el médico y periodista de la BBC que dio a conocer la dieta 5:2, vuelve ahora con la ‘Blood Sugar Diet’, que ya es el régimen más popular de Reino Unido

Cuando las evidencias se hicieron aun más sólidas, la industria comenzó a contar con personajes más renombrados, contratando a científicos de Harvard para velar por sus intereses. Tal era la influencia de sus lobbies que rápidamente se expandió la idea por todo el mundo: si quieres adelgazar come menos grasa y haz deporte. Y algo parecido se puede decir del trigo, promocionado por departamentos gubernamentales como el Departamento de Agricultura de Estados Unidos, quien de la noche a la mañana se convirtió en un oráculo de la nutrición, a pesar de que su función era la promoción de los productos. La pirámide alimenticia no fue un acto de educación sobre la población, fue un acto de marketing para favorecer la industria autóctona.

Si crees que estamos ante hechos aislados, recomendaría una mayor lectura de la prensa diaria. El pasado mes se descubrió que tanto Coca-Cola como Pepsi pagaron a 96 organizaciones sanitarias estadounidenses para silenciar sus críticas sobre el azúcar. Cada vez que alguien abre la boca criticando, las multinacionales hacen presión para hacerlo cambiar de parecer, dándose situaciones tan rocambolescas como ver a la Asociación Americana contra la Diabetes luchando por eliminar un posible impuesto sobre el azúcar. Más criticable aún que las prácticas de la industria, copiadas de las empleadas tabaqueras, es la facilidad con la que se venden los gobiernos y organismos públicos.

Si alguien, a pesar de todas las evidencias existentes, cree que se trata de una conspiración, quizá debería de escuchar a Margaret Chan, Directora General de la Organización Mundial de la Salud. El organismo, que durante años ha suavizado sus posturas al respecto (y recibido millones de euros), afirma ahora (traducción de Juan Revenga) lo siguiente:

[…] En la actualidad las campañas de promoción de estilos de vida saludables y la adopción de conductas para alcanzarlos se encuentran con la oposición de fuerzas que distan mucho de ser “amables”. Más bien, todo lo contrario.

El esfuerzo público dirigido a prevenir las enfermedades no transmisibles [tras hacer una especial alusión a la obesidad, la diabetes y el cáncer] se enfrenta a intereses comerciales de poderosos agentes económicos. En mi opinión, este es uno de los mayores retos a los que se enfrentan las campañas de promoción de la salud.

Tal y como pone de manifiesto la publicación que resume esta conferencia [ver al completo en este enlace] no solo se trata del problema observado en otro tiempo con las grandes tabacaleras (Big Tobacco). Ahora, la Salud Pública tiene que lidiar también con otras industrias en los mismos términos que entonces; se trata de la “Big Food”, “Big Soda” y “Big Alcohol”. Son estas industrias las que en este momento temen una regulación de sus productos por parte de las administraciones sanitarias y las que están recurriendo a las mismas tácticas que antaño puso en práctica la industria tabacalera.

El pasado reciente aporta pruebas suficientes para documentar que estas tácticas por parte de la industria alimentaria ya se han puesto en marcha. Entre ellas, se incluyen la creación de empresas dentro del mismo grupo con una “cara amable”, la creación de grupos de presión [lobby], el realizar promesas de autorregulación, el interponer demandas y el financiar estudios de investigación que lo que consiguen es tergiversar la evidencia y confundir al ciudadano.

Además, este tipo de tácticas también incluye la realización de donaciones, regalos y contribuciones relacionados con causas nobles, o bien vistas por parte de la comunidad, de forma que estas industrias terminan siendo percibidas como corporaciones respetables tanto a los ojos de la ciudadanía como ante los de la clase política. Entre sus estrategias destacan también el hacer descansar la responsabilidad de una mala salud en cada persona, individualmente; así como pretender hacer creer que las acciones de los gobiernos por regular estas cuestiones no son otra cosa sino una forma más de interferir en la libertad personal de cada cual y su derecho a elegir libremente.

La oposición ejercida es de una magnitud formidable. Ya que un amplio poder en los mercados se traduce en poco tiempo en poder político, son pocos los gobiernos que han priorizado las cuestiones de salud frente a los grandes negocios. Tal y como hemos aprendido de experiencias anteriores, como con la del tabaco, cuando una corporación poderosa se lo propone, puede vender casi cualquier cosa a la población.

Déjenme recordarles una cosa. Ni un solo país del mundo ha conseguido darle la vuelta a la epidemia de obesidad en todos los grupos de edad. Esta realidad no es consecuencia de una falta de voluntad individual. Es consecuencia de la ausencia de voluntad política a la hora de meter mano en este gran negocio.

Estoy profundamente preocupada por dos actuales tendencias:

La primera implica la posibilidad de que la industria y las administraciones lleguen a acuerdos “comerciales” fruto de las denuncias de la primera sobre las segundas. En la actualidad, algunos de los gobiernos que han establecido medidas para proteger la salud de sus ciudadanos están siendo llevados a los tribunales por parte de la industria. Y esto es peligroso.

La segunda se refiere al interés que pone la industria para moldear las políticas de Salud Pública, en especial aquellas medidas que afectan a sus productos. Si una industria está involucrada en la formulación de políticas de Salud Pública, tengan la seguridad de que aquellas medidas más eficaces serán o bien minimizadas o bien apartadas en su totalidad. Esta tendencia también está bien documentada y es así mismo peligrosa.

Gracias.

Yo no soy nutricionista, ni médico. No sé de alimentación más que lo que he leído en meses tratando de aprender al respecto. Pueden creerme, o no. Pueden tomar en cuenta los consejos y reducir azúcar y harinas refinadas (las grasas trans también estarían bien), o no. Pero sí sé como actúan muchas empresas, y la conducta de Coca-Cola o Pepsi, o de otros que venden cereales nada saludables, encaja perfectamente con la de empresas que ven como su negocio podría menguar si la población descubre la verdad.

El azúcar o la harina blanca son adictivos, saben bien y son baratos, la industria no está preparada para prescindir de ellos (sus esperanzas a futuro están puestas en los controvertidos edulcorantes). Sin embargo lo más probable es que sean uno de los elementos más perjudiciales que tenemos en nuestra alimentación actual. Lo más probable es que esa tripa que tienes sea su culpa, no de la falta de ejercicio, no del exceso de grasas. Por supuesto que el deporte es sano, y no hay que caer en dietas extremistas, pero va siendo hora de poner las cosas en su sitio y señalar a los verdaderos culpables. ¿Los ciudadanos? No, las mentiras interesadas que nos han contado.

Ayn Rand

La magnitud de la corrupción en España

Para que no se nos olvide.

Y si la Filosofía desaparece del Bachillerato, ¿qué?

La semana pasada alguien colgó en la la sala de profesores de mi centro una carta (la enésima) sobre lo importante que es la Filosofía, que nos salva a todos de no ser unos borregos, y su desaparición (falso) del bachillerato.

No sé quien ha sido pero me imagino que será el mismo que hace unos años colgó un artículo sobre esquiroles antes de una huelga general para insultarnos en la cara a la mitad del claustro.

Huelga decir que es una gran falta de respeto lo que hace este compañero ya que en un centro educativo todos los profesores tenemos nuestra propia ideología y, en contra de lo muchos piensan, muchos no somos ni rojos ni progres, ni, por supuesto, creemos que la Filosofía es la salvadora de nada ni de nadie y que hay muchas otras asignaturas en las que los alumnos aprenden a «pensar» mucho más.

Como dice el artículo que pongo a continuación, estos profesores solo pretenden salvar su acomodado puesto de trabajo, los más privilegiados de cualquier centro de secundaria. Un «quéhaydelomío» de libro.

 

«Y si la Filosofía desaparece del Bachillerato, ¿qué?

Lo mejor será que empiece negando la posibilidad de que se cumpla lo que plantea el título. Es sencillamente imposible que la Filosofía desaparezca del Bachillerato. Y es imposible de la misma manera que es imposible que la UE expulse de su seno a una hipotética Cataluña independiente.

Seguramente algunos ya habrán visto el truco en esta extraña equiparación, sólo con reparar en el título. La imposibilidad en los dos casos no deriva de una posición de fuerza ni de una situación inmutable, sino justamente de la inexistencia de eso de lo cual se plantea la desaparición. La Filosofía no desaparecerá del Bachillerato porque hace tiempo que no está, y Cataluña no sería expulsada de la UE porque no pertenece a ella.

Lo de Cataluña no es discutible, por mucho que se empeñen Romeva, Mas y el resto de osados galos. Si finalmente se consuma la ilegalidad que han ido explicitando este último año largo, es de esperar que el cielo caiga sobre sus cabezas. Y sobre la del resto de los alegres compatriotas que consciente o inconscientemente les han seguido -o empujado- hasta el abismo.

Ahora bien, ¿en qué sentido decimos que la Filosofía no existe en el Bachillerato? Al fin y al cabo, ha sido hasta ahora una asignatura obligatoria en los dos cursos de los que se compone. En todo caso, habría que preocuparse por la eliminación de la obligatoriedad en el segundo año, como han apuntado tantos escritores, profesoreso incluso alumnos en los últimos meses. Es sorprendente este súbito amor no ya por el conocimiento, sino por la Filosofía, que se ha despertado desde que Wert introdujo esta cuestión en su reforma de la Ley de Educación.

Pero no es este despertar lo que voy a tratar ahora. Al fin y al cabo, es posible que ese amor siempre haya estado ahí, escondido. Lo que voy a tratar, porque me parece preocupante, es la idealización desde la que se contempla la Filosofía. O, mejor dicho, la asignatura de Filosofía. Esa idealización se observa perfectamente en uno de los argumentos que más se han esgrimido para criticar la pérdida de la obligatoriedad en Bachillerato, y es el siguiente: “Si se elimina Historia de la Filosofía del Bachillerato, estaremos condenando a los alumnos a una existencia borreguil, puesto que perderán la capacidad de desarrollar un pensamiento crítico.”

Serán “esclavos del sistema”, dicho de otro modo.

Esto, por no extendernos demasiado, es una estupidez. Y si lo dice un profesor de Filosofía, además, una idiotez.

Dos pruebas.

La primera: hasta ahora hemos tenido varias promociones de alumnos con dos cursos de Filosofía obligatorios. La última de ellas la “generación más preparada de la Historia”, expresión a la que es ya imposible acercarse sin malicia. No creo que nadie se atreva a afirmar que todos ellos han desarrollado un mínimo de pensamiento crítico. Quiero decir, es de suponer que el mismo Romeva cursó dos años de Filosofía. Más aún, todos aquellos que aplauden las mentiras y piden otra ración, todos aquellos que hablan de sentimientos colectivos y convierten en afrenta cualquier opinión disidente, todos ellos, han cursado dos años de Filosofía. Pero podemos ir aún más cerca. Concretamente, a las asambleas del 15M. Muchos de los autores de las propuestas psicodélicas que pudieron verse durante esos días no sólo cursaron dos años de Filosofía en Bachillerato, sino que incluso prosiguieron sus estudios sobre la materia en la universidad. Algunos, incluso, eran profesores de Filosofía. Por lo tanto, parece que la mera presencia de la Filosofía en el Bachillerato no es un antídoto eficaz contra el borreguismo.

La segunda, más que una prueba, es una cuestión de léxico. Y una apelación al principio de realidad. Es decir, una reivindicación de la propia esencia de la Filosofía, que no es otra que el abandono del pensamiento mágico. Valga el oxímoron. La Filosofía en ese sentido no es más que hablar con propiedad, llamar a las cosas por su nombre. Empezando, claro, por la propia Filosofía.

La Filosofía, en Bachillerato, es una asignatura. Y hay que situarla en un contexto determinado. Los alumnos, los profesores y el sistema de enseñanza son la parte fundamental de ese contexto. Y todas las asignaturas son afectadas -determinadas- por ese contexto concreto. Así, la asignatura de Filosofía de hoy en día poco tiene que ver con la que podía haber hace treinta o cuarenta años.

Comenzando por la Historia de la Filosofía, que es la que en principio está en riesgo de desaparecer, debemos aceptar que ni es Historia, ni es Filosofía. Son apenas cinco o seis cuestiones sobre cinco autores, sin conexión entre ellas. Seguramente hay profesores -y alumnos- que aprovechan al máximo la asignatura, pero el caso es que es perfectamente posible acabar el curso sin haber entendido nada. Diría incluso que es lo más frecuente. Al fin y al cabo, la economía del centro y la de los estudiantes coinciden en ese sentido: maximizar el resultado, minimizar el coste. Lo que se traduce en el aprendizaje de cinco autores. En realidad son diez, como todos sabemos, pero en el examen siempre se ofrece la posibilidad de elegir uno de un par de autores que comparten la misma época. Platón o Aristóteles, San Agustín o Santo Tomás, Descartes o Kant, Marx o Nietzsche, Ortega o Wittgenstein. La clave está en la disyuntiva.

Por simplificar, un alumno de 2º de Bachillerato puede estudiar a Aristóteles sin haber visto más que un par de pinceladas de Platón. Y de hecho, puede sacar buena nota en la prueba de Selectividad. Porque como ya hemos dicho, de lo que se trata es de conseguir una buena nota, y para eso basta con memorizar algunas respuestas tipo.

Es cierto que hay una parte del examen para la que no basta ese aprendizaje memorístico. Es el comentario crítico. O como quiera que se llame ahora. Disertación, creo. Casi un tercio de la nota, trescientas palabras como máximo. Aquí es donde, dicen, tienen que lucirse. El problema es que no tienen la base necesaria para hacerlo. Es un obsceno ejercicio de voluntarismo. No hay tiempo para trabajarlo en clase, y depende de dos cuestiones que son ajenas a la asignatura: el conocimiento material y el conocimiento formal. Es decir, referencias externas y madurez expresiva. Sin eso, poco se puede hacer.

Pretender que los alumnos sean capaces de hacer una disertación filosófica sin esos conocimientos es como pretender hacer una tortilla sin huevos. Se le puede llamar Filosofía a lo primero y tortilla a lo segundo, pero no lo son.

La Filosofía de Bachillerato, por tanto, no es lo que dirige a los alumnos hacia la madurez, al contrario de lo que apuntaba Montano hace poco.

Queda por tanto la Filosofía de 1º. Ahí podríamos situar la aportación específica de la Filosofía en el curriculum educativo. No hay examen final y hay más flexibilidad para adaptar la asignatura. No sé hasta qué punto es bueno, pero ésa es otra cuestión. De cualquier manera, no es suficiente por sí mismo. Todo profesor sabe que es muy difícil generar pensamiento crítico. A no ser que digamos que el pensamiento crítico es lo mismo que la opinión. Entonces sí. Hay opiniones a patadas. Sobre cualquier tema. Todos los alumnos tienen una, o incluso varias, sobre la misma cuestión. Y ahí radica el engaño. La Filosofía ha servido para perpetuar el imperio de la doxa contra el que ya advirtieron Platón y Aristóteles. En la ESO y en el Bachillerato se ha instalado la creencia de que los alumnos tienen que opinar sobre todo, y enseguida además. Seguramente esa creencia tiene la misma fuente pedagógica que el diabólico “Que lean lo que sea, pero que lean”. Y así, en lugar de aprovechar esos años para borrar todas las opiniones, para quedarnos sólo con los análisis y los hechos, para alertar contra los sesgos y la tiranía de los afectos y para, en definitiva, colocar a la razón en el lugar que le corresponde, se ha ido construyendo una ampliación de la educación infantil, en la que lo importante es lo superficial. Ése es el contexto, del cual nada escapa. Ni los profesores, ni los alumnos, ni las asignaturas.

Así que, en contra de lo que han expuesto muchos otros compañeros de profesión, no creo que la Filosofía corra el peligro de desaparecer del Bachillerato, porque a esto que tenemos ahora sólo se le puede llamar Filosofía por inercia. Y difícilmente puede ser ese generador de madurez y pensamiento crítico que muchos, con buena intención, le suponen. Precisamente el insistir en esa idealización no es sino una prueba más de lo que estamos diciendo. Cualquier alumno de Bachillerato debería ser capaz de hacer un análisis similar. Y no digamos un profesor de Filosofía.

Lo que sí está en peligro de desaparecer, justamente, es esto mismo. La profesión de profesor de Filosofía en Bachillerato. Y es desde luego un asunto importante, fundamentalmente para quienes nos dedicamos a ello. Extender esa visión mágica sobre nuestra labor probablemente nos granjeará muchas simpatías, y puede que incluso sirva para convencer al resto de la sociedad de que es imperativo que siga ocupando el espacio que hasta ahora ha ocupado en la enseñanza. Bien es verdad que no lo tendremos demasiado difícil, puesto que ya nos hemos ocupado de prepararles para que consuman con gusto ese pensamiento débil.

Imagino que los tiros seguirán yendo por ahí. Lo sentimental, el énfasis y la indignación. Justo lo contrario de lo que se supone que es la Filosofía.

ACTUALIZACIÓN:

En este blog han escrito una réplica a lo que publiqué aquí hace unos días. Si a alguien le interesa, puede leer tanto la réplica como mi respuesta a esa réplica. El tono es desagradable porque el autor de la réplica lo lleva a lo personal, así que advertidos quedan los lectores.»

«Cuidado con lo que deseas en Internet»: la siniestra historia del señor Montgomery

A woman´s eye. Wikimedia Commons

http://es.gizmodo.com/cuidado-con-lo-que-deseas-en-internet-la-siniestra-h-1788991604

Cada año surgen miles de nuevas parejas en Internet, unas estadísticas que se multiplican con el paso del tiempo. Muchas de estas relaciones han acabado perdurando, otras han servido como simple encuentro sexual. Luego están aquellas que han acabado mal. Por último, está la historia de Tommy y Jessi.

Nueve estudiantes de una universidad le piden sexo al resto del campus, ¿qué podía salir mal?

Un joven universitario camina por el campus pensando en sus cosas. Aunque él jamás se ha…Seguir leyendo

Se calcula que el 75% de los estadounidenses solteros ha utilizado la red al menos una vez para ligar. En otros países como España las estadísticas del INE indican que al menos la mitad de los solteros y solteras de menos de 20 años suele utilizar la red una vez al mes con la esperanza de encontrar una relación.

A estas cifras hay que sumarle otras más perturbadoras. Por ejemplo, y siguiendo la senda de los chats de citas y similares, se calcula que el 81% de los miembros de estos portales miente en sus perfiles, ya sea con la edad, su peso, altura, foto, o en el peor de los casos, con todo.

Resulta tan sencillo como escalofriante hacerse pasar por otra persona en Internet. Una industria, la de las citas online, a la que no parece afectarle este tipo de “trastornos” inherente en muchas personas. En el año 2007 generaba 700 millones de dólares y que para el 2012 ya rozaba los dos mil millones de dólares.

Se calcula que el 81% de los miembros de estos portales miente en sus perfiles, ya sea con la edad, su peso, altura, foto, o en el peor de los casos, con todo

Si tuviésemos que establecer un tiempo para definir el momento previo al boom de este tipo de apps, sin duda sería antes del 2007. Por aquellas fechas estábamos en los inicios de la gigantesca industria que hoy habita en la red, esa que frecuentemente nos recuerda lo importante que es mantener una contraseña segura.

Antes del 2007 cualquier chat valía para comenzar un ligero flirteo bajo el manto y la seguridad del anonimato que ofrecía un nick elegido por el usuario. Pogo fue una de esas webs. Aunque nunca ha dejado de ser una página de juegos online, muchos usuarios la utilizaban por aquel entonces para lanzar sus mensajes algo más atrevidos a otros usuarios, lo que en ocasiones acababa en encuentros reales fuera del espacio digital.

Así fue como se conocieron la primavera del 2005 los usuarios Talhotblondy MarineSniper.

Esta fue su escalofriante historia.

Cuando Tommy encontró a Jessi

Thomas Montgomery. ABC News

Cada día y durante los últimos 12 años Thomas Montgomery se había levantado a la misma hora de la mañana para acudir a la fábrica de perforación en la que trabajaba en Clarence, una pequeña ciudad en el norte de Nueva York. Un hombre aplicado, a menudo de los últimos en salir del trabajo, que cuando terminaba su jornada acudía rápido a casa para pasear al perro y llevar a sus dos hijas a natación.

A Montgomery se le conocía en la ciudad como un tipo agradable, un buen hombre, buen padre y un marido decente para su esposa Cindy, con la que llevaba 16 años casado. De entre las aficiones que tenía en su tiempo libre destacaba una: su pasión por las partidas cada viernes al Texas Hold ‘Em en la web Pogo.com.

A Montgomery se le conocía en la ciudad como un tipo agradable, un buen hombre, buen padre y un marido decente para su esposa Cindy, con la que llevaba 16 años casado

Thomas tenía por aquellas fechas 45 años, un ex marine con bigote rojizo, algo obeso y poco pelo. Quizá por ello un día se miró a sí mismo y no le gustó lo que veía. Quizá por ello también un día decidió que no quería ser esa persona. Sería un marine como en sus tiempos de joven, un marine de 18 años llamado Tommy. Además también sería cinturón negro de kárate, un tipo atlético con una cicatriz de bala en el hombro izquierdo que le daría al personaje un aura de héroe.

Estos pensamientos los convirtió en realidad (digital). Thomas, envalentonado, entró en Pogo y se registró como ese joven vigoroso. Y fue al poco tiempo de crearse esa nueva identidad cuando Thomas Montgomery conoció a Talhotblond, una joven de 17 años natural de Virginia cuyo verdadero nombre era Jessica, cómo luego le revelaría la joven.

Ashley Madison. Getty

Jessica en muy poco tiempo pasó a ser “Jessi” en el chat privado que comenzaron a seguir ambos. Conforme pasaba el tiempo Thomas iba creando un perfil de película. Un joven cuya madre había muerto cuando él era pequeño, un chico problemático que se alistó en el ejército como única salida y que estaba listo para ir a combatir a Irak.

Los cuentos de Tommy acerca de su difícil vida atrajeron a la joven. Tommy era un alma herida y Jessi le proporcionó consuelo, a menudo le decía lo orgullosa que estaba de él a pesar de los errores. La intimidad entre ambos iba creciendo hasta tal punto, que un día Tommy le aseguró a Jessi que pensaba suicidarse en Irak, a lo que ella respondió que le debía prometer que volvería sano y salvo por ella.

Un día decidió que no quería ser esa persona. Sería un marine como en sus tiempos de joven, un marine de 18 años llamado Tommy. Además también sería cinturón negro de kárate

Como recordaría años después el señor Thomas “pensaba que jamás nos íbamos a encontrar, así que simplemente estaba jugando con ella”. Pero en muy poco tiempo comenzaron con el flirteo que acabaría en romance. Jessi comenzó a enviarle fotos sugerentes y provocativas a Tommy. Cuando el joven estaba ausente por los entrenamientos en el ejército Jessi hablaba con Tom Jr., el padre de Tommy, en esencia otro personaje que había creado Montgomery para darle “mayor autenticidad”.

Tras las primeras fotos sugerentes de Jessi esta le pidió a Tommy algunas de él. Montgomery entonces le envió fotos antiguas de cuando estaba en el ejército, imágenes que encontraba y que hacían honor a su nick MarineSniper, un nombre creado como recuerdo nostálgico de los seis años que pasó en el ejército cuando era joven. Un espacio en el tiempo en el que se graduó como experto francotirador.

Pogo.com actualmente

La relación online fue subiendo de tono en muy poco tiempo, los mensajes de chat en su ordenador recuperados posteriormente mostraban una relación que comenzaba a devorar al hombre de familia, un flirteo que consumía el interior de Montgomery. Como él mismo le dijo al tribunal: “se hizo más real para mí que la vida real”.

El sentimiento parecía ser mutuo. Jessi y Tommy comenzaron a intercambiar regalos, luego comenzaron las primeras llamadas telefónicas, las primeras cartas de amor… “Te quiero por siempre jamás, Tommy”, le escribía Jessi. “Nunca me he sentido así”, respondía Montgomery.

Cuando el joven estaba ausente por los entrenamientos en el ejército Jessi hablaba con Tom Jr., el padre de Tommy, en esencia otro personaje que había creado Montgomery para darle “mayor autenticidad”

Aquello ya no era un flirteo. “Había sexo virtual entre ella y Tommy”, comentó al tribunal Montgomery. En este punto, mientras que el hombre aseguraba sentirse “sucio” por el sexo virtual, también decía que estaba profundamente enamorado como para poder cortar los lazos con ella.

Por aquellas fechas, el hombre de familia al que sus allegados tenían por una persona decente estaba a punto de perder el contacto con la realidad. De hecho, al poco tiempo cruzó el umbral escribiéndose una nota a sí mismo donde se decía:

El 2 de enero de 2006 Tom Montgomery (de 46 años de edad) deja de existir y es reemplazado por un marine de 18 años de edad, con cicatrices de batalla … Se está trasladando a Virginia para estar con el amor de su vida.

Jessi se había enamorado de Tommy, y Montgomery de Jessi, o al menos, bajo la idea de sí mismo como Tommy. Pero los fotos de Jessi provocaron la primera explosión importante en la pareja. Montgomery se convenció de que esas fotos también se las había enviado a otros admiradores en la red y la acusó de traicionarlo. Para disculparse, Jessi le envió una carta y una cadena de plata con las iniciales T&J. Tommy la perdonó pero Montgomery, en el papel del padre Tom Jr., no lo hizo en un primer momento.

Como podemos apreciar, Montgomery estaba muy cerca de cruzar esa fina línea que separa a la locura de la razón.

Unos meses más tarde la relación explotaría por todos lados.

Cuando Cindy encuentra a Jessi

La familia de Montgomery. Talhotblond

En la navidad del 2005, unos ocho meses después de conocerse online, Tommy le propone matrimonio y Jessi acepta. Montgomery estaba consumido por esa relación virtual, mientras trabajaba no dejaba de hablar de ella y le decía a sus compañeros que planeaba dejar a su esposa y trasladarse a vivir a Virginia.

Mientras, Cindy, la mujer de Montgomery, seguía sin saber nada de la doble (casi triple si contamos a Tom Jr.) vida de su marido. Sabía que algo había cambiado en el hombre, quién desde que llegaba a casa del trabajo no salía de Internet en los últimos meses.

Pero en marzo del 2006 todo cambió. Una de las hijas de Montgomery estaba utilizando el ordenador de su padre mientras este trabajaba, cuando de repente salta un mensaje instantáneo de Jessi que la joven pudo leer. La hija acudió rápidamente a su madre y está encontró de golpe el chat abierto con las conversaciones íntimas entre su marido y Jessi. Cindy registró el cuarto y las pertenencias de su marido y encontró cartas de amor entre ambos y unas bragas rojas que le había enviado Jessi.

En la navidad del 2005, unos ocho meses después de conocerse online, Tommy le propone matrimonio y Jessi acepta. Montgomery estaba consumido por esa relación virtual

¿Qué hizo Cindy? Le envió a Jessi una foto de su familia junto al siguiente mensaje:

Permítame presentarles a estas personas. El hombre del centro es Tom, MI MARIDO desde 1989. Por cierto, Tom tiene 46 años. De lo que estoy sacando en claro de tus cartas es que estás más cerca de la edad de mi hija que de la mía. ¿Tienes más de 18 años?

Cindy había descubierto algo todavía más perturbador. La ropa interior había sido enviada al “padre” de Tommy durante unas fechas en las que se suponía que Tommy estaba en el ejército (realmente fueron fechas en navidad donde Montgomery no tuvo mucho tiempo para chatear con la joven). Cindy le dejó a su marido una nota al lado de su ordenador para que la viera cuando llegara del trabajo. Una nota que decía:

No puedo creer que estés viviendo esta extraña fantasía, pero aún peor, como padre e hijo. Si quieres separarte podemos hacerlo… pero seguir mintiéndome a mí y a tus hijas mientras ella envía “regalos” para tu supuesto hijo es totalmente inaceptable.

La pareja siguió viviendo los meses siguiente en la misma casa, aunque Montgomery pasó a vivir en el sótano. Mientras, Jessi no sabía a quién creer. ¿Tommy no existía o era Cindy la que se había inventado esa historia para separarlos?

Montgomery (izquierda y centro) y Barret (derecha). Tapology

Así fue como la joven encontró a un amigo que Montgomery había mencionado en alguna ocasión y que también frecuentaba Pogo bajo el nick Beefcake1572. Se trataba de Brian Barret, un estudiante de 22 años que trabajaba a media jornada en la empresa de Montgomery y solía jugar al póker con él. Barret le confirmó a Jessi sus peores temores y la joven quedó devastada.

El joven Barret comenzó a intimar con Jessi, primero consolándola, más tarde como algo más. Sus conversaciones se volvieron más íntimas y comenzaron a hacer público en los chats online quién era realmente Tommy. Montgomery quedó como un mentiroso, un depredador sexual de 46 años mientras ambos se burlaban de él. En cuestión de días su vida estaba destruida y el hombre llegó a plantearse el suicidio.

Permítame presentarles a estas personas. El hombre del centro es Tom, MI MARIDO desde 1989. Por cierto, Tom tiene 46 años

Montgomery comenzó una escalada de violencia verbal, le llegó a decir a Jessi que “Brian pagaría en sangre” lo que estaba haciendo. El hombre estaba devastado y había tocado fondo… cuando de repente le llega un nuevo mensaje de Jessi. La chica estaba desgarrada, loca y desesperada por volver a ese amor con un hombre que sabía que no existía. Parecía como si la nueva situación le provocara. De alguna forma, Montgomery era todo lo que quedaba de Tommy. Así comenzaron a chatear de nuevo:

Jessi: Me gustaba estar con Tommy, ¿lo echas de menos?

Tommy: Más de lo que jamás podrás imaginarte. Mi corazón me duele de escuchar que me llamas “tu Tommy”. Ojalá pudiera ser ese joven marine de 18 años para ti.

Jessi: Lo sé Tom.

Así fue como ambos volvieron a estar en contacto. Como decía Barbara Schoeder en el documental Talhotblond que contaba esta turbia relación:

En su mente, este fue el mayor premio. Él había sido aceptado con sus 47 años y esa joven lo seguía queriendo. Era como una droga, era adicto a ella y no podía quitársela de la cabeza.

Meses después Montgomery contaría que jamás hubo nada sexual entre ellos después de que Jessi descubriera su edad, pero el registro de los mensajes posteriores contaban una historia diferente:

Marinesniper: Deseo que estuvieras desnuda.

Talhotblond: ¿qué harías?

Marinesniper: mirarte fijamente.

Talhotblond: ¿eso es todo?

Marinesniper: no. Podría hacer magia.

Talhotblond: mmmmmm… hazme el amor Tommy.

Pero a pesar de estas promesas, Jessi continuó su romance con Barret. Sus conversaciones con él reflejaban los típicos problemas de adolescentes: se quejaban de sus padres, hablaban de los preparativos del baile, hablaban de sus primeros trabajos…

La joven parecía dividida entre los dos hombres. Cuando Barret la quería visitar durante sus vacaciones, ella le decía que no estaría. A Montgomery le decía que quería romper con Barret. El hombre la creyó hasta tal punto, que por primera vez en 15 años se dedicó a un duro entrenamiento corriendo 7 kilómetros diarios y pasando horas en el gimnasio… hasta que al final del verano del 2006 descubrió que Jessi y Barret seguían manteniendo la relación.

Cuando Tommy encontró a Barret

Montgomery, Jessi y Barret. DailyMail

Montgomery insultó gravemente a la joven y está rompió todos los lazos con el hombre. Habían terminado para siempre y para colmo Barret hizo público que iba a conocer a Jessi en persona.

Así, el 13 de septiembre del 2006, Tommy escribe a Jessi a la 1:33 en el chat que aún guardaba. Un mensaje donde la insulta y la amenaza. Ella le responde que la deje en paz. Montgomery responde pero ella no vuelve a escribirle. Al día siguiente Montgomery vuelve a intentar entrar en contacto con la joven insultándola. Ella seguía sin responder.

El hombre la creyó hasta tal punto, que por primera vez en 15 años se dedicó a un duro entrenamiento corriendo 7 kilómetros diarios y pasando horas en el gimnasio

A la mañana siguiente, el 15 de septiembre, Montgomery llama a la joven gritándole, lleno de rabia como recordaría Jessi. La joven colgó el teléfono. Más tarde, sobre las 22:00, Barret salía tarde de su jornada en la fábrica y se dirige a su coche. El joven abre la puerta y se acomoda en el asiento. En ese instante tres disparos perforan la ventanilla lateral del conductor y Barret cae fulminado. Tres disparos mortales que le alcanzaron en el cuello y en la parte superior del brazo.

Unas horas después Montgomery estaba delante de su ordenador tratando de chatear con Barret. Se trataba de una serie de mensajes donde le insistía a hablar con él. En entrevistas posteriores los detectives ya tenían a Montgomery como un posible culpable del asesinato. Poco después un compañero de trabajo les daba una pista crucial. Dos semanas antes Montgomery le había preguntado por la hora que solía terminar de trabajar Barret.

Montgomery. DailyMail

Cuando los detectives examinaron las llamadas de Barret encontraron el número de Jessi. Después de hablar con ella y en cuestión de minutos ataron cabos y tuvieron la certeza de la existencia de ese extraño triángulo amoroso que había ocurrido en Internet. Montgomery seguía desaparecido y la chica corría un grave peligro. El teniente Ron Kenyon que llevaba el caso llama una vez más a la joven y le dice que no abra la puerta a nadie bajo ningún concepto, que van a enviar a una patrulla de Virginia a su dirección lo más rápido posible. Como diría en el juicio:

Eran más o menos las tres de la mañana y nuestra primera y máxima preocupación era llegar hasta Jessi y asegurarnos de que seguía con vida.

Pero cuando la policía llegó a la dirección ofrecida por la joven se encontraron con una sorpresa. Allí sólo estaba Mary Shieler, una mujer obesa de 45 años y pelo corto en una casa blanca destartalada. La mujer le contó al oficial que era la madre de Jessi y que la adolescente no estaba en casa y no tenía manera de encontrarla.

El joven abre la puerta y se acomoda en el asiento. En ese instante tres disparos perforan la ventanilla lateral del conductor y Barret cae fulminado. Tres disparos mortales que le alcanzaron en el cuello y en la parte superior del brazo

El oficial llamó a Kenyon y este no podía creérselo, le dijo al oficial que insistiera, que había hablado con la joven hacía escasas horas y debía estar allí. Cuanto más presionó el oficial, más nerviosa se mostró Mary, quién finalmente dijo la verdad. Mary era la mujer con la que había hablado Kenyon por teléfono. Mart era la mujer por la que había muerto Barret. Y sí, Mary también fue la mujer por la que Montgomery había matado.

Mary era Jessi y Talhotblond y había usado la identidad de su hija para seducir a dos hombres hasta el fatal desenlace. La mujer se había dedicado a enviar las fotos de su hija, quién nunca tuvo conocimiento de la vida cibernética de su madre.

Poco después Montgomery fue detenido, acusado y finalmente declarado culpable del asesinato de Brian Barret. Le cayeron 20 años.

Por su parte, los fiscales buscaron la forma de acusar a Mary Shieler de algo, lo que fuera, cualquier cosa. Pero finalmente concluyeron que la mujer había caminado por una fina línea moral y ética, aunque sin cruzar la frontera de lo legal.

Con todo, Mary Shieler no salió indemne. Su esposo se divorció de ella tras enterarse de toda la trama.

Su hija jamás volvió a dirigirle la palabra.

LO QUE SE HA CAÍDO

http://www.albertonoguera.com/2016/11/lo-que-se-ha-cado.html

18 de noviembre de 2016
LO QUE SE HA CAÍDO
Cuando en una columna anterior he dicho que con las elecciones de EEUU se ha caído un «entramado progre» no sé si me he llegado a explicar bien. Lo que llamo entramado progre no es un mundo propiedad de los progres, sino la plutocracia (o el «establecimiento» como dicen en el programa de Bayly) que ellos han sustentado.

Después de la II Guerra Mundial fueron poco a poco creciendo en Francia ciertos movimientos ideológicos contrarios a las ideologías identitarias. Estos movimientos se basaban en tres principios: feminismo, multiculturalismo y pacifismo. Pronto todo esto acabó confluyendo en el fracasado Mayo del 68 y a partir de ahí pasó a las cátedras universitarias, desde las que se fue enviando gente hacia la educación y los medios de comunicación. El capitalismo no generó estos movimientos, pero pronto entendió que le convenía aprovecharlos en su beneficio. Tanto los judíos que se habían refugiado en los EEUU como la antigua burguesía que aún quedaba en Europa entendieron muy rápidamente lo siguiente:

Feminismo = mano de obra barata.

Multiculturalismo = mano de obra más barata.

Pacifismo = procurar que no nos hostien por los dos puntos anteriores.

Entonces, antes ya de que cayese el famoso muro de Berlín se había entendido que el ideario progre cumplía dos funciones: bloqueaba a la izquierda marxista (porque ocupaba su lugar) y generaba una repulsión hacia los movimientos vitalistas identitarios (a los que temían aún más). Todo el capitalismo siguió por ese camino y vio que, cuanta más moralina progre inyectaba en la sociedad, más se llenaban sus bolsillos y más se vaciaban los de los obreros. Era una cuadratura del círculo muy bien diseñada. Los problemas de insostenibilidad, de límite de la deuda o de invierno demográfico por baja natalidad, les daban igual.

Los progres han estado imbuidos de su propia doctrina como los primeros cristianos. Se han metido en la mente sus dogmas de un modo irreversible y su visión de la realidad es básicamente una distorsión. Sus ideas políticas se han expresado a través de la socialdemocracia, que es un diseño de Keynes basado en dar dinero a la clase obrera y apuntar la factura a la deuda pública para que la pague la generación siguiente. Y esa generación siguiente somos nosotros. Mientras tanto, ha habido barra libre para engordar obscenamente los beneficios de la banca y las grandes corporaciones.

Como toda la socialdemocracia, y por extensión todo el mundo progre, están basados en la deuda pública y en la manipulación mediática, es evidente que no van a poder continuar. Trump gana las elecciones porque Obama ha llevado a EEUU ya por encima del 100% del PIB de deuda (aparte de la expansión monetaria). Todos los periódicos, casi sin excepción, apoyaron a Hillary. Y nadie les hizo caso.

España está en la misma situación y los demás países europeos no andan muy lejos. No vamos a poder vivir bien dejando la factura por pagar a la generación siguiente, como tampoco vamos a poder mantener las paguitas y subsidios subiendo más los impuestos porque ya estamos en un nivel confiscatorio. Es un sistema que ha caído igual como la Unión Soviética. De hecho, la URSS duró 74 años. La chapuza de Keynes ha durado aún menos.

La gente de EEUU y Europa está empezando a entender que, en un mercado de oferta y demanda como el mercado de trabajo, un aporte ilimitado de mano de obra implica una caída de su precio. Además, el 53% de las mujeres blancas votó a Trump, lo que desmonta la idea de feminismo y «empoderamiento» a base de meter al marido en un calabozo y fichar en una fábrica a las ocho de la mañana. Hay la sensación (y esto lo decía también Hillary) de que no se va a vivir mejor recogiendo las migajas del enriquecimiento del 1%.

Como bien dice Roberto Centeno, hay unas «élites depredadoras» que se han apropiado de todo el crecimiento de las últimas décadas. Esto no hubiese sido posible con la antigua izquierda marxista, ni con el nazismo/fascismo/falangismo. Esto ha sido posible sólo en el mundo progre, en el mundo de la autoinhibición, de los complejos y de poner la otra mejilla, en la sociedad de las feministas, los gays y los medios alineados. Las continuas cortinas de humo en forma de moralizaciones y sermones han impedido que veamos la simple realidad: tenemos mucho menos dinero y no hay ningún motivo objetivo para que esto sea así.

Pero Trump sí que ha sabido encontrar el problema verdadero, que se llama establishment, y cortar el círculo de paguitas, limosnas y deuda pública. Ahora lo que habrá será una regulación para que las empresas compitan por la mano de obra, y no al contrario. Los obreros ya no van a poner la mano al Papá Estado, ahora tendrán más empleos, cobrarán más y el viento soplará a su favor.

El nombramiento de Steve Bannon como una especie de hombre fuerte mediático/cultural del gobierno Trump, con una influencia muy transversal, me dice que sí que hay una intención clara de hacer un cambio cultural. No va a ser Trump como el cándido de Rajoy, que aún tiene a las feministas campando a sus anchas en TVE. Trump sabe de qué modo la plutocracia controla los medios, y quiere ponerle fin a eso. Bannon será el encargado de diseñar una estrategia mediática a gran escala para desmantelar el ideario progre. Esto es una gran noticia.

Poco a poco, vamos a ver cómo los medios de línea editorial progre van a ir reduciendo su tamaño, algunos incluso cerrando, mientras que otros en la línea de Breitbart crecen y se hacen fuertes. En las universidades, encontrarán la forma de arrinconar a los profesores politizados. En la educación, se tomarán medidas para evitar el adoctrinamiento ideológico. En Hollywood, se verán más Klint Eastwood y John Wayne que Miley Cyrus y Kim Kardashian. Así como Trump supo apretar las tuercas en los estados pendulares para ganar las elecciones, ahora las sabrá apretar en la cultura para que la corriente social vaya a su favor.

Todo esto se filtrará muy pronto a Europa y España quedará como una isla en su ignorancia. Rajoy no está haciendo nada por un cambio cultural. Sólo maneja para mantenerse en el sillón a duras penas y no entiende la importancia de la cultura. Los progres siguen a sus anchas, sin plumas y cacareando. Sólo con la irrupción de un partido completamente nuevo (porque Rajoy yo pienso que se marchará cuando se muera, como Franco) podríamos empezar a revertir la situación. Pero también puede este pueblo español necio y ladrón tomar el camino contrario, ir en la dirección de Venezuela a través de Podemos y acabar a puñetazos por dos cebollas mientras se le echa la culpa al «austericidio», al imperialismo yankee y al heteropatriarcado. Hay que hacer ahora un esfuerzo de divulgación y seguir con atención lo que ocurre fuera.

16:26:37 ———————  EPUB

Felipe González y sus mentiras

Felipe González hablando de puertas giratorias:

¿Y si Clint Eastwood tuviera razón? Hacia una sociedad adolescente

http://www.vozpopuli.com/opinion/Clint-Eastwood-razon-sociedad-adolescente-correcion-politica_0_973103229.html

El irresistible avance de la corrección política es una señal muy potente que nos advierte de la infantilización de la sociedad occidental, reflejada con pavorosa nitidez en su universidad, de donde precisamente proviene.

El actor y director de cine Clint Eeastwood. Gtres

19.11.2016 – 04:00

En la genial novela de de Philip Roth, La mancha humana, la vida del decano universitario Coleman Silk se desmorona tras interesarse por dos estudiantes que han faltado a todas sus clases, “¿Conoce alguien a estos alumnos? ¿Tienen existencia sólida o se han desvanecido como negro humo?” pregunta en el aula. Desgraciadamente para Coleman, uno de los aludidos resulta ser afroamericano y, cuando llega a sus oídos la pregunta, la interpreta como un ataque racista. Aunque no había ánimo ofensivo en sus palabras, puesto que jamás había visto al estudiante, Silk es acusado de racista, cesado como decano y despedido. Sin otra universidad dispuesta a contratarlo, su economía familiar se deteriora rápidamente. Padece el rechazo de la comunidad, el repudio de amigos y conocidos y, en el colmo de la desdicha, su esposa sufre una apoplejía a causa del estrés y fallece.

Numerosos profesores norteamericanos son censurados o expulsados de las universidades porque sus discursos, o siquiera sus apreciaciones, turban a un alumnado cada vez más sobreprotegido e infantilizado

Aunque el decano Silk sea un personaje de ficción, Philip Roth refleja las vivencias de infinidad de profesores norteamericanos censurados o expulsados de las universidades porque sus discursos, o siquiera sus apreciaciones, turbaban a un alumnado cada vez más sobreprotegido e infantilizado. Porque no se ajustaban a lo políticamente correcto.

¿Universidades o jardines de infancia?

Hace poco más de dos años, según realtó Judith Shulevitz, estudiantes de la Universidad de Brown organizaron un debate abierto sobre agresiones sexuales. Inmediatamente, otro grupo de alumnos, temeroso de que los intervinientes pudieran exponer ciertas ideas “negativas”, protestó ante la dirección argumentando que la universidad debía ser un “espacio seguro” donde nada avivara los traumas de las víctimas. Las autoridades académicas no cancelaron el acto, pero pusieron a disposición de los asistentes su propio «espacio seguro»: una sala contigua donde cualquiera pudiera acudir para recuperarse de algún punto de vista turbador, y, si se sentía con fuerzas, regresar al debate. La estancia estaba equipada con cuadernos para colorear, juegos de plastilina, cojines, música relajante, mantas, galletas, chuches, incluso un video relajante en el que aparecían perritos jugando. También contaba con personal cualificado para atender posibles traumas. Cuando el evento finalizó, dos docenas de personas habían pasado por esta sala, una de las cuales explicó: «me sentía bombardeada por unos  puntos de vista que van en contra de mis creencias más íntimas».

En otra ocasión, un profesor del Columbia College recomendó la visita a una interesante exposición de arte samurai japonés. Inmediatamente, uno de sus estudiantes protestó airadamente, tachando su sugerencia de políticamente incorrecta porque podía herir la sensibilidad de los alumnos chinos. Obviamente, la objeción era absurda; la invasión de China por el ejército imperial japonés había finalizado setenta años atrás. Sin embargo, para el estudiante el tiempo transcurrido era irrelevante. Siguiendo su lógica, el arte alemán ofendería en Francia, el francés en España por la invasión napoleónica, o el español en Flandes.

Larry Summers tuvo la desgraciada ocurrencia de publicar un estudio donde mostraba que el coeficiente de inteligencia de los hombres presenta una dispersión, una varianza mayor que el de las mujeres

Otro caso llamativo es el del ex presidente de la Universidad de Harvard, el economista Larry Summers, que tuvo la desgraciada ocurrencia de publicar un estudio donde mostraba que el coeficiente de inteligencia de los hombres presenta una dispersión, una varianza mayor que el de las mujeres, planteando como hipótesis que este hecho podía influir en la asignación de puestos de trabajo en las escalas más altas y más bajas. Automáticamente fue acusado de machista y, tras una durísima campaña en su contra, Summers se vio obligado a dimitir en 2006.

Del oscurantismo a la ignorancia

El calvario de todos estos profesores ilustra la plaga de la corrección política, una moda que invade los campus universitarios del mundo desarrollado, constituyendo una asfixiante censura que, en no pocas ocasiones, provoca dramas absurdos perfectamente evitables. Lo peor, con todo, es que condena a la sociedad al oscurantismo, a la ignorancia. Al fin y al cabo, Summers sólo podría haberse ahorrado el calvario falseando los resultados de su investigación, adaptándolos a la “realidad” de lo políticamente correcto o, sencillamente, renunciando a investigar. Por su parte, el profesor de Columbia debería pensárselo dos veces antes de recomendar exposiciones de arte a sus alumnos puesto que todas, de alguna manera, herirán la sensibilidad de alguien. En cuanto a los estudiantes de la Universidad de Brown, para evitar sobresaltos tendrían que renunciar a organizar debates abiertos.

«La universidad no puede ser un ‘espacio seguro’. El que lo busque, que se vaya a casa y abrace a su osito de peluche»

Richard Dawkins

El irresistible avance de la corrección política es una señal muy potente que nos advierte de la infantilización de la sociedad occidental, reflejada con pavorosa nitidez en su universidad, de donde precisamente proviene. Tanto despropósito llevó a Richard Dawkins, profesor de biología evolutiva de la Universidad de Cardiff a advertir a sus estudiantes, con indisimulada indignación: «La universidad no puede ser un ‘espacio seguro’. El que lo busque, que se vaya a casa, abrace a su osito de peluche y se ponga el chupete hasta que se encuentre listo para volver. Los estudiantes que se ofenden por escuchar opiniones contraria a las suyas, quizá no estén preparados para venir a la universidad».

La corrección política es producto de ese pensamiento infantil que cree que el monstruo desaparecerá con solo cerrar los ojos. Pero la maduración personal consiste justo en lo contrario, en descubrir que el mundo no es siempre bello ni bueno, en la toma de conciencia de que el mal existe, en llegar a aceptar y encajar la contrariedad, el sufrimiento. Y, por supuesto, en aprender a rebatir los criterios opuestos. En su esfuerzo por hacer sentir a todos los estudiantes cómodos y seguros, a salvo de cualquier potencial shock, las universidades están sacrificando la credibilidad y el rigor del discurso intelectual, remplazando la lógica por la emoción y la razón por la ignorancia. En definitiva, están impidiendo que sus alumnos maduren.

La trampa del “espacio seguro”

Cuando se designa unos espacios universitarios como seguros, implícitamente se está marcando otros como inseguros y, por lo tanto, tarde o temprano habrá que “asegurarlos”, hasta que cualquier opinión desconcertante quede prohibida en todo el campus. Y, si esto es válido para la universidad, ¿por qué no trasladarlo a la sociedad en su conjunto? Así, la represión se extiende como mancha de aceite, prohibiendo palabras, términos, actitudes, estableciendo una siniestra policía del pensamiento.

En la práctica, es la autoridad quien acaba dictaminando lo que es políticamente correcto y lo que no. Y lo hace, naturalmente, a favor del ‘establishment’ y de los grupos de presión mejor organizados

Desde el punto de vista conceptual, la corrección política es incongruente, cae por su propio peso. Dado que no todo el mundo opina igual ni posee la misma sensibilidad, no es posible separar con rigor lo que es ofensivo de lo que no lo es, establecer una frontera objetiva entre lo políticamente correcto y lo incorrecto. Hay personas que no se ofenden nunca; otras, sin embargo, tienen la sensibilidad a flor de piel. La ofensa no está en el emisor sino en el receptor, Así, en la práctica, es la autoridad quien acaba dictaminando lo que es políticamente correcto y lo que no. Y lo hace, naturalmente, a favor del establishment y de los grupos de presión mejor organizados.

La corrección política es una forma de censura, un intento de suprimir cualquier oposición al sistema. Y es además ineficaz para afrontar las cuestiones que pretende resolver: la injusticia, la discriminación, la maldad. No es más que un recurso típico de mentes superficiales que, ante la dificultad de abordar los problemas, la fatiga que implica transformar el mundo, optan por cambiar simplemente las palabras, por sustituir el cambio real por el lingüístico.

«Es un error juvenil confundir los nombres con las cosas. Las palabras son sólo signos convencionales para identificar objetos o hechos: son estos últimos los que cuentan»

W. E. B. Du Bois

Lo expresó de forma certera el defensor de los derechos civiles W. E. B. Du Bois en 1928. Tras ser recriminado por un joven exaltado por usar la palabra «negro», Du Bois respondió: «Es un error juvenil confundir los nombres con las cosas. Las palabras son sólo signos convencionales para identificar objetos o hechos: son estos últimos los que cuentan. Hay personas que nos desprecian por ser negros; pero no van a despreciarnos menos por hacernos llamar ‘hombres de color’ o ‘afroamericanos’. No es el nombre… es el hecho». En efecto, ni la discriminación, ni el racismo, ni cualquier otro problema, se resuelven por cambiar los nombres. Como mucho, se logra tranquilizar la mala conciencia de algunos.

Y el resultado es… Donald Trump

Hay mucha gente en el mundo, demasiada en España, que, al parecer, carece de la madurez emocional o de la capacidad intelectual para escuchar una opinión política que se aparte de sus convicciones sin considerarla un insulto personal. Al poner los sentimientos por encima de los hechos, de las razones, cualquier opinión válida puede ser desactivada tachándola de racista, sexista, discriminatoria. Puede que a estas personas la corrección política les haga sentirse más cómodos, pero a costa de instaurar la cultura del miedo en los demás. Clint Eastwood declaró: «Secretamente, todo el mundo se está hartando de la corrección política, del peloteo. Estamos en una generación de blandengues; todos se la cogen con papel de fumar». Aun así no era plenamente consciente del peligro que se avecinaba: tarde o temprano el virulento efecto péndulo invierte las magnitudes, la gente acaba hastiada de tanta censura, y como reacción… vota a Donald Trump.

Renunciar al libre discurso, al libre pensamiento, para evitar herir la sensibilidad de algunos es peor que estúpido: es peligroso porque pone en cuestión los principios de la democracia. Debemos ser respetuosos con todo el mundo, por supuesto. Pero también expresar con libertad nuestras ideas y argumentos. Si alguien se molesta, se rasga las vestiduras, es muy probable que esté mostrando su talante inmaduro, su carácter infantil e intolerante. Lo advirtió George Orwell en su novela 1984: «La libertad es el derecho de decir a la gente aquello que no quiere oír».

Siberia un congelador a pleno rendimiento en el otoño de 2016

http://www.cazatormentas.net/siberia-un-congelador-a-pleno-rendimiento-en-el-otono-de-2016/

Diferencia de temperatura de las previsiones normales según el modelo Europeo el 17 de noviembre (WeatherBell.com)

El otoño de 2016 está dejando en el Hemisferio Norte profundos desajustes térmicos. En más de una ocasión os hemos hablado de la importante anomalía térmica positiva que está experimentando el Ártico estas semanas.

Pero no menos notable es la desviación térmica negativa que está sufriendo buena parte de Asia y zonas aledañas de Europa. Anomalías de hasta 50 ºF (unos 27 ºC) respecto a lo esperado para esta época del año. Siberia es un congelador a pleno rendimiento, cerrado, que puede influir de forma transcendental en nuestra atmósfera en las próximas semanas cuando esa puerta se abra y el frío se extienda a otras zonas del Hemisferio Norte. De hecho, en esta otra entrada, podéis ver como repercutirá esta situación en nuestro tiempo durante los próximos días, al quedar el vórtice polar muy alterado como consecuencia de ese caldeamiento del Ártico.

Siberia es un auténtico congelador

Este otoño Siberia está actuando como un auténtico congelador. En aquella parte del Planeta están registrando temperaturas negativas muy acusadas, incluso muy bajas para aquella zona a esta altura del calendario.

El invierno más crudo aún no ha llegado y en estos momentos están instalados en un ambiente muy riguroso. Y como recogen estos mapas, las temperaturas más frías de lo normal se han llegado a extender por muchas zonas de Eurasia.

Estas temperaturas mínimas se alcanzaron el pasado 6 de noviembre

Estocolomo: nevada de récord en noviembre

Aunque con mayores fluctuaciones térmicas, los países escandinavos también han sufrido episodios fríos importantes, aunque lo más llamativo es la nevada de récord que se registró en Estocolmo el pasado 9 de noviembre de 2016.

En 111 años de registro no se había medido una nevada tan importante como la que llegó a la capital sueca en el arranque de noviembre. Pese a ser un país acostumbrado a las inclemencias invernales, los habitantes de la zona quedaron sorprendidos por una nevada tan intensa cuando aún estamos a mitad de noviembre.

Ver imagen en Twitter

Seguir

#eustorm @EUStormMap

NEW SNOW RECORD Stockholm’s snowiest day in more than 100 years! 9.11.16 Img Via Partners @severeweatherEU 18/30 #eustorm

18:12 – 10 nov 2016 · Stockholm, Sweden

• 101101 Retweets

• 8686 me gusta

Seguirá Siberia almacenando frío…

Si las previsiones a largo plazo se cumplen, todo apunta a que Siberia seguirá acumulando frío durante las próximas semanas.

Ver imagen en Twitter

Seguir

Judah Cohen @judah47

Am I the only one impressed with the large reservoir of cold in Eurasia? Still no signs of abating.

16:14 – 11 nov 2016

• 2626 Retweets

• 5454 me gusta

De momento parece que las altas presiones no se van a desplazar de la zona, lo que favorecerá que el aire continúe estático en aquella parte del Planeta guardando más y más frío.

Esta perspectiva una Siberia helada y un Ártico cálido podría favorecer el mantenimiento de un índice AO negativo lo que a su vez debería repercutir en que el vórtice polar siga débil, como se muestra este otoño. Ya sabéis que si el Ártico se calienta, Europa se enfría…

Ver imagen en Twitter

Seguir

En Babia @Las_Quimbambas

Temperaturas en la topografía de 850 hPa, mañana a las 00 UTC: embolsamiento de hasta -28 ºC en #Siberia central

Aunque es muy complejo establecer una causa-efecto directa sobre nuestra atmósfera, estas condiciones podrían propiciar un tiempo inestable y frío en nuestra zona en los próximos meses.

En este gráfico, hemos tratado de resumir esa conexión. Se trata de lo que denomina una teleconexión  entre la troposfera y la estratosfera, a partir de la propagación de la temperatura en la superficie de Eurasia hacia capas superiores de la atmósfera durante el otoño, condicionado a su vez el estado de la estratosfera meses después el tiempo en invierno. Un ciclo se puede definir en 6 pasos

15 nov

Pedro C. Fernández @PedroCFernandez

Las anomalías en el #Artico crecen mientras #Siberia es un congelador ¿invierno crudo en #Europa? http://lsh.re/19DV0 pic.twitter.com/SYdKaw2mRs

Seguir

Iñaki Ramos Gorospe @Gorospe1992

@PedroCFernandez @LaMeteoAstur si a eso le sumamos que el Jet Stream va quedar hecho unos zorros a finales de mes, «huele» a siberiana…. pic.twitter.com/4apn1MQskO

15:46 – 15 nov 2016

• Retweets

• 22 me gusta

La teoría apunta a que un inicio de otoño nivoso y frío en buena parte de Eurasia, junto a un Ártico más cálido de lo normal debe traernos en invierno una atmósfera muy dinámica a España, con situaciones propicias tanto para las lluvias como para la llegada de advecciones de aire frío árticas o siberianas.

Este escenario nos dejaría, en el norte de la Península Ibérica, un invierno no demasiado frío, pero sí muy húmedo https://t.co/VgC7hQpfGC

— David Arango (@arangometeo) 15 de noviembre de 2016

AO Negativo

Como sabéis, el índice de Oscilación Artica es una medida del patrón dominante de la presión a nivel del mar a la latitud de 20N.

Un índice AO negativo se asocia a ondulaciones del jet stream acusadas, con altas presiones dominantes en el Polo Norte. Esto trae como consecuencia la irrupción de aire frío polar a distintas regiones del hemisferio Norte a baja latitud, que pueden traer temporales de nieve o lluvia a zonas más sureñas, como la Península Ibérica.

Un índice AO positivo, se asocia a bajas presiones dominantes en el Polo Norte y a una corriente en chorro menos ondulada que circula de forma estable a una latitud media-alta. De esta forma es de esperar un tiempo más húmedo en Alaska, Reino Unido, Escandinavia… y más seco en Estados Unidos o el Mediterráneo.

De momento, ese cinturón anticiclónico más la retirada de las altas presiones de las Azores, parece que nos va a traer el primer temporal de lluvia importante generalizado del otoño. Mucho tienen que cambiar los mapas para que esto no suceda… Aquí os estamos informando al minuto de ese posible escenario para la semana que viene.

Ciclo con baja cobertura de nieve en Eurasia al inicio del otoño

¿Qué ocurriría hipotéticamente en una situación inversa? Este otro gráfico, indicaría lo que podríamos encontrar en inviernos que son precedidos de octubres cálidos en Eurasia.

Veremos si estas relaciones tan complejas que vemos reflejadas de manera simplificada en estos gráficos se ven cumplidas en las próximas semanas y meses.

Una historia triste: El hombre que borró sus huellas hasta desaparecer

¿Quién era Peter Bergmann? El hombre que borró sus huellas hasta desaparecer

Un hombre semi-desnudo en una playa y ni una sola huella que permita identificarle. Como el caso de Tamam Shud en Australia ocurrido tras la Segunda Guerra Mundial, el misterio del hombre de Sligo sigue los mismos patrones. Con una diferencia. Ahora era el 2009 y las cámaras CCTV grabarían todos sus pasos.

En una época como la actual, rodeados de tanta tecnología, uno podría pensar que resulta tremendamente difícil desaparecer sin dejar huellas de ningún tipo. El caso de Jim Gray es quizá una de esas raras excepciones de casos sin resolver. En la historia que hoy nos ocupa, el tipo que decía llamarse Peter Bergmann se las ingenió para que todo el mundo fuera partícipe del borrado de su identidad.

Si bien el final del relato parece explicar las razones del viaje, la travesía que emprendió este hombre para desaparecer sin dejar rastro se mantiene como uno de los grandes misterios de los últimos tiempos.

El cuerpo en la playa

Rosses Point. Wikimedia Commons

Al noreste de Irlanda se encuentra la pequeña ciudad costera de Sligo. Pequeña en cuanto a tamaño, porque estamos ante el segundo mayor centro urbano de la provincia de Connacht, hoy convertida en centro cultural, histórico, industrial, comercial y de servicios de gran influencia regional. Además y debido a su localización, la ciudad es un destino turístico muy popular, principalmente por su gran variedad y belleza natural de la zona.

En el extremo este de la ciudad nos encontramos con Rosses Point, una pequeña villa y playa que cubren toda la zona. Un lugar de temperaturas normalmente frías que iba a deparar una misteriosa sorpresa el 16 de junio del 2009.

Cuando llegaron hasta el cuerpo los peores presagios se hicieron realidad. El hombre no respondía a los intentos de llamada de Arthur y Brian

Era una mañana agradable, de cielo despejado y temperaturas altas para lo que viene siendo habitual incluso en junio. El día anterior Arthur Kinsella había planeado salir a correr a la playa temprano con su hijo Brian. Ambos se estaban preparando para un triatlón que tendría lugar en pocos meses. Cuando padre e hijo comienzan el entrenamiento divisan una extraña figura a lo lejos. Eran las 06:45 de la mañana.

Desde el lugar en el que se encontraban parecía una persona desnuda muy cerca del mar, casi en la orilla. Podría estar durmiendo, pero a esa hora de la mañana resultaría muy extraño, quizás podría tratarse de alguien en problemas, pensaron. Cuando llegaron hasta el cuerpo los peores presagios se hicieron realidad. El hombre no respondía a los intentos de llamada de Arthur y Brian. Arthur le toma el pulso y no nota nada. Inmediatamente llaman a la policía.

A las 08:10 es la doctora Valerie McGowan quien declara al hombre oficialmente muerto. A partir de entonces comenzaría una ardua búsqueda por identificar aquel cadáver y las causas que lo llevaron hasta allí.

Cinco meses de una investigación llena de sorpresas.

Un viaje sin retorno

Sligo. Wikimedia Commons

Mientras el cuerpo estaba siendo analizado por los forenses la policía comienza la investigación paralela para intentar resolver el rompecabezas. El cuerpo del hombre en la playa se había encontrado semi-desnudo en la arena mojada, con unos calzoncillos de color negro, descalzo y una camiseta en el torso. Pero no había nada más a su alrededor. La policía encontró horas después, a unos 300 metros de la zona, lo que parecían sus efectos personales. Según publicaron se trataba de:

• Unos zapatos.
• Unos calcetines.
• Unos pantalones.
• Un jersey.
• Una chaqueta de cuero negra.
• Un paquete de pañuelos.
• Una suma de dinero en efectivo.
• Unas hojas de papel en blanco.
• Unas tabletas de aspirina.
• Un reloj de pulsera.

Ni rastro de una tarjeta o DNI que pudiera identificarle. Únicamente había una pista que les decía algo acerca de este misterioso personaje en la playa. Se trataba del jabón del hotel, lo que finalmente les condujo a reconstruir los pasos del hombre los días anteriores. Todo gracias a las numerosas cámaras CCTV que se pueden encontrar en los comercios y espacios públicos junto a los informes de los testigos que entraron en contacto con el misterioso personaje.

El cuerpo del hombre en la playa se había encontrado semi-desnudo en la arena mojada, con unos calzoncillos de color negro, descalzo y una camiseta en el torso

Se trataba de un tipo delgado, de pelo corto gris/blanco, probablemente de entre 50 y 60 años. Su estatura era de 1,78 centímetros, ojos azules y tez morena. Los primeros testigos ya hablaban de un tipo extranjero, su acento parecía delatarlo como alemán. Un señor que vestía perfectamente arreglado y aseado, con el rostro afeitado y el cabello limpio y peinado.

Las cámaras mostraban a un tipo que vestía una chaqueta de cuero negra, unos pantalones azules, unos calcetines azules también, un cinturón de cuero negro y par de zapatos negros, todo ello y como vemos, parte de las pertenencias encontradas en las cercanías de la playa.

Otro detalle en el que se fijaron era que la marca de toda la ropa era de C&A, una compañía de moda bastante famosa en Europa, siendo Alemania y Austria los sitios donde hay más tiendas. Los investigadores dirían que por su apariencia parecía un tipo con trabajo. Por último y según pudieron recoger en los primeros vídeos de las cámaras, se trataba de un fumador empedernido.

Seguimiento de las cámaras

Your browser does not support HTML5 video tag.

El primer vídeo del que se tiene constancia del tipo es del viernes 12 de junio. En ese momento las cámaras lo captan por primera vez en la parada de autobuses del aeropuerto de Derry, ciudad de Irlanda del Norte. Desde allí se dirige hasta la estación de autobuses de la ciudad, lugar donde las cámaras lo vuelven a captar a las 16:00 del viernes.

A continuación se observa como toma un autobús con dirección a la estación de Sligo. En este momento se puede apreciar que porta una bolsa negra en el hombro junto a una maleta de equipaje de mano estándar. A las 18:28 las cámaras capturan la llegada del autobús y el misterioso hombre a la estación de Sligo. Desde allí toma un taxi hasta el Hotel Sligo City.

Your browser does not support HTML5 video tag.

Aquí entra en contacto con la recepcionista del hotel. El hombre, muy educadamente, se registra bajo el nombre de Peter Bergmann pagando en efectivo por tres noches. Además ofrece su dirección o residencia actual como Ainstettersn 15, 4472, Viena, Austria. Cuando la policía llega hasta esta pista rápidamente investigan por el nombre y la dirección. Desgraciadamente, quien quiera que fuera ofreció unos datos falsos, no existía ni el señor Bergmann ni la dirección ofrecida. Dicho de otra forma, aquella fue la primera señal que parecía indicar que el hombre quería permanecer en el anonimato.

Al día siguiente, el sábado 13 de junio, el falso Bergmann es captado por las cámaras de varios comercios. Ese día había salido temprano del hotel y se dirigió a la oficina de correos a las 10:49. Allí compró ocho sellos de 82 centavos junto a varias pegatinas de correo aéreo. Los investigadores creen que presumiblemente estaba escribiendo una serie de cartas a sus seres queridos, ¿se estaría despidiendo?

Your browser does not support HTML5 video tag.

El domingo 14 de junio, entre las 11:00 y las 11:30 el tipo deja el hotel Sligo City. Antes y como recordarían los empleados del hotel solicita un taxi. Una vez dentro del vehículo el hombre le pregunta al taxista por alguna recomendación, una playa bonita y tranquila a la que pudiera ir y nadar. El taxista le recomienda Rosses Point y le dice que es el mejor lugar que conoce. El falso Bergmann le pide al taxista que lo lleve hasta allí.

Cuando llegan a la zona Bergmann le pide al taxista que espere unos segundos. Con tranquilidad se baja del taxi y en absoluto silencio se queda mirando la postal de la playa durante unos minutos, parecía como si estuviera analizando la zona. Tras este breve espacio de tiempo Bergmann asiente con la cabeza, vuelve al coche y le pide al taxista regresar a la ciudad, en este caso a la estación de autobuses de Sligo.

Your browser does not support HTML5 video tag.

Pero había un detalle que no se le había escapado a los investigadores. Tanto el sábado como el domingo el hombre había salido del hotel con una bolsa de plástico de color violeta. Las cámaras CCTV lo captaban saliendo con ellas, pero cuando regresaba de sus largos paseos ya no volvía con dicha bolsa. ¿Por qué?

El rastreo exhaustivo posterior de las cámaras de vigilancia de la ciudad mostrarían a Bergmann deshaciéndose de lo que fuera que llevara en las bolsas. Lo hacía en diferentes puntos de la urbe y jamás se pudo identificar qué era lo que tiraba a los contenedores, entre otras cosas, porque el misterioso hombre lo hacía siempre en los puntos ciegos de las cámaras de vigilancia. Era, sin ninguna duda, unos movimientos meticulosos, metódicos y estudiados, como si supiera dónde iba a esconder sus pertenencias sin que lo pudieran identificar. Este fue el móvil que siempre barajó la policía.

Your browser does not support HTML5 video tag.

El lunes 15 de junio nuestro hombre misterioso sale del hotel al mediodía. El reloj marcaba las 13:06. En el hall del hotel deposita las llaves de la habitación y sale por la puerta principal con una bolsa negra al hombro (la misma con la que había llegado a la ciudad), la bolsa de plástico violeta y una nueva maleta de mano de color negra. No era la misma con la que había llegado a Sligo.

La ruta que siguió captada por las cámaras le llevó hasta la estación de autobuses, lo hizo a través de Quay Street, luego Wine Street y a mitad de camino se detiene en el centro comercial Quayside, espacio en el tiempo donde pasa unos minutos.

Cuando el reloj marcaba las 13:16 se marcha del centro comercial y camina en dirección a la estación, todavía con las tres bolsas. A las 13:38 el tipo llega a la estación y se puede observar como entra en un café. Los trabajadores del local recuerdan que pide un capuccino y un sándwich de jamón y queso. Ya fuera del local se aprecia como el falso Bergmann disfruta de la comida mientras se dedica a mirar los papeles que guarda en sus bolsillos.

Your browser does not support HTML5 video tag.

Cuando acaba de comer rompe el papel por la mitad y lo tira en una basura cercana. Luego se monta en un autobús que parte de la estación a las 14;20 con dirección a Rosses Point. No fue lo último que se supo de él. Al menos 16 personas informaron que lo vieron mientras caminaba ese día por la playa. Además, casualmente Bergmann o como fuera que se llamara, se mostró afable con muchos de ellos saludándoles cuando pasaban a su lado.

Y aquí se pierde la pista de las últimas horas del hombre. A la mañana siguiente Arthur y su hijo Brian encontraban su cuerpo en la playa. ¿Por qué? El informe forense declaró que probablemente se había ahogado, aunque las causas siguen siendo un misterio. Los médicos no encontraron señales de ningún tipo que pudieran sugerir que la muerte del hombre fue un homicidio.

A la mañana siguiente Arthur y su hijo Brian encontraban su cuerpo en la playa. ¿Por qué? El informe forense declaro probablemente se había ahogado, aunque las causas siguen siendo un misterio

Pero a pesar de lo bien vestido y afable que se mostró exteriormente en determinadas circunstancias, el hombre estaba en muy mala salud. La autopsia reveló que se encontraba en una etapa muy avanzada de cáncer de próstata junto a varios tumores óseos. Su corazón mostraba claros signos de ataques cardíacos previos y que poseía un único riñón, el otro anteriormente extirpado.

Para un hombre bajo estas condiciones de salud tan sumamente graves, lo más extraño de todo era que el informe toxicológico demostró que no estaba bajo ningún tipo de medicación. El médico forense indicó que debido a sus ataques cardíacos previos y a su debilitado estado de salud, lo normal era que aquel tipo habría estado padeciendo un gran dolor durante mucho tiempo, un estado que normalmente se trata de paliar con medicamentos prescritos, o como mínimo, con analgésicos que ayuden a manejar estos niveles de dolor.

Así que tras cinco meses de investigación, el hombre sin nombre, el falso Bergmann, era enterrado en la ciudad de Sligo a la espera de que alguien lo reclame. No parece que eso vaya ocurrir después de varios años. Su viaje a la ciudad parecía indicar que aquel tipo había decidido que Sligo y la playa de Rosses Point serían el lugar desde el que decir adiós.

Jamás se supo porqué decidió morir sin un nombre o la razón que le llevó a eliminar todas las huellas que pudieran descubrir su identidad. Ese misterio se lo llevó a la tumba y ni siquiera las autoridades europeas podrán averiguarlo. No existe una notificación de la Interpol para el hombre sin nombre porque el cuerpo se recuperó, razón por la que no puede ser “persona desaparecida” o “persona bajo búsqueda”.

Solamente a su país de origen le corresponde denunciarlo como desaparecido.

 

Ikea crea un carril rápido para solteros

http://www.elmundotoday.com/2016/11/ikea-crea-un-carril-rapido-para-solteros/

LOS CLIENTES CON PAREJA PODRÁN USAR EL CARRIL A LA TERCERA DISCUSIÓN, CUANDO LA RUPTURA SEA IRREVERSIBLE

Por Javi Ramos

 

Con el fin de que las parejas que discuten y bloquean los pasillos no ralenticen a los clientes solteros, el fabricante de muebles Ikea ha decidido habilitar un carril rápido para solteros en todos sus establecimientos. “Muchos se quedaron sin pareja tras una visita a Ikea, es lo mínimo que podemos hacer por ellos”, ha declarado esta mañana Tolga Öncü, director general de Ikea Ibérica y lámpara de techo.

“Las discusiones matrimoniales en los pasillos solían crear embudos y se formaban largas retenciones que en ocasiones colapsaban varias secciones”, constata Godmorron Martínez, de la sección de dormitorios. “Con tantas aglomeraciones se perdían muchos niños y luego había que reutilizarlos como muebles o cajeros”, asegura Isfjorden Rodríguez, de menaje.

Asimismo, la empresa ha construido una rotonda en la sección de plantas para facilitar la incorporación de los solteros a la línea de cajas y, con el objetivo de evitar que algún marido se aproveche de la iniciativa, los clientes que entren con pareja sólo podrán sumarse al carril de solteros tras la tercera discusión, cuando la ruptura de lazos afectivos sea irreversible. Un asesor matrimonial a sueldo de la empresa será el encargado de dictaminar si la pareja está completamente rota o si todavía está abierta a una segunda oportunidad.

A partir de ahora, los solteros que se conozcan en Ikea podrán intimar en las camas de la sección de dormitorios con la idea de que acaben formando una nueva pareja que vuelva a Ikea y acabe rompiendo en el establecimiento, perpetuando así el ciclo de enamoramiento y ruptura que ha convertido la empresa en líder de su sector.

Los mejores libros que puedes leer sobre matemáticas

http://www.microsiervos.com/archivo/ciencia/los-mejores-libros-sobre-matematicas.html

POR @ALVY — 12 DE NOVIEMBRE DE 2016

Esta selección proviene de Goodreads; habiendo leído más o menos la mitad tan solo puedo concordar con ella. Es una lista ideal para quien quiera descubrir, aprender y maravillarse con las matemáticas:

LOS LIBROS MÁS POPULARES DE MATEMÁTICAS

1. Gödel, Escher, Bach: un Eterno y Grácil Bucle(Douglas R. Hofstadter, 1979/1995). ¿Pueden un sistema o una mente comprenderse a sí mismos? Una cuestión clave del pensamiento científico, de la filosofía y del arte, examinada a través de tres genios: el matemático Kurt Gödel, el artista M.C. Escher y el compositor Johann Sebastian Bach. Obtuvo el Premio Pulitzer, 1980. [Sobre el autor.]

2. Planilandia: un romance en muchas dimensiones(Edwin Abbott, 1884). Planilandia es el mundo en el que el protagonista de la obra (A. Square, «A. Cuadrado») describe las costumbres y la física de un universo plano en dos dimensiones. El protagonista es visitado de repente por un ser de la «tercera dimensión» y esto le abre un nuevo mundo desconocido para él hasta ahora – no exento de problemas. [Reseña de la primera y segunda partes de la novela.]

3. El enigma de Fermat (Simon Singh, 1997/2015). «He descubierto una demostración verdaderamente maravillosa, pero este margen es demasiado estrecho para contenerla». La historia de uno de los más famosos problemas –y su solución– a lo largo de varios siglos. [Sobre la solución del problema y entrevista con el autor.]

4. Cero: biografía de una idea peligrosa (Charles Seife, 2006). «Los babilonios lo inventaron, los griegos lo proscribieron, los indios lo veneraron y la Iglesia católica lo esgrimió para atajar herejías». La historia del número cero, un concepto aparentemente inofensivo pero que resultaba contrario a la intuición de muchas civilizaciones antiguas aunque tuvieron que enfrentarse a él en algún momento para prosperar en las ciencias. [Reseña.]

5. The Man Who Loved Only Numbers: The Story of Paul Erdos and the Search for Mathematical Truth(Paul Hoffman, 2001). Un retrato del matemático húngaro Paul Erdős: genio excéntrico y hombre campechano tras el cual están algunos de los más sorprendentes descubrimientos de la matemática moderna. [Existe una versión en castellano descatalogada: El hombre que solo amaba los números.]

6. How Not to be Wrong: The Hidden Maths of Everyday Life (Jordan Ellenberg, 2014). Un manifiesto sobre la utilidad de las matemáticas en la vida cotidiana. Calificado del «Freakonomics de las matemáticas» trata desde las bases de las matemáticas más sencillas a su aplicación formal y mediante trucos y otras técnicas fáciles de entender.

7. Los códigos secretos (Simon Singh, 2000). Una breve historia de la criptografía y el criptoanálisis, así como sus implicaciones –casi siempre decisivas– de los «códigos secretos» a lo largo de la historia de las diversas civilizaciones. [Está a la altura de The Codebreakers, aunque no alcanza al clásico de David Khan.]

8. El placer de la X: una visita guiada por las matemáticas, del uno al infinito (Steven Strogatz, 2013). Un recorrido por diversas ramas de saber y su relación con las matemáticas a través de sorprendentes conexiones con la literatura, la filosofía, la medicina o el arte, no siempre evidentes pero siempre llamativas.

9. El universo de las matemáticas: un recorrido alfabético por los grandes teoremas, enigmas y controversias (William Dunham, 2006). Los grandes teoremas, enigmas, controversias y misterios irresueltos que han conformado el fascinante mundo de las matemáticas, desde hace cinco mil años hasta nuestros días.

10. La música de los números primos (Marcus Du Sautoy, 2003/2013). Este libro es un recorrido por la vida de algunos de los más grandes matemáticos de la historia y sus trabajos sobre la Hipótesis de Riemann y su misteriosa y a la vez extraña relación con los números primos. [Reseña; sobre el problema más famoso relacionado con los números primos: The Riemann Hypothesis de Karl Sabbagh es el más completo.]

11. La proporción áurea: la historia de phi, el número más sorprendente del mundo (Mario Livio, 2008). La historia del número Φ a lo largo de los tiempos, sus características matemáticas y cómo se fueron aplicando en diversas áreas, así como los méritos de todas sus «supuestas apariciones» a lo largo de la historia en todo tipo de contextos. [Reseña.]

12. El andar del borracho: cómo el azar gobierna nuestras vidas (Leonard Mlodinow, 2010). Matemáticas, probabilidad, la forma de entender las encuestas, el juego, el riesgo, los acertijos que desafían a la intuición y decenas de temas relacionados. [Un ejemplo del libro.]

13. El hombre anumérico (John Allen Paulos, 1988). «Yo es que soy de letras». Cómo el desconocimiento de las matemáticas no solo nos afecta personalmente en cuestiones avanzadas y complejas sino también en la vida cotidiana, casi siempre en nuestra contra. [Por aquí hemos publicado varios de los problemas planteados en el libro.]

(Vía @pickover.)

Por qué el imbécil no era Trump sino los que se reían de él

No ha habido trabajo más fácil en 2016 que el de presentador de informativo satírico de la televisión estadounidense: bastaba con decir que Donald Trump era un imbécil (y sus potenciales votantes más cretinos todavía) para que la audiencia progresista se rompiera las manos a aplaudir. Lo curioso es que el modus operandi de la prensa seria y de la campaña de Hillary Clinton para combatir a Trump ha sido muy parecido: superioridad moral contra la (denunciaban satisfechos) idiocia trumpista. ¿Pero quién es aquí el imbécil en realidad?

El año electoral ha consistido básicamente en jugar al juego propuesto por Trump, el de la batalla cultural (o sea, choques entre conservadores y progresistas en torno a la moral, las costumbres y los estilos de vida). En efecto, la campaña que ha llevado a Trump en la Casa Blanca ha sido el ejemplo más depurado y chirriante vivido hasta ahora de guerra cultural. Trump ha jugado a fondo la baza de incendiar al progresismo cultural a golpe de improperio. No ha quedado minoría racial por difamar, feminista por insultar, ni izquierdista por soliviantar… Trump ha ganado esta refriega por KO técnico; entre otras cosas, por la suficiencia moral con la que el bloque progresista entró al trapo. Obviamente la batatilla cultural no ha sido el único motivo de la victoria de Trump -la incapacidad de los demócratas para leer el malestar social pasará a la historia- pero sí ha sido un factor clave.
La élite progre

Contexto: En los años noventa, los neocon estadounidenses convirtieron la guerra cultural en una lucha de clases a la inversa: las élites ya no eran los ricos sino los esnobs progresistas; esos neoyorquinos cosmopolitas que vivían a lo grande, veían cine afrancesado y se burlaban de los granjeros precarios del Medio Oeste por sus hobbies -las carreras de coches de la Nascar- y sus opiniones conservadoras sobre moral sexual.

El contraataque cultural necon triunfó porque -al margen de su chusca distorsión de la lucha de clases a golpe de resentimiento- se apoyaba en malestares reales, y hasta tuvo algo de profecía autocumplida: un cuarto de siglo después, “progre” y “élite” se han convertido en sinónimos. ¿Hay algo más mainstream (y más calcinado) hoy día que figuras de grandes partidos progresistas internacionales como Felipe González y Hillary Clinton?

Por otro lado, el descrédito de los vips culturales progresistas como prescriptores políticos ha alcanzado máximos históricos en 2016: la farándula progre internacional clamó contra el Brexit y contra Trump, con el éxito ya conocido, incapaces de dinamizar una alternativa política a la austeridad que fuera más allá del ‘votad a los nuestros hagan lo que hagan, que los otros son imbéciles y están locos’.

No es extraño que el escritor y periodista Thomas Frank, el mayor experto estadounidense en guerras culturales, fuera uno de los primeros en alertar en marzo de este año de que ridiculizar a Trump en lugar de tomárselo en serio no era buena estrategia. Y es que, el trumpismo era una versión mejorada de las guerras culturales noventeras de los neocon: al discurso antiprogre tradicional se le sumaba una novedosa andanada contra el establishment financiero. La derecha se echaba al monte en plena era del malestar social. Trump era un enemigo mucho más temible de lo que parecía. O los demócratas lograban contrarrestar políticamente los cañonazos del trumpismo contra el daño que la deslocalización y el libre comercio habían hecho al cinturón industrial de EEUU… o iban a tener un serio problema.

No sabemos admitir que nosotros, los de ideas progresistas, tenemos alguna responsabilidad en el ascenso de Trump

“Los mensajes de Trump dan forma al contraataque populista contra el liberalismo que ha ido cobrando forma lentamente durante décadas y podría llegar a ocupar la Casa Blanca, cuando todo el mundo se verá obligado a tomar en serio sus locas ideas. Sin embargo, aún no podemos afrontar esta realidad. No sabemos admitir que nosotros, los de ideas progresistas, tenemos alguna responsabilidad en el ascenso de Trump, a causa de la frustración de millones de personas de clase trabajadora, de sus ciudades arruinadas y sus vidas en caída libre. Es mucho más fácil burlarse de ellos por sus almas retorcidas y racistas, y cerrar los ojos ante la evidente realidad de la que el trumpismo es sólo una expresión vulgar y cruda: que el neoliberalismo ha fracasado por completo”, escribió Frank hace ocho meses. ¿La respuesta de los demócratas? Elegir al candidato más establishment que tenían a mano -Hillary- para combatir a un movimiento basado en el odio al… establishment. De traca, sí.

Hillary Clinton ha sacado cerca de 10 millones de votos menos que Obama en 2008, y 6 millones menos que Obama en 2012; y eso que competía contra un candidato -Trump- que generaba toneladas de rechazo y pavor. O el progresismo fofo como imparable rodillo desmovilizador en un contexto de hartazgo explosivo. Y luego que si Bernie Sanders está fuera de la realidad…

Thomas Frank ha publicado ahora un artículo en ‘The Guardian’ en el que concluye lo siguiente sobre el ‘clintoncidio’: “La América de cuello blanco se ha pasado todo el año insultando y silenciando a todo aquel que no compartiera su valoración. Y resulta que han perdido. Quizá ha llegado la hora de valorar si su estridente santurronería, bramada desde un estatus de clase alta, echa para atrás a la gente. Un problema aún mayor es que una especie de complacencia crónica echó raíces hace años años en el progresismo americano… Es el progresismo de los ricos, que primero falló a las clases medias, y ahora ha fallado electoralmente”.

Resultados Elecciones Estados Unidos 2016: Por qué el imbécil no era Trump sino los que se reían de él. Blogs de Animales de compañía

Inger Enkvist: «Hoy las escuelas son guarderías para adolescentes»

http://www.elmundo.es/cronica/2016/11/11/581f0cde268e3e502d8b456c.html

Su discurso no casa muy bien con los recientes entusiastas de la nueva educación, la que cree que los niños se aburren en clase por culpa de un sistema que no ha cambiado tan rápido como la tecnología. O sea, muchos de los acólitos del educador británico Sir Ken Robinson, los que piensan que los niños ya no pueden permanecer sentados en clase, los que creen que los colegios matan la creatividad, los que abominan de una mínima memorización.
Además, para los que confían en que un pacto educativo de todos los partidos es la panacea para los problemas de fracaso escolar que padecemos, Inger Enkvist no trae buenas noticias: quizás es más cuestión de lo que se respira en el ambiente, en casa, que en la letra pequeña de una nueva ley, como han demostrado los distintos estudios de los que ella se hace eco en La buena y la mala educación sobre distintas comunidades de inmigrantes por todo el mundo. Es incómodo pero, ¿por qué los descendientes de chinos lo hacen mejor que los de mexicanos en California con exactamente el mismo sistema educativo?

En España, hace años, la llamaron para escuchar su opinión sobre la ley catalana de Educación. Allí, en el Parlament, habló de resultados de la educación diferenciada entre niños y niñas. Pero un parlamentario de Esquerra le dijo que los colegios femeninos y masculinos en este país eran un símbolo de la Iglesia y de la dictadura. Quizás por eso, porque sabe lo fácil que se embarra aquí el debate, Enkvist se muestra cauta a la hora de abordar algunos asuntos. De los otros… aquí está ella.

Cuando lee o escucha que hay que cambiar la educación porque seguimos dando clase como en el siglo XIX, antes de que existiera Google, una de las frases más recurrentes entre los gurús de la nueva educación, ¿qué piensa?

Eso lo dice alguien que no es educador. En la educación de base, fundamentalmente la obligatoria hasta los 16 años, lo que necesitan aprender los alumnos no tiene que ver con Internet. Necesitan lengua, matemáticas, geografía, historia… Necesitan convertirse en buenos lectores con un amplio vocabulario y una sólida base de conocimientos generales sobre el mundo. Para conseguir esto, Internet es más una distracción que una ayuda. Internet les va a servir después de conseguir esa formación de base. Es magnífico para la persona que ya tenga conocimientos y necesite comprobar un dato o la ortografía de un nombre. Para sacar provecho de la Red, el usuario debe saber formular una pregunta y evaluar la respuesta, y eso no es el caso del alumno promedio. Se podría hablar también de un error de percepción. Hay mucho material en Internet, es cierto, pero el problema del educador de hoy no es tanto tener acceso a materiales para ilustrar lo que deben aprender los alumnos como el problema de la voluntad de hacer un esfuerzo prolongado para aprender.

En muchos países occidentales, por las encuestas de Pisa sabemos que los niños de familias sin recursos económicos apenas tienen oportunidad de llegar a la universidad. ¿Qué está pasando? La educación ha dejado de ser un ascensor social…

Tiene que ver con varias cosas pero se pueden señalar unos factores. El primero sería que, en el Estado del bienestar, los alumnos no sienten la urgencia de antes de estudiar para sobrevivir social y económicamente, y tampoco los padres ven así la situación. Segundo, con el Estado del bienestar se han difundido teorías permisivas en la educación que dicen que los profesores deben aceptar que no haya tanta disciplina en clase. Se dice que el aprendizaje debe ser divertido y, si no es así, los alumnos están en su derecho de rechazarlo. Esas teorías son negativas para todos, pero sobre todo para los que aprenden menos en su casa. Finalmente, esos factores han llevado a que no sea tan gratificante ser profesor, con lo cual los estudiantes más inteligentes e idóneos para ser profesores se dirigen hacia otras profesiones. Los nuevos docentes quizá no tengan la misma capacidad de ganarse a los alumnos con menos interés para los estudios.

En su libro de La buena y la mala educación, describe la educación en barriadas francesas como aterradora, con adolescentes que apenas se sitúan en el mundo. ¿Cómo se ha llegado a eso?

Mi explicación es que grupos de intelectuales han machacado a políticos y profesores diciendo que Occidente sería opresora y que su cultura sería «excluyente». Esto ha creado una duda de si es legítimo enseñar lo que se enseña desde siempre y en particular si es legítimo exigir que personas de otro origen étnico aprendan lo que típicamente contiene el currículum en una escuela occidental. El resultado es que los profesores no exigen un esfuerzo, los alumnos no aprenden y, a pesar de esto, obtienen el aprobado, y los políticos no intervienen porque no se sienten seguros de su mandato para intervenir. Se invierte un dineral en las escuelas pero no se obtienen resultados y se convierten en guarderías para adolescentes. Tras la edad escolar, estos jóvenes están desamparados ante la vida en una sociedad moderna.

Estudiantes aislados

Cuando dice que en esas barriadas de París están desamparados y perdidos en el mundo, ¿podría explicar en qué sentido? Y no pasa tanto con las chicas musulmanas. ¿Por qué cree?

Si los alumnos del origen que sea no adquieren en la educación primaria un vocabulario amplio, unos conocimientos del mundo y una conducta socialmente aceptable, nunca llegarán a «entrar en el mundo del libro». No se acostumbran a ver el mundo desde diferentes perspectivas, como la de un adulto o la de un anciano, de alguien de otra cultura, de otra región geográfica o de otra etapa histórica. Así corren el riesgo de adquirir menos flexibilidad mental… Sin conocimientos previos sobre el mundo y sin un vocabulario que vaya más allá de las 500 palabras e incluya las abstractas, los chicos no entienden ni el telediario. No sólo es que se sientan aislados del resto de la sociedad, es que lo están. A su vez, eso lleva a que todas las noticias les lleguen de sus compañeros en el barrio y eso da por resultado una tendencia a juzgar todo desde la perspectiva del círculo inmediato de amigos y conocidos. Así pueden crecer Estados dentro del Estado, sociedades paralelas. Se trata de un círculo vicioso. El desarrollo descrito es más frecuente entre los chicos que entre las chicas porque las familias tradicionalistas suelen acostumbrar a las chicas al trabajo y al autosacrificio. Además, para ellas, el estudio suele verse como una manera de escapar del control de la familia y, por eso, se esfuerzan más. Finalmente, en bastantes ambientes machistas, suele estar más aceptado que estudie una chica porque suelen considerar que estudiar es algo femenino.

Ahora en España mucha gente fía la solución de los problemas de la educación a que se alcance un pacto de partidos políticos pero, en su libro, describe a comunidades de chinos que lo hacen muy bien en China y también en California. ¿Hasta qué punto el desempeño académico depende de los valores familiares más que del sistema educativo?

La cultura de la casa es muy importante y más importante que el nivel socioeconómico. El ejemplo de los chinos en Occidente no cuaja con la visión de algunos partidos políticos de que todo depende del nivel económico y no del respeto por la educación y de la voluntad de los alumnos y de sus padres.

Si alabamos la educación de los niños chinos en sus familias, los hay que enseguida traen a colación la felicidad y vienen a decir que esos niños no lo son. ¿Hay pruebas de que así sea? ¿Qué deberían aprender en algunas casas occidentales de esa cultura de los padres chinos?

No hay ningún indicio de infelicidad generalizada entre los alumnos de alto rendimiento escolar. Si antes en las escuelas occidentales existía una cultura del esfuerzo sin que nadie dijera que los alumnos exitosos eran infelices, ¿por qué ahora lo dicen?

La empatía es una palabra que se ha puesto de moda en la educación pero a veces da la sensación de que, cuando se conocen las circunstancias específicas de cada alumno, se puede caer en la tentación de sentir pena y exigirle menos de lo que puede hacer. ¿Es así?

Hay unas corrientes educativas que enfatizan la empatía y proponen una educación «del cuidado», terapéutica. Es una corriente fuerte en la educación preescolar y en la educación primaria. Esta empatía puede ser negativa si no se combina con un enfoque en el aprendizaje porque, aunque protege al joven al comienzo, podría dejarle sin conocimientos para la vida adulta.

Suecia es modelo en la introducción del cheque escolar, con el que los padres eligen el colegio que quieren para sus hijos, ya sea privado o público. Y los resultados se ven con cierto escepticismo. ¿Cómo cree usted que ha funcionado?

El cheque escolar fue introducido en una situación muy especial, y por eso lo que ha pasado en Suecia no se puede tomar como un ejemplo general de cómo funciona este sistema. El cheque escolar significa, en el caso sueco, que toda la educación se paga con el dinero de los contribuyentes pero la gestión de una escuela puede ser privada. Sin embargo, los currículos, la ley escolar y las reglas administrativas son iguales para todas las escuelas. Cuando se introdujo al comienzo de los años 90 había un gran descontento con la educación pública, y lo que sucedió fue que se crearon cadenas de escuelas gestionadas por empresas más que por profesores idealistas. Estas escuelas atraen cada vez a más alumnos porque, desde entonces, la situación general de las escuelas públicas ha seguido empeorando debido a varios factores. La situación no se ha arreglado y una de las causas es que la energía del sistema escolar se dirige hacia la integración de los nuevos grupos de refugiados asiáticos y africanos.

La politización

Según explica usted, la educación, al menos en algunos sitios, se ha mezclado con la ideología, sobre todo de izquierdas, como cuando se pone en cuestión el papel de Occidente en el mundo. ¿Cree que también ha desempeñado un papel el nacionalismo como ideología en Cataluña, región que usted conoce bien?

Sí, obviamente. La educación ha sido el instrumento principal para el nacionalismo.

En ambientes tecnológicos ahora no se ve machista poner a las niñas en clases diferenciadas, sólo para ellas, para aprender a programar, por ejemplo. ¿Cree que en España el debate de la educación diferenciada está contaminado por prejuicios de la dictadura y de la Iglesia?

Creo que el tema se ha politizado en España y que se ve conectado con la Iglesia católica. No se toma en consideración lo propiamente educativo.

Si el Estado de bienestar ha conseguido un nivel mínimo en el que no se incita a los más perjudicados a luchar por la excelencia académica, ¿eso cómo se arregla? ¿Cómo se puede motivar a los de más abajo a ser excelentes y, así, en cierta manera, acortar la brecha de la desigualdad?

Haría falta más tiempo para contestar a la pregunta pero, en resumen, sería preciso hacer lo contrario de lo que se hace ahora. No tener promoción automática, ofrecer itinerarios interesantes para los alumnos que se esfuercen, y atraer a excelentes profesores en todos los niveles, y sería muy importante especialmente en la educación primaria.

Ahora parece que andamos todos un poco obsesionados con Finlandia como modelo educativo, pero, si tuviéramos que imitar lo mejor de un país, ¿con qué modelo se quedaba usted?

Mejor que mirar hacia cierto país es asegurarse de que funcionen cuatro factores: los alumnos deben aceptar que estudiar significa esforzarse, los profesores deben estar cuidadosamente seleccionados y tener una formación sólida, los padres deben apoyar la educación escolar de sus hijos y tener confianza en los profesores y el Estado debe establecer currículos y tipos de exámenes adecuados para asegurar la calidad del sistema educativo.

LA CLASE TRABAJADORA GANÓ LAS ELECCIONES

http://katehon.com/es/article/la-clase-trabajadora-gano-las-elecciones

10.11.2016

Estados Unidos

Paul Craig Roberts

Las elecciones presidenciales de Estados Unidos son históricas, porque el pueblo estadounidense fue capaz de derrotar a los oligarcas. Hillary Clinton, agente de la oligarquía, fue derrotada a pesar de la cruel campaña mediática contra Donald Trump. Esto demuestra que los medios y los establishmentde los partidos políticos ya no tienen credibilidad entre el pueblo estadounidense.

Queda por ver si Trump puede seleccionar y nombrar un gobierno que le sirva a él y a sus objetivos para restaurar los empleos estadounidenses y establecer relaciones amistosas y respetuosas con Rusia, China, Siria e Irán.

También queda por ver cómo responderá la oligarquía a la victoria de Trump. Wall Street y la Reserva Federal pueden causar una crisis económica para poner a Trump a la defensiva, y pueden usar la crisis para obligar a Trump a nombrar a uno de los suyos como Secretario del Tesoro. Los agentes rebeldes en la CIA y el Pentágono pueden causar un ataque de bandera falsa que interrumpa las relaciones amistosas con Rusia. Trump podría cometer un error y conservar a los neoconservadores en su gobierno.

Con Trump hay al menos esperanza. A menos que Trump se vea obstaculizado por el mal juicio en sus nombramientos y por los obstáculos puestos en su camino, debemos esperar el fin del conflicto orquestado de Washington con Rusia, la eliminación de los misiles estadounidenses en la frontera de Rusia con Polonia y Rumania, Ucrania, y el final de los esfuerzos de Washington para derrocar al gobierno sirio. Sin embargo, logros como estos implican la derrota de la oligarquía de los Estados Unidos. Aunque Trump derrotó a Hillary, la oligarquía todavía existe y sigue siendo poderosa.

Trump dijo que ya no ve el sentido a la OTAN 25 años después del colapso soviético. Si se atiene a su punto de vista, esto significa un gran cambio político en los vasallos de Washington de la UE. La hostilidad hacia Rusia de los actuales funcionarios de la UE y de la OTAN tendría que cesar. La canciller alemana Merkel tendría que cambiar su posición o ser reemplazada. El Secretario General de la OTAN, Jens Stoltenberg, tendría que ser despedido.

No sabemos a quién seleccionará Trump para servir en su gobierno. Es probable que Trump no esté familiarizado con las distintas posibilidades y sus posiciones sobre los asuntos. Realmente depende de quién esté aconsejando a Trump y de qué consejo le den. Una vez que veamos su gobierno, sabremos si podemos tener esperanzas acerca de los cambios que ahora tienen una oportunidad.

Si la oligarquía es incapaz de controlar a Trump y realmente tiene éxito en contener al poder y el presupuesto del complejo militar/de seguridad, y en mantener al sector financiero políticamente responsable, Trump podría ser asesinado.

Trump dijo que pondrá a Hillary en prisión. Primero debería llevarla a juicio por traición y crímenes de guerra junto con todos los neoconservadores. Eso despejaría el camino para la paz con las otras dos grandes potencias nucleares sobre las que los neoconservadores buscan la hegemonía. Aunque los neoconservadores todavía tendrían contactos en el estado profundo oculto, haría difícil para las alimañas el organizar operaciones de bandera falsa o un asesinato. Los elementos rebeldes en el complejo militar/de seguridad todavía podrían provocar un asesinato, pero sin los neoconservadores en el gobierno sería más difícil disimularlo.

Trump tiene más entendimiento y perspicacia de lo que sus oponentes perciben. Para que un hombre como Trump arriesgara a ganarse tantos enemigos poderosos y arriesgara su riqueza y su reputación, debía saber que la insatisfacción del pueblo con el establishment gobernante significaba que podría ser elegido presidente.

No sabremos qué esperar hasta que veamos quiénes son los secretarios y los subsecretarios. Si es la gente habitual, sabremos que Trump ha sido capturado.

Un feliz resultado duradero de las elecciones es el completo descrédito de los medios de comunicación estadounidenses. Los medios de comunicación predijeron una victoria fácil de Hillary e incluso el control del Partido Demócrata del Senado de los EE.UU. Aún más importante para la pérdida de influencia y credibilidad de los medios de comunicación, a pesar del cruel ataque de los medios de comunicación contra Trump durante las primarias presidenciales y la campaña presidencial, es que los medios no tuvieron ningún efecto fuera de las costas noreste y occidental. El resto del país ignoró a los medios de comunicación.

No pensé que la oligarquía permitiría a Trump ganar. Sin embargo, parece que los oligarcas fueron engañados por su propia propaganda mediática. Asegurados de que Hillary era la ganadora segura, no estaban preparados para poner en práctica los planes para robar las elecciones.

Hillary ha caído, pero los oligarcas no. Si se aconseja a Trump que sea conciliador, que tienda su mano y que adopte al establishment en su gobierno, el pueblo estadounidense volverá a ser decepcionado. En un país cuyas instituciones han sido tan completamente corrompidas por la oligarquía, es difícil lograr un cambio real sin derramamiento de sangre.

‘Esos idiotas peligrosos’: los medios progresistas, Trump y los estadounidenses de clase obrera

http://www.eldiario.es/theguardian/idiotas-peligrosos-progresistas-comprender-estadounidenses_0_571843050.html

Los partidarios de Trump no son la caricatura que presentan los periodistas. Sarah Smarsh, una periodista de origen humilde de Kansas, critica los estereotipos y el clasismo que se cuela en las redacciones

«Los medios han presentado a los blancos de clase trabajadora como un todo y han creado un imaginario caduco y traicionero que resulta muy conveniente para el capitalismo. Según este mensaje, los pobres son unos idiotas peligrosos»

Votantes de Trump en uno de sus mítines EFE

MÁS INFO

• ¿Por qué millones de trabajadores norteamericanos apoyan a Trump?
• ETIQUETAS: The Guardian,América, Estados Unidos,Donald Trump

En marzo mi abuela Betty, una anciana de 71 años, hizo tres horas de cola para poder votar a Bernie Sanders en el caucus del Partido Demócrata en el estado de Kansas. Era la primera vez que votaba en unas primarias y aunque fue un suplicio, en ningún momento se planteó regresar a casa sin haber votado. Betty, una mujer blanca que no terminó sus estudios de secundaria, que tuvo a su primer hijo a los dieciséis años y vivió en la más absoluta pobreza la mayor parte de su vida, quería votar.

Esperó su turno a pesar de sus debilitadas rodillas; las mismas que en el pasado la mantuvieron de pie durante horas en una fábrica. Esperó su turno a pesar del enfisema pulmonar provocado por el tabaquismo y de la dentadura postiza que ha lucido desde que era una veinteañera, dos señales claras de la clase social a la que pertenecemos. En la década de los sesenta, antes de la sentencia Roe contra Wade, la mujer que esperó su turno pagó a un desconocido para que le introdujera un gancho de alambre en el útero tras descubrir que estaba embarazada de un hombre del que huyó después de que le rompiera la mandíbula.

Durante muchos años, Betty trabajó como funcionaria de libertad condicional para el sistema judicial de Wichita, en Kansas. Su trabajo consistía en hacer un seguimiento de violadores y de asesinos. Por eso, está curada de espantos. Sin embargo, no ha dudado en afirmar que el candidato republicano Donald Trump es un sociópata “con la boca llena de mierda”.

Nadie detesta a Trump más que ella. El candidato dijo que debe castigarse a las mujeres que aborten y ha dicho cosas horribles de colectivos que ella conoce desde su infancia y con los que ha trabajado codo a codo. Su estilo pomposo e indecente ofende su sensibilidad humilde y del medio oeste americano.

La clase trabajadora, integrada por personas como Betty, se ha convertido en la obsesión de todos aquellos que cuando comentan estas elecciones presidenciales hablan de “clases”: ¿Quién está detrás de esta bestia feroz y por qué apoya a Trump?

Los votantes de Trump no son tan pobres

Las cifras cuantitativas ponen en duda, o niegan de plano, la tan regurgitada teoría de que el nivel de educación o de ingresos permite predecir el apoyo a Trump, o la afirmación de que la clase trabajadora blanca lo apoya desproporcionadamente.

El mes pasado, el resultado de una encuesta elaborada por Gallup sobre una muestra de 87.000 personas dejó entrever que los partidarios de Trump no tienen más problemas económicos o derivados de la inmigración que aquellos que se oponen al candidato republicano.

Según este estudio, sus seguidores no tienen ingresos más bajos o una tasa de desempleo más alta que otros estadounidenses. La información relativa a los ingresos se pierde elementos importantes: aquellos con ingresos altos también pueden tener problemas de salud o ser propensos a empeorar económicamente.

Sin embargo, la mayoría de encuestados no se aferraban a trabajos que podrían perder. Uno de los analistas de Gallup explicó que, sorprendentemente, “parece no haber ningún tipo de relación entre sufrir la amenaza de la competencia comercial con otro país y apoyar políticas nacionalistas en Estados Unidos”.

El típico grandullón que amenaza a personas todavía más débiles que él y que amenaza a las personas de color para que huyan del pueblo, insulta a las mujeres y utiliza pistolas de aire comprimido para disparar contra gatos. Así sería Trump si hubiera nacido donde yo nací.

A principios de año, los sondeos que se llevaron a cabo antes de las primarias mostraron que aquellos que votaron a Trump tienen un mayor poder adquisitivo que el resto de estadounidenses, con unos ingresos familiares de 72.000 dólares, lo cual supera los ingresos de los que votaron a Hillary Clinton o a Bernie Sanders. El 44% tiene un título universitario; en comparación con la media nacional, que es del 29% para el conjunto de la población, o del 33% en el caso de la población blanca.

En enero, el politólogo Matthew MacWilliams indicó que uno de los factores que permite predecir el apoyo a Trump es una cierta tendencia al autoritarismo , mientras que los ingresos, la educación, el género, la edad o la raza no son factores determinantes.

Sin embargo, todos estos hechos objetivos no han servido para que los expertos y los periodistas dejen de repetir hasta la saciedad que la clase obrera blanca ha decidido apoyar a un demagogo que se distingue por su grandilocuente verborrea.

Para explicar correctamente por qué parte de la ciudadanía se siente atraída por Trump, una cobertura mediática equilibrada debería incluir más reportajes sobre el racismo y la misoginia en los barrios acomodados donde viven algunos votantes de Trump. O, en el supuesto de que se esté valorando la amargura de la clase trabajadora causada por la situación económica, también deberían publicarse reportajes sobre legisladores demócratas que en las últimas décadas han decidido destruir la red de bienestar, se subieron al carro de Wall Street y se olvidaron de los trabajadores estadounidenses cuando negociaron acuerdos comerciales internacionales.

Sin embargo, para los medios de comunicación nacionales, integrados, en su mayoría, por progresistas de clase alta o de clase media, eso supondría tener que mostrar los rostros de sus semejantes.

Si bien es ciert o que los rostros que los periodistas muestran en televisión –rostros enfurecidos que hacen comentarios sexistas cerca de una bandera de la Confederación– se merecen algún tipo de cobertura mediática, no son un reflejo de las comunidades que yo conozco tan bien. El hecho de que los medios de comunicación hayan ignorado comunidades como la mía ha creado una falta de comprensión tan grave que con un primer vistazo a un blanco con problemas económicos parece servir para describir a la totalidad.

El ejemplo antropológico de JD Vance

Un vistazo a la actualidad nos lleva hasta JD Vance, autor de una autobiografía que ha sido éxito de ventas, Hillbilly Elegy (Elegía del palurdo) . Es la historia de un abogado de éxito que creció en una pequeña ciudad siderúrgica de Ohio y cuya familia, a pesar de ser de clase media, lidiaba con la precariedad. El libro nos habla del caos que suele perseguir a una familia que ha quedado atrapada en un ciclo de pobreza durante generaciones.

Vance se autodefine como conservador y afirma que no votará a Trump. Sin embargo, intenta comprender por qué muchas personas de clase trabajadora sí lo harán. Tiene que ver con una ansiedad cultural que surge cuando muchos amigos consumen opiáceos y mueren por sobredosis y la casta política ya te ha dejado claro que no te ayudará. Si bien su experiencia es extrapolable a la de otras personas de zonas concretas, los periodistas de la Costa Este han convertido a Vance en portavoz de toda la clase obrera blanca.

Los entrevistadores y los críticos literarios parecen sentirse aliviados por el hecho de haber encontrado a alguien que tiene unas opiniones que confirman las suyas. The Run-Up, el podcast de las elecciones del The New York Times, afirmó que la autobiografía de Vance también es un estudio de antropología cultural de la clase obrera blanca que ha apoyado la candidatura de Trump (al tuitear la crítica del libro, el The New York Times ironizó con la pregunta: ¿Quieren saber más sobre las personas que le han dado alas a Donald Trump?”.

Seguir

J.D. Vance

✔@JDVance1

WSJ: a beautiful memoir but it is equally a work of cultural criticism about white working-class America (buy here: http://tinyurl.com/gwyw52r )

19:17 – 31 jul 2016

Hillbilly Elegy: A Memoir of a Family and Culture in Crisis

#1 NEW YORK TIMES BESTSELLER «You will not read a more important book about America this year.»—The Economist «A riveting book.»—The Wall Street Journal»Essential reading.»—David Brooks, New York…

amazon.com

• 136136 Retweets

• 329329 me gusta

Si bien los orígenes de Vance se remontan a la industria minera de Kentucky, la mayoría de los blancos con dificultades económicas no son hombres conservadores y protestantes de los Apalaches. A veces parece ser el único elemento del imaginario colectivo: un tipo escondido en una chabola situada en una montaña remota, como un fantasma polvoriento, como si la pobreza de los blancos no estuviera delante de nuestras narices, pasando nuestras tarjetas de crédito en una tienda de rebajas en Denver o pidiendo limosna en una calle de Los Ángeles.

Los estereotipos simplones suelen penetrar allí donde el periodismo no consigue llegar. La última vez que la clase a la que pertenezco por nacimiento recibió una atención mediática de estas proporciones fue 20 años atrás. No salió en los informativos sino en una serie de televisión,Roseanne . El guión de esta serie resulta más riguroso y certero que las reflexiones de los comentaristas de las cadenas de televisión de Nueva York.

Las imágenes de personas blancas de clase trabajadora y progresistas, entre las que se incluyen mujeres como Betty, no son mostradas por unos medios de comunicación obsesionados por las audiencias y que cubren estas elecciones como si se tratara de una carrera de caballos.

Los pobres, idiotas peligrosos

Este paradigma de los medios de comunicación ha alimentado la leyenda de un Estados Unidos polarizado, el azul demócrata contra el rojo republicano, en el que el 42% de los habitantes de Kansas que votaron a Barack Obama en 2008 han quedado silenciados.

En estas primarias, el número de habitantes de Kansas que participó en el caucus demócrata superó el de aquellos que votaron en el caucus de Donald Trump. Se trata de una información relevante y lo cierto es que ningún periódico nacional la ha mencionado, tal vez porque no pudo entender que en esa zona que observa desde la lejanía viven millones de estadounidenses más progresistas que los que se pueden encontrar en los bastiones de Clinton.

En lugar de dar este tipo de información, los medios han presentado a los blancos de clase trabajadora como un todo y han creado un imaginario caduco y traicionero que resulta muy conveniente para el capitalismo. Según este mensaje, los pobres son unos idiotas peligrosos.

La superioridad moral que siente la clase adinerada de Estados Unidos ha dado alas a esta leyenda urbana relativa a los blancos de clase trabajadora y que los presenta como los culpables del auge de Donald Trump y que presupone que aquellos que lo apoyan por los peores motivos representan al conjunto de partidarios.

Esta noción se repite en todos los análisis sobre estas elecciones, como también la creencia de que los blancos pobres no solo tienen problemas económicos sino también de personalidad.

En un artículo sobre estas elecciones publicado por el National Review en marzo, Kevin Williamson escribió un análisis sobre los votantes blancos con pocos recursos. En las últimas décadas este colectivo ha visto como su tasa de mortalidad ha aumentado considerablemente. Su artículo se hace eco de una creencia compartida por conservadores y progresistas cuando indica que estas comunidades, devastadas por la oxicodona, “se merecen morir”.

“ Los blancos de clase baja están instalados en una subcultura tóxica y egoísta cuyas consecuencias son la miseria y el consumo de heroína”, afirma. “Los discursos de Donald Trump hacen que se sientan bien. Como la oxicodona”.

Para confirmar que muchos periodistas no comprenden a este colectivo y que no se trata de un fenómeno limitado a los conservadores más provocadores, solo hace falta leer una serie de reportajes publicada por el The Washington Post que analiza por qué la tasa de mortalidad de las mujeres blancas que viven en zonas rurales se ha disparado. Se centra en sus hábitos como fumadoras y describe con todo detalle “sus caras demacradas” y el proceso de embalsamamiento de sus cuerpos. Es difícil imaginar un reportaje que analizara a mujeres blancas de clase alta tras su fallecimiento. La indignación de sus familiares y amigos con la educación, el tiempo y la voluntad de escribir cartas a los directores de los periódicos sería descomunal.

Dignidad y tristeza en la clase trabajadora

Un sentimiento que me parece incluso más ridículo que el desprecio y la humillación es su “primo pobre”: la piedad.

En una columna de opinión que publicó recientemente David Brooks en el The New York Times, titulada Dignity and Sadness in the Working Class (Dignidad y tristeza de la clase trabajadora), el periodista nos habla de un obrero del sector de la metalurgia que vive en el estado de Kentucky y que ha perdido su trabajo. En su último día en la fábrica, el hombre se dirige hacia la salida mientras es vitoreado por sus compañeros, una escena que a mí me parece triunfal pero que a Brooks le parece lamentable. El periodista señala que el hombre trabajó muy duro por una miseria y que era muy capaz pero su trabajo no se valoraba. Según él “irradiaba la tristeza residual de un corazón solitario”.

Me resulta difícil imaginar un desprecio mayor. Estos profesionales de la comunicación han ignorado los problemas de la clase trabajadora durante décadas y ahora suplican al país que tenga compasión. No necesitamos sus análisis y todavía menos sus lágrimas. Lo que necesitamos es que alguien explique nuestra situación; a ser posible un periodista que pueda entrar en una fábrica sin que una niebla de culpabilidad empañe sus gafas.

Uno de estos periodistas, Alexander Zaitchik, viajó durante varios meses a lo largo y ancho de seis estados del país para conocer de primera mano a blancos de clase trabajadora que apoyan a Trump. Quería que el libro que publicará –The Gilded Rage (La Furia Dorada, en un juego de palabras con ‘the Gilded Age’, la edad dorada)– reflejase la complejidad de las historias humanas que son ignoradas por la cobertura mediática diaria. Zaitchik explica que el proyecto nació como consecuencia de los duros comentarios realizados por personas que viven en un huso horario completamente distinto al de estas comunidades y que tienen unos niveles de ingresos completamente distintos.

Zaitchik describe de forma inteligente su encuentro con la clase media trabajadora, integrada en su mayoría por blancos que han trabajado duro y que han sufrido graves pérdidas, tanto durante la crisis financiera de 2008 como por los cierres de fábricas y despidos de los últimos años. Descubrió que el apoyo a Trump se debe en gran medida a motivos económicos, de principio a fin. Pudo constatar la ira de estas personas y descubrió que están indignados con los de arriba, no con los de abajo. Están enfadados con todos aquellos que negociaron acuerdos comerciales globales, no con las minorías.

Al mismo tiempo, es cierto que en estas comunidades se dan actitudes racistas y nacionalistas, como también se dan entre los demócratas y las personas con una situación más privilegiada.

Una encuesta realizada la pasada primavera por Reuters refleja que un tercio de los demócratas encuestados apoyarían que temporalmente se prohibiera la entrada de musulmanes en Estados Unidos. En otra encuesta, en este caso de YouGov, el 45% de los demócratas encuestados reconocieron que tienen una mala opinión del Islam, sin que se apreciaran diferencias entre los encuestados con distinto nivel de ingresos. Muchos de los que no votarán a Trump no son un dechado de virtudes mientras que los que sí lo harán se convierten en un blanco de ataque fácil y se les considera la plaga moral del país.

El clasismo y “una panda de abominables”

Cuando recientemente Hillary Clinton afirmó que la mitad de los que apoyan a Trump son “una panda de abominables”, Zaitchik le comentó a otro periodista que esta expresión se podía interpretar como otra forma de decir “otro cubo de basura blanca”. Clinton no tardó en disculparse por este comentario. Sin embargo, generalizar de este modo en un acto que se celebró en la parte baja de Manhattan, en el que se recaudaron 6 millones de dólares, con asistentes que llegaron a pagar entradas de hasta 50.000 dólares, me evocó algunas escenas de la comedia televisiva Veep; una sátira política en la que un poderoso político de Washington habla con desdén sobre “la gente corriente”.

Cuando hablamos, Zaitchik mencionó al presentador de la cadena HBO Bill Maher, “cuyas opiniones sobre los que votan a Trump se fundamentan en la eugenesia, ya que considera que tiene defectos congénitos. Sería imposible hablar de otro grupo de personas en estos términos y no ser despedido”.

Tal vez Maher es un ejemplo extremo de petulancia clasista. En el verano de 1998, cuando tenía 17 años y me acababa de graduar del instituto, trabajé en un elevador de grano durante la siega del trigo. Un elevador que estaba situado a unos 80 kilómetros, en Haysville, Kansas, explotó (el polvo del trigo es muy inflamable) y siete trabajadores murieron en la explosión. El accidente sacudió a mi comunidad, a mi familia y a mí y nos sirvió de recordatorio de todos los peligros que corremos cuando trabajamos como agricultores.

http://widget.smartycenter.com/webservice/embed/9355/1924767/630/354/0/0/0/100/1/1

Como todos los demás, seguí haciendo mi trabajo. Tras una larga jornada transportando sacos pesados y cargando camiones que transportan trigo, solía ver el programa de televisión Politically Incorrect, un programa de ABC que por aquel entonces presentaba Maher. En un contexto en el que todavía se estaba buscando el cuerpo de uno de los trabajadores muertos en la explosión de Haysville, Maher bromeó acerca de que la gente debería tener mucho cuidado con las rebanadas de pan.

Creo que por primera vez tomé conciencia del hecho de que a lo largo de mi vida me iba a identificar políticamente con aquellos que insultan mis orígenes.

Este tipo de bromas están tan generalizadas que los más privilegiados económicamente no suelen darse cuenta. Los que escriben, debaten y publican periódicos, libros y revistas con la mejor de las intenciones suelen ofender desde la ignorancia.

Por ejemplo, fueron muchos los que me recomendaron el éxito de ventas White Trash (Basura blanca), de Nancy Isenberg, sin percatarse de que el título me ofende a mí y a las personas que quiero. El alivio que sentía por el hecho de que alguien hubiera escrito sobre un pasado que compartimos se esfumaba cada vez que lo veía en mi biblioteca, hasta el punto que al final opté por quitarle la portada. Sorprendentemente, los ejemplares promocionales del libro reflejan el tipo de nociones elitistas que Isenberg quiere denunciar: “Este libro parte de nuestros mitos reconfortantes sobre la igualdad y deja al descubierto el legado fundamental de la omnipresente y embarazosa, aunque a veces entretenida, basura blanca pobre”.

El libro, en cambio, está escrito con más tacto y expone hechos que deberían servir para terminar con los prejuicios a los que se refiere el título. Aunque lo cierto es que ni siquiera Isenberg consigue librarse del marco clasista.

Cuando a principios de año la presentadora de On the Media, Brooke Gladstone, le pidió a Isenberg que hablara de prejuicios que presentan a los blancos pobres como personas intolerantes, la autora habló del problema: “Tienen ciertas actitudes que sin duda son racistas y no puedes esconderlas y hacer como que no existen. Forma parte de su mentalidad”.

¿Solo los ignorantes son racistas?

Todas estas generalizaciones sobre los grupos más vulnerables nos permiten ver que los debates en torno a las clases en un país que es relativamente joven y que creía que no tenía castas son extremadamente simplones.

“ El problema es que muchos intentan presentar a los blancos pobres como los únicos racistas del país”, le explicó Isenberg a Gladstone: “Como si fueran más racistas que el resto”.

La raíz de este problema reside en la creencia de que la clase alta tiene una moral más elevada. Como escribió la periodista Lorraine Berry en un artículo publicado el mes pasado, se ha consolidado la noción de que solo los ignorantes son racistas. Según este discurso, el racismo desaparece con la educación. Soy la primera persona de mi familia con un título universitario y les puedo asegurar que ningún miembro de mi familia necesitó pasar por una universidad para aprender qué es tener un mínimo de decencia humana.

Berry señala que los republicanos formados en las universidades de élite están detrás de esta creencia. De hecho, no fueron los blancos pobres, ni siquiera los blancos republicanos, los que promulgaron leyes para mantener la segregación racial o los que durante décadas observaban cómo las banderas confederadas ondeaban en los capitolios estatales. No fueron los blancos pobres los que convirtieron a los negros en criminales con leyes que prohibían la marihuana y la guerra contra las drogas. Tampoco fueron los blancos pobres los que se inventaron el fantasma de la “reina de la beneficencia” para referirse a los afroamericanos.

Con ello no quiero minimizar la importancia del racismo en los estratos más bajos de la sociedad pero sí recordar que estos comportamientos horribles también están presentes en las clases más altas de distinta forma y con mucha más fuerza.

Los periodistas y los comentaristas también deberían señalar con el dedo a otro tipo de blancos: conservadores sociales que donan dinero a la campaña de Trump pero que son demasiado civilizados como para ir a un mitin y chillar para expresar sus opiniones.

Según el discurso de la campaña de Trump y la información disponible, lo votarán personas a las que les va bastante bien pero que se consideran víctimas del sistema.

Los medios no parecen entender que gran parte de la clase trabajadora blanca preferiría cerrar filas con cualquier otro sentimiento que no sea el de victimismo. En la actualidad, fichan cuando entran y salen de su trabajo, guardan los cupones de descuentos de los supermercados, educan a sus hijos en el respeto e intentan esquivar la cobertura mediática.

Brecha entre realidad y política

Barack Obama, un hombre negro formado a partir de la experiencia negra, suele citar a sus descendientes por parte de madre; gente blanca de clase trabajadora: “Muchas de mis influencias proceden de mis abuelos maternos, que crecieron en el interior de Kansas”, indicó este mes a propósito de un encuentro celebrado en la Casa Blanca sobre cuestiones rurales.

El año pasado, en una conversación con la autora Marilynne Robinson, del The New York Review of Books, Obama lamentó todos estos conceptos erróneos y tan comunes sobre las pequeñas localidades del interior de Estados Unidos, por las que él siente admiración. “Hay una brecha enorme entre la realidad de las vidas diarias de estas personas y cómo hablamos de la realidad de Estados Unidos y de la vida política”. Señaló que uno de los elementos que contribuyen a ampliar esta brecha son “los filtros entre las personas corrientes” que hacen lo que pueden por sobrevivir, así como los debates políticos demasiado complejos.

«Nos debería hacer reflexionar el hecho de que destacados comentaristas progresistas consideren que el término “populismo” tiene connotaciones negativas»

“ Me siento muy reconfortado cuando tengo la oportunidad de conocer a estas personas en su contexto”, explicó: “Por algún motivo, el filtro hace que en el ámbito político nacional sus realidades no se presenten de forma alentadora”.

Sin duda, una de estas descripciones desalentadoras, la caricatura del votante blanco que destila odio y que lleva vaqueros grasientos, responde a una realidad. En mi pueblo conocí a uno o dos; el típico grandullón que amenaza a personas todavía más débiles que él y que amenaza a las personas de color para que huyan del pueblo, insulta a las mujeres y utiliza pistolas de aire comprimido para disparar contra gatos. Así sería Trump si hubiera nacido donde yo nací.

La fascinación de los medios de comunicación hacia el votante de Trump alimenta la teoría, tan de moda, de que detrás de su apoyo se esconde la intolerancia. Es cierto que los problemas económicos de la clase trabajadora blanca son un punto más para Trump, como también lo es la falta de dinero de las personas de color, que al mismo tiempo son el blanco de ataque de sus comentarios racistas y xenófobos y que por este motivo le han dado la espalda. Sin embargo, uno creería que a los progresistas blancos que pertenecen a la élite y que a lo largo de esta campaña han transmitido una imagen de grandeza ética les costaría más pensar en términos globales sobre relaciones comerciales e inmigración si hubieran tenido que cerrar su fábrica o su comunidad hubiera sido diezmada.

Analistas acomodados

Los analistas acomodados que se oponen a Trump suelen examinar los males sociales desde un determinado punto de vista; están convencidos de que sus tendencias políticas son un reflejo de sus valores y de su personalidad. Cabe suponer que muchos de ellos heredaron estas ideas, de la misma forma que muchos estadounidenses que crecieron en los estados republicanos heredaron las suyas. Si creciste en un ambiente progresista, no deberías estar tan orgulloso de ti mismo por votar en contra de Trump.

También está de más esta idea condescendiente de que los demócratas que en las últimas décadas no se han sentido representados por su partido y que se han unido al Partido Republicano “están votando en contra de sus intereses. Esta noción tiene un trasfondo antidemocrático, ya que parte de la premisa de que un gran número de estadounidenses carece de las condiciones mentales que se precisan para votar”.

Son muchos los que siguen apoyando a Trump a pesar de todo lo publicado sobre su trato a las mujeres, sus actitudes racistas y otras actitudes temerarias. Son capaces de decidir su voto y de tomar sus propias decisiones. Cuando intentemos discernir de quien estamos hablando, debemos ser conscientes de nuestros prejuicios de clase.

¿Periodista? No de clase obrera

Un artículo publicado recientemente en la edición impresa del The New York Times describía a un hombre de Kentucky así: “Mitch Hedges cultiva ganado y suelda herramientas que se utilizan en las minas de carbón. Cree que va a perder su trabajo en seis meses pero no apoya a Trump, al que considera un idiota”.

Celebré que, por una vez, se hablara de un hombre blanco de clase obrera que no vota a Trump. Me hizo reír la expresión “cultivar ganado” ya que uno puede cultivar la cosecha o criar animales. Para una periodista que durante su juventud hizo ambas cosas, es difícil tomarse en serio este reportaje de The New York Times.

La principal razón por la cual los medios de comunicación más importantes no parecen comprender las cuestiones de clase es precisamente que no hay diversidad socioeconómica en las redacciones.

Pocas personas que crecieron rodeadas de pobreza terminan trabajando en las redacciones o publicando libros. De hecho, son tan pocas que me pareció necesario dar un giro a mi carrera y especializarme en cuestiones de clase en un sector rico y privilegiado de la misma forma que los periodistas negros hablan de raza en un sector que está integrado mayoritariamente por blancos.

Con esto no quiero decir que uno debe pertenecer a un determinado grupo o lugar para hacerles justicia, como han demostrado los buenos periodistas de investigación y los comentaristas en el último siglo e incluso antes.

Escuchen la serie sobre pobreza que ha emitido la radio On the Media. El segundo episodio de esta serie incluye la siguiente reflexión de Gladstone: “Los pobres, en su conjunto, son un grupo tan poco homogéneo como cualquier otro”.

Sé que muchos periodistas son personas muy trabajadoras que quieren presentar la historia bajo el ángulo correcto y no me gusta criticar a los medios de comunicación. El clasismo de los presentadores de la televisión por cable es simplemente un reflejo del clasismo del sector más privilegiado de Estados Unidos. Lo vemos en todas partes, desde los tuits que presentan a los votantes de Trump como palurdos sin remedio hasta en el hecho de que el Partido Demócrata que no se tomó la molestia de crear una plataforma centrada en las medidas de reducción de la pobreza hasta un mes antes de las elecciones presidenciales.

Medios deliberadamente obtusos

La distancia económica que separa al periodista de los protagonistas de sus reportajes nunca ha sido tan peligrosa como en la actualidad, marcada por una histórica disparidad entre ricos y pobres. A menudo los reportajes se centran en el mercado de valores y no en las personas que nunca tuvieron acciones.

Durante décadas, los medios de comunicación de Estados Unidos han sido deliberadamente obtusos cuando han tenido que informar de las quejas de los ciudadanos de a pie. Este ha sido un factor que sin duda ha ayudado a crear el espacio de resentimiento que Trump ahora ocupa. Nos debería hacer reflexionar el hecho de que destacados comentaristas progresistas consideren que el término “populismo” tiene connotaciones negativas.

Estamos ante un periodismo que integra la plutocracia que debería criticar, que no ha sabido cumplir con su deber de guardián de la verdad y que ha perdido el respeto hacia todas aquellas personas que no dudan en llamar las cosas por su nombre.

Mi abuelo Arnie, que ya ha fallecido, era una de estas personas. Hombres parecidos a Trump pasaban con sus lujosos vehículos por nuestra granja, con la intención de hacer negocios. Mi abuelo sabía reconocer a los que eran unos embusteros y unos estafadores, los trataba con amabilidad y los mandaba a paseo. Si por algún motivo te despedías de alguno de ellos con un apretón de manos, mi abuelo se reía y te decía: “mejor que cuentes tus dedos”.

En un mundo en el que las “Bettys” y los “Arnies” prácticamente no tienen voz, los que tienen una plataforma desde la que lanzar sus opiniones deberían reflexionar antes de despotricar sobre ellos.

Tal vez quieras generalizar y crear un estereotipo para presentar a un grupo de personas y especular sobre sus ideas políticas o creerte superior a ellos, por ejemplo, los que hacían un tercer turno en una fábrica de Boeing mientras otros viajaban a México de vacaciones, los que limpiaban el suelo de un McDonalds mientras otros debatían en las redes sociales en torno al salario mínimo, los que tuvieron que vaciar sus casilleros cuando se cerró la fábrica de cerveza Pabst mientras otros bebían cervezas artesanales en bares de moda, los que regresaron de Oriente Medio dentro de un ataúd mientras otros escribían columnas de opinión sobre política exterior. Si este es el caso, deberías aceptar el hecho de que tal vez te pareces más a Trump de lo que te gustaría.

Donald Trump presenta un contrato a los ciudadanos americanos con medidas a cumplir en 100 días

http://www.mundiario.com/articulo/politica/donald-trump-presenta-contrato-ciudadanos-americanos-medidas-cumplir-100-dias/20161109182608071884.html

El flamante ganador de las elecciones presidenciales en Estados Unidos ya ha publicado lo que hará en los 100 primeros días de su mandato. Entre sus planes: anunciar la retirada de EE UU del Acuerdo de Asociación Transpacífico (TPP), bajar impuestos, derogar el Obamacare y construir el muro con México.

Donald Trump. / donaldjtrump.com

Donald Trump, contra todo pronóstico, encuesta o sondeo, ganó a la demócrata Hillary Clinton, la gran favorita de casi todos los medios de comunicación de todo el mundo. Pero los medios no votan, vota la gente y millones de estadounidenses tenían claro que 4 años más de un gobierno demócrata no resultaría satisfactorio para sus intereses. El tiempo dirá si acertaron o no colocando a Trump en el puesto más importante del planeta, el de presidente de los Estados Unidos de América.

Mientras ese tiempo llegue y demuestre algunas cosas, el republicano ya ha publicado en su web un plan de acción a cumplir en los 100 primeros días de su presidencia.

Lo primero que Trump señala son el establecimiento de 6 medidas para limpiar la corrupción en el país. Desde congelar la contratación de empleados federales para reducir la mano de obra (quedan exentos los militares, trabajadores de la seguridad pública y de la sanidad pública), a limitar a 5 años el trabajo de los funcionarios del Congreso y la Casa Blanca, prohibir de por vida que funcionarios que hayan ejercido en la Casa Blanca trabajen para gobiernos extranjeros o prohibir el trabajo de los grupos de presión extranjeros en las elecciones estadounidenses.

Después plantea 7 medidas para proteger el trabajo de los estadounidenses. Anuncia así su intención de renegociar NAFTA (El Tratado de Libre Comercio de América del Norte entre los gobiernos de Canadá, EEUU y México) o retirarse del Acuerdo bajo el artículo 2205.

Va a anunciar la retirada de EE UU del Acuerdo de Asociación Transpacífico (TPP).

Dirigirá la Secretaría de Comercio y Representante de Comercio para identificar todos los abusos comerciales extranjeros que afectan directamente a los trabajadores estadounidenses y pondrá fin a los mismos. Además cancelará los miles de millones de pagos que EE UU hace a la ONU para programas de cambio climático y usará el dinero para reestructurar las infraestructuras de agua y medio ambiente en América.

En cuanto a la seguridad y el estado de derecho constitucional, Trump plantea para sus primeros 100 días de gobierno 5 acciones entre las que se encuentran comenzar con el proceso de selección para reemplazar al fallecido Juez Scalia en la Corte Suprema de EE UU; cancelar todos los fondos federales destinados a las ciudades santuario (ciudades consideradas como amigables con los inmigrantes ilegales); comenzar a expulsar a los más de 2 millones de inmigrantes ilegales y criminales que Trump estima están en el país. Y cancelar las visas a países extranjeros que no los devuelvan. Además suspenderá la inmigración de regiones propensas al terrorismo.

Otras de las medidas que recoge en este plan de acción en 100 días son aliviar de impuestos a la clase media y poner en marcha un plan económico diseñado para hacer crecer la economía en 4% anual, creando además 25 millones de nuevos empleos. Reducir y simplificar los impuestos. Su plan fiscal reduce los tramos del impuesto sobre la renta a tres. Así, los matrimonios que ganan menos de 75.000 dólares anuales tendrían un impuesto del 12%, los que ganan menos de 225.000 tendrían uno del 25% y por encima de esa cantidad el porcentaje sería del 33% (para los que hacen su declaración de manera individual las cantidades serían la mitad de las indicadas).
Reducirá el impuesto de sociedades del 35 al 15%.

Establecerá tarifas para disuadir a las empresas de trasladarse a otros países. Aprovechará las asociaciones público-privadas e inversiones privadas mediante incentivos fiscales para estimular en 1 billón de dólares las inversión en infraestructura durante diez años.

En cuanto a aspectos educativos redireccionará el dinero destinado a la educación para dar a los padres el derecho de enviar a sus hijos a un colegio público, privado, religioso, etc. según su elección. Ampliará la educación en comunidades locales y hará más asequible la universidad.

En temas sanitarios derogará la ley sanitaria de Obama (Obamacare) y reemplazarla con el Health Savings Accounts: los ciudadanos usen cuentas bancarias de ahorro para gastos sanitarios con ventajas fiscales y condiciones flexibles que se pueden pasar a la descendencia. También sugiere que la gente pueda deducir sus pagos por las primas de su seguro médico.

Acelerará la aprobación de medicamentos que puedan salvar vida y permitir a los estadounidenses deducir servicios de cuidado de niños y ancianos de sus impuestos.

Por supuesto, no se olvida de la inmigración. Dará fin a la Ley de Inmigración Ilegal y se reafirma en el hecho de construir un  muro en la frontera con México que pagará el propio país latinoamericano en su totalidad.

Establecerá un pena de 2 años mínimos en una prisión federal por reingreso ilegal en los EE UU después de una deportación anterior y un mínimo obligatorio de 5 años en prisión para los que ingresen ilegalmente en el país con condenas por delitos mayores, menores o con dos o más deportaciones anteriores.

Mejorará las penas por exceso de tiempo de permanencia en el país; garantizar el empleo priorizando a los ciudadanos estadounidenses; disminuir la delincuencia, las drogas y la violencia creando un grupo de trabajo sobre el tema y un programa que entrene y ayude a la policía local.
Aumentará los recursos para las agencias federales y fiscales con el fin de desmantelar bandas criminales.

Restablecerá la Ley de Seguridad Nacional, reconstruirá el ejército y ampliará la inversión militar.

Las 7 propuestas de Donald Trump que los grandes medios censuraron… y que explican su victoria

Por Ignacio Ramonet

El éxito de Donald Trump ( como el ‘Brexit’ en el Reino Unido, o la victoria del ‘no’ en Colombia ) significa primero una nueva estrepitosa derrota de los grandes medios dominantes y de los institutos de sondeo y de las encuestas de opinión. Pero significa también que toda la arquitectura mundial, establecida al final de la Segunda Guerra Mundial, se ve ahora trastocada y se derrumba. Los naipes de la geopolítica se van a barajar de nuevo. Otra partida empieza. Entramos en una era nueva cuyo rasgo determinante es lo ‘desconocido’. Ahora todo puede ocurrir.

¿Cómo consiguió Trump invertir una tendencia que lo daba perdedor y lograr imponerse en la recta final de la campaña ? Este personaje atípico, con sus propuestas grotescas y sus ideas sensacionalistas, ya había desbaratado hasta ahora todos los pronósticos. Frente a pesos pesados como Jeb Bush, Marco Rubio o Ted Cruz, que contaban además con el resuelto apoyo del establishment republicano, muy pocos lo veían imponerse en las primarias del Partido Republicano, y sin embargo carbonizó a sus adversarios, reduciéndolos a cenizas.

Hay que entender que desde la crisis financiera de 2008 (de la que aún no hemos salido) ya nada es igual en ninguna parte. Los ciudadanos están profundamente desencantados. La propia democracia, como modelo, ha perdido credibilidad. Los sistemas políticos han sido sacudidos hasta las raíces. En Europa, por ejemplo, se han multiplicado los terremotos electorales (entre ellos, el Brexit). Los grandes partidos tradicionales están en crisis. Y en todas partes percibimos subidas de formaciones de extrema derecha (en Francia, en Austria y en los países nórdicos) o de partidos antisistema y anticorrupción (Italia, España). El paisaje político aparece radicalmente transformado.

Ese fenómeno ha llegado a Estados Unidos, un país que ya conoció, en 2010, una ola populista devastadora, encarnada entonces por el Tea Party. La irrupción del multimillonario Donald Trump en la Casa Blanca prolonga aquello y constituye una revolución electoral que ningún analista supo prever. Aunque pervive, en apariencias, la vieja bicefalia entre demócratas y republicanos, la victoria de un candidato tan heterodoxo como Trump constituye un verdadero seísmo. Su estilo directo, populachero, y su mensaje maniqueo y reduccionista, apelando a los bajos instintos de ciertos sectores de la sociedad, muy distinto del tono habitual de los políticos estadounidenses, le ha conferido un carácter de autenticidad a ojos del sector más decepcionado del electorado de la derecha. Para muchos electores irritados por lo « políticamente correcto », que creen que ya no se puede decir lo que se piensa so pena de ser acusado de racista, la « palabra libre » de Trump sobre los latinos, los inmigrantes o los musulmanes es percibida como un auténtico desahogo.

A ese respecto, el candidato republicano ha sabido interpretar lo que podríamos llamar la « rebelión de las bases ». Mejor que nadie, percibió la fractura cada vez más amplia entre las élites políticas, económicas, intelectuales y mediáticas, por una parte, y la base del electorado conservador, por la otra. Su discurso violentamente anti-Washington y anti-Wall Street sedujo, en particular, a los electores blancos, poco cultos, y empobrecidos por los efectos de la globalización económica.

Hay que precisar que el mensaje de Trump no es semejante al de un partido neofascista europeo. No es un ultraderechista convencional. Él mismo se define como un «conservador con sentido común» y su posición, en el abanico de la política, se situaría más exactamente a la derecha de la derecha. Empresario multimillonario y estrella archipopular de la telerealidad, Trump no es un antisistema, ni obviamente un revolucionario. No censura el modelo político en sí, sino a los políticos que lo han estado piloteando. Su discurso es emocional y espontáneo. Apela a los instintos, a las tripas, no a lo cerebral, ni a la razón. Habla para esa parte del pueblo estadounidense entre la cual ha empezado a cundir el desánimo y el descontento. Se dirige a la gente que está cansada de la vieja política, de la « casta ». Y promete inyectar honestidad en el sistema ; renovar nombres, rostros y actitudes.

Los medios han dado gran difusión a algunas de sus declaraciones y propuestas más odiosas, patafísicas o ubuescas. Recordemos, por ejemplo, su afirmación de que todos los inmigrantes ilegales mexicanos son “corruptos, delincuentes y violadores”. O su proyecto de expulsar a los 11 millones de inmigrantes ilegales latinos a quienes quiere meter en autobuses y expulsar del país, mandándoles a México. O su propuesta, inspirada en « Juego de Tronos », de construir un muro fronterizo de 3.145 kilómetros a lo largo de valles, montañas y desiertos, para impedir la entrada de inmigrantes latinoamericanos y cuyo presupuesto de 21 mil millones de dólares sería financiado por el gobierno de México. En ese mismo orden de ideas : también anunció que prohibiría la entrada a todos los inmigrantes musulmanes…Y atacó con vehemencia a los padres de un militar estadounidense de confesión musulmana, Humayun Khan, muerto en combate en 2004, en Irak.

También su afirmación de que el matrimonio tradicional, formado por un hombre y una mujer, es “la base de una sociedad libre”, y su critica de la decisión del Tribunal Supremo de considerar que el matrimonio entre personas del mismo sexo es un derecho constitucional. Trump apoya las llamadas “leyes de libertad religiosa”, impulsadas por los conservadores en varios Estados, para denegar servicios a las personas LGTB. Sin olvidar sus declaraciones sobre el “engaño” del cambio climático que, según Trump, es un concepto “creado por y para los chinos, para hacer que el sector manufacturero estadounidense pierda competitividad”.

Este catálogo de necedades horripilantes y detestables ha sido, repito, masivamente difundido por los medios dominantes no solo en Estados Unidos sino en el resto del mundo. Y la principal pregunta que mucha gente se hacía era : ¿ cómo es posible que un personaje con tan lamentables ideas consiga una audiencia tan considerable entre los electores estadounidenses que, obviamente, no pueden estar todos lobotomizados ? Algo no cuadraba.

Para responder a esa pregunta tuvimos que hendir la muralla informativa y analizar más de cerca el programa completo del candidato republicano y descubrir los siete puntos fundamentales que defiende, silenciados por los grandes medios.

1) Los periodistas no le perdonan, en primer lugar, que ataque de frente al poder mediático. Le reprochan que constantemente anime al público en sus mítines a abuchear a los “deshonestos” medios. Trump suele afirmar: « No estoy compitiendo contra Hillary Clinton, estoy compitiendo contra los corruptos medios de comunicación » . En un tweet reciente, por ejemplo, escribió : « Si los repugnantes y corruptos medios me cubrieran de forma honesta y no inyectaran significados falsos a las palabras que digo, estaría ganando a Hillary por un 20%.

Por considerar injusta o sesgada la cobertura mediática, el candidato republicano no dudó en retirar las credenciales de prensa para cubrir sus actos de campaña a varios medios importantes, entre otros : The Washington Post, Politico, Huffington Post y BuzzFeed. Y hasta se ha atrevido a atacar a Fox News, la gran cadena del derechismo panfletario, a pesar de que lo apoya a fondo como candidato favorito…

2) Otra razón por la que los grandes medios atacaron con saña a Trump es porque denuncia la globalización económica, convencido de que ésta ha acabado con la clase media. Según él, la economía globalizada está fallando cada vez a más gente, y recuerda que, en los últimos quince años, en Estados Unidos, más de 60.000 fábricas tuvieron que cerrar y casi cinco millones de empleos industriales bien pagados desaparecieron. 3) Es un ferviente proteccionista. Propone aumentar las tasas sobre todos los productos importados. « Vamos a recuperar el control del país, haremos que Estados Unidos vuelva a ser un gran país. », suele afirmar, retomando su eslogan de campaña.

Partidario del Brexit, Donald Trump ha desvelado que, una vez elegido presidente, tratará de sacar a EE.UU. del Tratado de Libre Comercio de América del Norte (NAFTA por sus siglas en inglés). También arremetió contra el Acuerdo de Asociación Transpacífico (TPP por sus siglas en inglés), y aseguró que, de alcanzar la Presidencia, sacará al país del mismo : « El TPP sería un golpe mortal para la industria manufacturera de Estados Unidos. »

En regiones como el rust belt, el «cinturón del óxido» del noreste, donde las deslocalizaciones y el cierre de fábricas manufactureras dejaron altos niveles de desempleo y de pobreza, este mensaje de Trump está calando hondo.

4) Así como su rechazo de los recortes neoliberales en materia de seguridad social. Muchos electores republicanos, víctimas de la crisis económica del 2008 o que tienen más de 65 años, necesitan beneficiarse de la Social Security (jubilación) y del Medicare (seguro de salud) que desarrolló el presidente Barack Obama y que otros líderes republicanos desean suprimir. Tump ha prometido no tocar a estos avances sociales, bajar el precio de los medicamentos, ayudar a resolver los problemas de los « sin techo », reformar la fiscalidad de los pequeños contribuyentes y suprimir el impuesto federal que afecta a 73 millones de hogares modestos.

5) Contra la arrogancia de Wall Street, Trump propone aumentar significativamente los impuestos de los corredores de hedge funds que ganan fortunas, y apoya el restablecimiento de la Ley Glass-Steagall. Aprobada en 1933, en plena Depresión, esta ley separó la banca tradicional de la banca de inversiones con el objetivo de evitar que la primera pudiera hacer inversiones de alto riesgo. Obviamente, todo el sector financiero se opone absolutamente al restablecimiento de esta medida.

6) En política internacional, Trump quiere establecer una alianza con Rusia para combatir con eficacia a la Organización Estado islámico (ISIS por sus siglas en inglés). Aunque para ello Washington tenga que reconocer la anexión de Crimea por Moscú.

7) Trump estima que con su enorme deuda soberana, los Estados Unidos ya no disponen de los recursos necesarios para conducir una politica extranjera intervencionista indiscriminada. Ya no pueden imponen la paz a cualquier precio. En contradiction con varios caciques de su partido, y como consecuencia lógica del final de la guerra fría, quiere cambiar la OTAN : « No habrá nunca más garantía de una protección automática de los Estados Unidos para los países de la OTAN. »

Todas estas propuestas no invalidan en absoluto las inaceptables, odiosas y a veces nauseabundas declaraciones del candidato republicano difundidas a bombo y platillo por los grandes medios dominantes. Pero sí explican mejor el por qué de su éxito.

En 1980, la inesperada victoria de Ronald Reagan a la presidencia de Estados Unidos había hecho entrar el planeta en un Ciclo de cuarenta años de neoliberalismo y de globalización financiera. La victoria hoy de Donald Trump puede hacernos entrar en un nuevo Ciclo geopolítico cuya peligrosa característica ideológica principal –que vemos surgir por todas partes y en particular en Francia con Marine Le Pen – es el ‘autoritarismo identitario’. Un mundo se derrumba pues, y da vértigo.

Este aterrador parásito es lo último que quieres ver después de pisar accidentalmente un grillo

Pisar un insecto es una circunstancia ya muy asquerosa de por sí, pero el horror alcanza nuevas cotas si lo que hemos pisado es un grillo afectado por nematomorfos. Este gusano parásito es tan desagradable que ha creado su propia leyenda en Chile y Argentina: la leyenda del pelo vivo.

El pelo vivo es un relato de los indios mapuches. Según la tradición, algunas mujeres con poderes mágicos (y malas intenciones) pueden dejar pelos vivientes en el agua que se cuelan por cualquier orificio corporal de las personas y provocan enfermedades o incluso la muerte.

Esto es lo que pasa cuando un enjambre de hormigas de fuego devora una cucaracha preñada

Ya lo decía Seth MacFarlane en aquella parodia de un programa de animales titulado: ¡Maldita sea,…Seguir leyendo

La leyenda es solo un mito en lo que a humanos concierne, pero es muy real para los grillos, las mantis o los saltamontes. Estos desafortunados insectos son el anfitrión favorito de los nematomorfos, también llamados a veces gusanos crin de caballo o gusanos gordianos. Se trata de un tipo de parasitoide que se introduce el el organismo de los grillos cuando estos comen otros insectos infectados con las pequeñas larvas del gusano. A partir de ese momento, los gusanos crecen en el interior del sistema digestivo del grillo o el saltamontes hasta alcanzar un tamaño que ocupa prácticamente toda la cavidad abdominal del insecto (hasta 30 cm, sí, centímetros), desplazando sus órganos pero manteniéndolo a su huésped con vida y funcional.

La manera que tienen los nematomorfos de matar a su anfitrión es más exótica. Cuando llega al estadio adulto, el gusano segrega neurotransmisores que vuelven loco al insecto. El infortunado grillo solo tiene una idea suicida en mente, que es encontrar una masa de agua lo bastante grande y arrojarse en ella.

Es en ese momento y solo en ese momento cuando los nematomorfos salen del insecto por la cloaca en un proceso tan fascinante como poco apto para estómagos delicados (sobre estas líneas hay un ejemplo). Eso es justamente lo que pasa cuando el excursionista pisa el grillo en el vídeo, que los gusanos se ven obligados a salir. Aunque no haya agua, los nematomorfos tratan de reproducirse formando un ovillo sobre el cadáver del grillo en un espectáculo que, aunque perfectamente natural, parece sacado de una película de terror. [Wired vía Science Alert]

El grito de un exbanquero condenado a muerte

 http://blogs.publico.es/escudier/2016/11/07/el-grito-de-un-exbanquero-condenado-a-muerte/

07/11/2016Francisco Luzón ha sido uno de los grandes banqueros del país. Participó en la fusión del Bilbao y el Vizcaya, fue presidente del Banco Exterior y de Argentaria y se jubiló en el Santander como responsable del negocio en Latinoamérica con una pensión de 65 millones de euros. Este domingo, en varias entrevistas de prensa, anunció que una esclerosis lateral amiotrófica (ELA) le estaba matando y que había decidido crear una fundación, a la que aportaría fondos, para convertirla en el embrión de una Comunidad Nacional de la ELA en la que participe el sector público y el privado, enfermos y médicos, investigadores y farmacéuticas, en busca de un remedio a lo que hoy es incurable.

De la existencia de esta mal llamada enfermedad rara, que afecta a unas 4.000 personas en España, supimos más hace un par de años, cuando todo el mundo empezó a echarse cubos de agua helada sobre la cabeza gracias a una campaña que, a diferencia de EEUU, aquí dejó escasos fondos. Una de sus variantes es la que tiene postrado a Stephen Hawking, aunque por lo general reduce la esperanza de vida a poco más de cinco años.

Para conocer cómo cursa este trastorno neuromuscular existe un blog estremecedor del periodista de El Confidencial, Carlos Matallanas, cuya última entrada fue del pasado día 12 de octubre, tras someterse a una traqueotomía de urgencia para poder respirar y ya prácticamente paralizado (“ya solo me puedo comunicar y seguir planeando escritos como este gracias a un ordenador que lee las pupilas”). Matallanas, que se sabe “en el corredor de la muerte”, ha ido relatando el avance de su enfermedad, de cómo recluye a sus víctimas entre las cuatro paredes de sus casas hasta hacerlas invisibles y de la indiferencia de unas administraciones que jamás se preguntan quienes sufren realmente su desidia y sus recortes.

Quizás a Luzón, por eso de que es rico, se le haga más caso, aunque se haya dado cuenta tarde de que la inversión para investigación en España es una quimera. El exbanquero empezó a tratarse en Estados Unidos, antes de descubrir que el Instituto de Salud Carlos III tenía una unidad de tratamiento multidisciplinar de la ELA “que, si se apoyase y se le añadiera potencia investigadora, podría convertirse en referente (…) Lo he hecho porque he descubierto que hay un gran equipo de profesionales (…) Pero lo que tengo claro es que nuestra estructura sanitaria no está bien gestionada para tratar enfermedades tan complejas como la ELA. Y eso me duele, porque parece que ningún gestor público lo entiende”, asegura en la entrevista de El País.

El Carlos III era desde 1990 el hospital de referencia para tratar la ELA y su equipo el encargado de realizar en España los ensayos clínicos internacionales para combatirla. Como, al parecer funcionaba, las autoridades sanitarias decidieron integrarlo en La Paz y liquidar la atención integral que sus neurólogos, psicólogos, neumólogos y fisioterapeutas prestaban a los pacientes.

Al frente de ese equipo se encontraba unos de los mayores especialistas en ELA, el doctor Jesús Mora, especialmente crítico con los recortes, contra el que se fabricó un expediente disciplinario en el que se le amenazaba con una suspensión de empleo y sueldo de dos años. Formado en el Massachusetts General Hospital y en el Tufts Medical Center de Boston, Mora, jefe de Neurología del Hospital Carlos II, dirige en España el proyecto MinE, un consorcio internacional que intenta descubrir la base genética de la ELA comparando el perfil de ADN de más de 15.000 enfermos con el de 26.000 controles a personas sanas. Gracias a este estudio han empezado a identificarse algunos de los genes que aumentan el riesgo de desarrollar la esclerosis lateral amiotrófica.

Así se funciona en un país en el que miles de enfermos de hepatitis C tienen que luchar para que la Administración no les deje morir con el argumento de que la medicación que les salvaría es cara y no estamos para dispendios, o que pudiendo aumentar la contribución a la investigación sobre la ELA la reduce a la mitad, que al fin y al cabo pocos van a enterarse ya que, aun siendo la tercera enfermedad neurodegenerativa con mayor incidencia, su prevalencia es baja porque quienes la padecen no tardan mucho en palmarla.

Un estudio de febrero de este año sobre el impacto económico y social de este tipo de enfermedades cifraba el coste medio en un año en 23.354 euros por paciente. El informe destacaba lo siguiente: “En términos estrictamente económicos, el principal impacto de la enfermedad (que no el único) recae sobre la disminución de oportunidades laborales que la enfermedad implica, no sólo para el propio afectado sino también para su cuidador principal. En relación con el impacto que la enfermedad tiene sobre los ingresos mensuales del cuidador principal, un 29% gana menos de lo que ganaba antes de declararse la enfermedad (…) El afectado y la familia asumen fundamentalmente la financiación de los proveedores de cuidados profesionales. De todo esto se deduce que las familias son en la actualidad las grandes proveedoras de servicios de apoyo a los enfermos con enfermedades neurodegenerativas, a coste cero para el sistema pero con un coste muy alto para sí mismas”. ¿Para qué gastar en lo que no nos cuesta?

Cada año se diagnostican en España alrededor de 900 casos de la ELA, los mismos que defunciones. Luzón estima en 2.000 millones los recursos necesarios para encontrar el tratamiento a una dolencia que lleva matando sin piedad a todos los que la sufren, ya sean banqueros, periodistas o fontaneros. “No podemos mirar hacia otro lado”, dice. Y lleva razón.

Nadie sabe cómo una de las mentes más brillantes de nuestro tiempo desapareció de la faz de la Tierra

Amanecer en el Océano Pacífico. WC

http://es.gizmodo.com/nadie-sabe-como-una-de-las-mentes-mas-brillantes-de-nue-1788538271

¿Qué ocurre cuando una de las mentes más brillantes de nuestro tiempo desaparece sin dejar rastro y sin motivo alguno? Que se organiza una de las búsquedas más épicas y asombrosas de cuantas han existido. Un rescate legendario.

Nadie sabe quién iba en este bote abandonado en el lugar más aislado del planeta

Es difícil hacerse una idea de lo lejos que esta Bouvet del resto del planeta. Seguramente la forma …Seguir leyendo

Hablamos de James Nicholas Gray, el hombre que ayudó como pocos a resolver problemas, el científico estadounidense que recibió el Premio Turing en el año 1998 por sus contribuciones a la base de datos y procesamiento de transacciones y a su liderazgo en la implementación de sistemas.

Sin embargo, una mañana del año 2007 todo cambió. Su misteriosa desaparición propició una de las más ambiciosas misiones de rescate de la historia. A la Guardia Costera se unirían un equipo formado por científicos, satélites, aviones de la NASA, Microsoft, Oracle, Amazon, Google, Bill Gates, la Marina de los Estados Unidos con sus oceanógrafos e ingenieros, los astrónomos de las principales universidades…

Esta fue la historia.

Gray antes de partir

Jim Gray en el 2006. Wikimedia Commons

Jim Gray nació en 1944 en San francisco, California. Fue el segundo hijo de una madre profesora, Ann, y padre del Ejército de los Estados Unidos, James. Al poco de nacer Jim los Grey se trasladan a vivir a Roma, espacio en el tiempo donde Grey pasó la mayor parte de su infancia. Allí aprendió italiano, además de inglés.

Poco después la familia regresa a Estados Unidos y se instala en Virginia, lugar donde los padres de Jim se divorcian y él regresa a la Bahía de San Francisco con su madre. Mientras, su padre James pasa a convertirse en inventor, momento en el que hace una pequeña fortuna patentando una especie de cartucho de tinta pionero para las primeras máquinas de escribir.

El día que disfrutó de su primer baño en el mar todo cambió. A partir de entonces, Jim supo que un día llegaría a tener un barco con el que emprender nuevas aventuras

Durante muchas de las visitas frecuentes al acuario y planetario en el Golden Gate Park, el joven Grey comienza a sentir fascinación por la ciencia. El chico devoraba libros y se atrevía a predecir que los ordenadores serían algún día lo suficientemente inteligentes como para jugar al ajedrez y enfrentarse a problemas sociales.

Paralelamente a la ciencia Jim también mostró una inusitada pasión por los viajes. A menudo acudía con su tío a Yosemite, espacio donde aprendió a sobrevivir en el bosque con muy pocos recursos, una de las “misiones” con las que su tío le desafiaba. Sin embargo, el día que disfrutó de su primer baño en el mar todo cambió. A partir de entonces, Jim supo que un día llegaría a tener un barco con el que emprender nuevas aventuras.

Cuartel general de IBM Research. Wikimedia Commons

Ocurre que ese sueño de joven no llegó a darse. A cambio, el Jim Gray capitán se convirtió en doctor en informática por la Universidad de Berkely. Apasionado por los ordenadores, comenzó su carrera en Bell Labscomo joven programador. Más tarde pasó a trabajar en IBM Research, espacio donde pasó a formar parte de un equipo de I+D que estaba tratando de convertir los modelos de base de datos de los bancos en un software funcional. Su trabajo se centró en las transacciones, y sus soluciones ahora las podemos encontrar en todo el mundo, desde la mismísima Amazon hasta la mayoría de grandes empresas como la misma Microsoft.

Gray había contribuido a varios de los principales sistemas de procesamiento de base de datos. Su equipo había creado el System R de IBM, el precursor que sentó las bases técnicas de una nueva industria. Más tarde trabajaría como investigador industrial y diseñador de software en varias compañías como Tandem y DEC hasta que en 2006 pasó a formar parte del equipo de Microsoft Research.

Su trabajo se centró en las transacciones, y sus soluciones ahora las podemos encontrar en todo el mundo, desde la mismísima Amazon hasta la mayoría de grandes empresas como la misma Microsoft

Un año después, en el 2007, Gray se podría decir que era un hombre feliz. Vivía en San Francisco con su segunda esposa Donna Carnes. El científico había conocido a la profesora de historia en 1984. De ascendencia noruega, Donna también era una experta marinera y excursionista. Ambos se habían convertido en uña y carne desde entonces, navegando y viajando siempre que podían.

Los dos disfrutaban de la embarcación que tenía en Gashouse Cove, un pequeño puerto deportivo en la Bahía. Se trataba de un crucero rojo de fibra de vidrio de unos 12 metros, una embarcación llamada Tenacious.

Un viaje sin retorno

Jim en el Tenacious. Wikimedia Commons

El 28 de enero del 2007 todo cambió. Gray se había despertado en el crucero. El programador de Microsoft tenía 63 años y nada más levantarse llama a su esposa, quién estaba de vacaciones con sus amigos en Wisconsin. Gray le dice que se iba a dirigir a los Farallones, una serie de pequeñas islas y peñascos a unos 43 kilómetros al oeste de las costas de San Francisco.

Un refugio de vida silvestre donde Gray iba a esparcir las cenizas de su madre Ann, quien había muerto en el mes de octubre. Para ello, el hombre se dirige a través del Golden Gate hacia el mar abierto. Los registros de aquel día marcaban marea y viento a favor. Sobre las 10:30 vuelve a llamar a su esposa y le dice que se estaba acercando a una boya con marca a unos 25 kilómetros de distancia.

Gray iba a esparcir las cenizas de su madre Ann, quien había muerto en el mes de octubre. Para ello, el hombre se dirige a través del Golden Gate hacia el mar abierto

Carnes recuerda preguntarle si llevaba puesto el arnés. Gray le respondió que sí y antes de cortar le dijo que la volvería a llamar más tarde. Minutos después el programador deja un mensaje de lo más optimista para su hija Heather. El mensaje dice:

Heather, estoy llevando a la abuela a su último lugar de descanso, estoy rodeado de delfines por esta zona, está un poco nublado, pero muy agradable, no hay ballenas sólo muchos delfines muy bonitos. Besos, te quiero mucho, cuídate, adiós.

Los Farallones. Wikimedia Commons

A las 11:50 su smartphone se sincroniza con el servidor de correo electrónico de Microsoft por última vez. Un par de horas más tarde, en el sureste de los Farallones, un naturalista llamado Brett Hartl vio un velero con un casco rojizo a dos o tres kilómetros en alta mar, una embarcación que se dirigía al norte.

Y a partir de aquí, Gray y su barco desaparecieron de la faz de la Tierra. La Guardia Costera no recibió ninguna llamada de Mayday y la EPIRB del barco de Gray, la radio de emergencia diseñada para transmitir una señal si se hunde, había permanecido en silencio. Ningún marinero de la zona reportó haber visto un barco a la deriva, un chaleco salvavidas o si quiera trozos de escombros del barco.

La red comenzó a lanzar todo tipo de teorías sobre el hombre. Entre ellas la posibilidad de que Gray fuera el nuevo D.B. Cooper

Obviamente, la noticia de su desaparición sorprendió a la comunidad de Silicon Valley. Gray ya era una leyenda cuyo trabajo ayudó a hacer posible muchos de los pilares de la vida moderna, desde los cajeros automáticos hasta el comercio online o los sistemas de la todopoderosa Google.

Desde Sergey Brin, ferviente admirador de su trabajo, hasta la propia Microsoft, de la que actualmente formaba parte e incluso había convencido para que construyeran un centro de investigación en San Francisco para que él y su esposa no tuvieran que mudarse. Incluso su influencia iba mucho más allá de la codificación y los ordenadores, Gray se había labrado un círculo cercano donde había de todo, desde astrónomos hasta oceanógrafos, geólogos, genetistas…

Norte de los Farallones. Wikimedia Commons

Cuando la búsqueda se hizo pública a nivel nacional, la red comenzó a lanzar todo tipo de teorías sobre el hombre. Entre ellas la posibilidad de que Gray fuera el nuevo D.B. Cooper, aunque lo cierto también, es que Gray no tenía ningún motivo para ello.

Desde el momento que se inició la búsqueda su esposa permaneció aislada sin hablar con la prensa. Aunque poco después accedió a hablar con el periodista S. Silberman para ofrecer pistas sobre la vida de Gray y el rescate que estaba a punto de comenzar. Según le dijo a Silberman:

Estoy decidida a averiguar qué le pasó a mi marido. Y también estoy decidida a encontrar a Tenacious, porque el barco es la llave hacia Jim y hacia este extraño, singular y doloroso misterio.

Una búsqueda legendaria

Inicios de la búsqueda. Getty

Como decíamos al comienzo, la esposa de Gray se encontraba en Wisconsis esquiando con un grupo de amigos. Cuando llegó la noche y preocupada por no tener noticias de su esposo, llamó al capitán del puerto, quién le verificó que el barco no había regresado. El capitán llamó a la Guardia Costera y el comandante adjunto David Swatland se haría cargo de las labores de búsqueda.

Lo primero que hizo el equipo de Swatland fue transmitir una descripción del velero a los marineros de la zona. Más tarde un barco patrulla salía del puerto en busca del científico. Al barco se unió durante la madrugada un helicóptero y un avión C-130 Hercules, ambos equipados con radar de imágenes térmicas, y avanzaron por toda la zona de los Farallones.

La mujer piensa que, dado el gran número de ilustres amigos del programador y las conexiones de estos en ámbitos de todo tipo, podrían ayudar al rescate

A la mañana siguiente (lunes) la Guardia Costera envió un comunicado estándar a la prensa sobre un marinero desaparecido. Por aquel entonces Swatland no tenía la menor idea de quién era gray ni lo conocido que podía ser en algunos círculos. Sin embargo, en muy poco tiempo los medios locales conectaron su nombre con Microsoft. En ese momento las cámaras de televisión se dirigieron a Yerba Buena y la noticia se difundió rápidamente entre los colegas y amigos de Jim.

Paula Hawthorn fue de las primeras en enterarse. La ex presidenta de Informix conocía a Gray desde hacía más de 35 años y decide buscar la manera de organizar una ayuda. La mujer piensa que, dado el gran número de ilustres amigos del programador y las conexiones de estos en ámbitos de todo tipo, podrían ayudar al rescate.

C-130 Hercules. Wikimedia Commons

Paula decide enviar un correo electrónico a un grupo central de personas con una idea simple. La mujer sugería el uso de imágenes satelitales para complementar el trabajo de los barcos y avión de la Guardia Costera. Un mensaje que finalizaba con un: ¿Con quién tenemos que hablar?

Una de las personas a las que le llegó el correo fue al mismísimo Sergey Brin, co-fundador de Google, quien prometió hablar con la empresa de satélites DigitalGlobe, el principal proveedor de imágenes de los de Mountain View. La lista de celebridades del sector tecnológico era enorme, y como Brin, la mayoría estaba dispuesto a colaborar en lo que fuera. Se había creado un grupo de trabajo para el rescate bajo el apodo de Los amigos de Jim.

La lista de celebridades del sector tecnológico era enorme, y como Brin, la mayoría se puso manos a la obra añadiendo sensores y tecnología como posibilidad

A mitad de semana Swatland ya había asignado más barcos, helicópteros y personal para la búsqueda. También se habían realizado simulaciones por ordenador para predecir por dónde podría estar a la deriva el barco en el supuesto de que el hombre hubiera sufrido un percance que le haya dejado inmóvil.

Ese día se suma a las ayudas Joe Hellerstein, profesor de ciencias de la computación de la Universidad de Berkeley y ex-alumno de Gray. Hellerstein se encargó de lanzar una web para coordinar esfuerzos online. Por su parte Microsoft, la empresa actual de Gray, trabajó junto a la Guardia Costera para aportar recursos técnicos a la búsqueda.

Swatland durante una de las ruedas de prensa. Getty

Curiosamente, entre la propia comunidad tecnológica la desaparición del científico sonaba a deja vú. Meses antes, en noviembre del 2006, el editor de la web CNET, James Kim y su familia, habían desaparecido en las montañas de Oregón. La esposa e hijos de Kim se salvaron porque dos ingenieros de Edge Wireless investigaron los registros telefónicos, lo que ayudó a un piloto de rescate a localizarlos justo a tiempo. Kim en cambio fue encontrado muerto dos días después.

Cingular (AT&T actualmente) aceptó la petición de Swatland sobre los registros completos del teléfono de Gray para poder triangular su último punto de contacto. Había esperanza de que Gray se mantuviera con vida. Además, el tiempo estaba cooperando, el cielo estaba cristalino y el mar tranquilo.

Meses antes, en noviembre del 2006, el editor de la web CNET, James Kim y su familia, habían desaparecido en las montañas de Oregón

Poco a poco el área de búsqueda se fue ampliando, pero mientras eso ocurría, la Guardia Costera debía empezar a considerar otras posibilidades, opciones como que el propio Gray no quisiera ser encontrado. Pero lo cierto es que no había un patrón para creerlo. Gray no tenía problemas financieros y parecía un tipo tremendamente feliz y enamorado de su esposa.

Quizá había un punto a considerar. Carnes le contó a Silberman que tras la muerte de la madre del programador en octubre, falleció el padre de ella. Gray estuvo afectado en los últimos meses de vida de su madre mientras la veía perder su capacidad para andar. Tras el fallecimiento le llegó a confiar a su esposa que había visto su futuro y que no era nada bueno. Pero incluso triste, nadie creía que el científico hubiera decidido abandonarse a la suerte en el mar. Era un escenario improbable.

Werner Vogels. Wikimedia Commons

Otra de las personas que se encontraban en la larga lista de los amigos de Jim era Werner Vogels, CTO y vicepresidente de Amazon.com en Seattle. Y es que Gray también fue profesor de prometedores principiantes como el propio Vogels, quién llegó a pedirle consejo tras la llamada de Jeff Bezos para contratarle en Amazon.

Rick Rashid, VP de Microsoft, también estaba en esa nutrida lista. Él fue quién reclutó a Gray en Microsoft (1995) en un momento donde las grandes firmas estaban detrás del hombre. O el astrónomo Alex Szalay, de la Universidad Johns Hopkins, con quién participó en un proyecto masivo de cartografía estelar a la web (Sloan Digital Sky Survey) de forma que los datos fueran accesibles para los astrónomos y astrólogos profesionales y estudiantes.

Cuando el mundo académico y científico cuyas carreras habían florecido bajo su influencia se enteró de que Gray se había perdido en el mar, se organizaron para ayudar sin dudarlo ni un instante

El resultado fue SkyServer, probablemente, el recurso astronómico más utilizado en el mundo y actor principal para el descubrimientos en materia de galaxias enanas o materia oscura desencadenadas por el Big Bang. Así que en general, cuando el mundo académico y científico cuyas carreras habían florecido bajo su influencia se enteró de que Gray se había perdido en el mar, se organizaron para ayudar sin dudarlo ni un instante.

Desgraciadamente, a los tres días de su desaparición Swatland celebró una conferencia de prensa para explicar lo siguiente:

Hemos peinado kilómetros de océano. No podemos buscar indefinidamente y siempre es difícil decidir cuando debes parar. La Guardia Costera no ha encontrado nada. El 1 de febrero se cancela la búsqueda oficialmente.

Alex Szalay. Wikimedia Commons

Pero los amigos de Jim no iban a parar. De repente, en la web comenzó a rondar la posibilidad de buscar a Gray con las mismas herramientas que él mismo había ayudado a inventar. En el fatal evento ocurrido con el editor de CNET ya se había sugerido el uso del crowdsourcing como un esfuerzo de rescate y ayuda online. ¿Qué hicieron?

El primer paso fue viralizar su rescate en redes, que su imagen se hiciera omnipresente. YouTube, subir imágenes del científico en su embarcación, enlaces a la noticia desde todas las webs… Microsoft por su parte decidió que sus esfuerzos se mantendrían fuera de los focos de los medios, quizá para no entorpecer las labores. Rick Rashid hizo saber que el dinero no iba a ser un problema y que si la Guardia Costera abandonaba, buscarían fuerza aérea privada. Bill Gates salió a escena y también ofreció personalmente toda la ayuda necesaria. Junto a Microsoft se coordinaron esfuerzos con Google para obtener imágenes por satélite.

Rick Rashid hizo saber que el dinero no iba a ser un problema y que si la Guardia Costera abandonaba, buscarían fuerza aérea privada

Y entonces ocurrió algo inesperado. Quizás por la presión mediática, quizás porque se arrepintió, Swatland redobló esfuerzos y no abandonó la búsqueda. Se desplegaron dos aviones C-130, tres helicópteros, tres patrullas y cuatro barcos para aventurarse en la mar en busca del científico.

Después de hacer una lista de buques importantes que podrían haber representado una amenaza para Gray y su embarcación, la Guardia Costera envió a los investigadores a examinarlos y detectar si existían signos de colisión. Ninguna de ellos mostraba señales o daño alguno, razón por la que Swatland desechó tal posibilidad.

El tiempo jugaba en contra del programador. Si seguía vivo, tenía muy pocos días de comida en el barco. Peor aún, se avecinaba mal tiempo y una tormenta en los próximos días.

Vista aérea de la Bahía de San Francisco. Wikimedia Commons

A media que la Guardia Costera redoblaba esfuerzos de búsqueda el trabajo de los amigos de Jim comenzó a dar resultados. La Agencia Espacial Canadiense había cedido el satélite Radarsat-1, el cual inspeccionaría el área de búsqueda con sensores térmicos. Poco después se sumaba a las labores el satélite QuickBird con una serie de pases de alta resolución apoyados en GeoEye.

La NASA se sumó más tarde ofreciendo un piloto para un vuelo de entrenamiento en un ER-2, algo así como un equivalente civil de un avión espía, con la idea de cubrir la costa con una cámara de infrarrojo cercano. Llegó un momento con el discurrir de las horas, en el que el área del océano que salía desde Golden Gate se había convertido en uno de los lugares más documentados de todo el planeta.

El área del océano que salía desde Golden Gate se había convertido en uno de los lugares más documentados de todo el planeta

La catarata de datos creó un nuevo conjunto de desafíos. Originalmente Microsoft y Amazon se habían ofrecido a utilizar un sofisticado software de procesamiento de datos para filtrar los millones de píxeles de cualquier cosa que parezca un barco a la deriva. Pero estos esquemas tenían un problema: las nubes que se movían por el Pacífico iban a enrarecer los algoritmos.

¿Qué se puede hacer cuando la tecnología no alcanza? Volver al principio, volver al hombre. El ojo humano iba a ser el mejor recurso para escoger entre imágenes borrosas y Amazon había lanzado hacía muy pocos meses el servicio Mechanical Turk que permitía contratar trabajadores online para tareas a corto plazo que los equipos informáticos no pueden hacer bien.

Golden Gate. Wikimedia Commons

A las pocas horas Vogels publicó una solicitud de voluntarios en su blog bajo el título de “Ayuda a encontrar a Jim Gray”. Dicha solicitud se hizo viral en minutos, aterrizó en la portada de Digg (el Reddit de antes) y salió un artículo en el New York Times. Unas horas después había 12 mil voluntarios inscritos.

Mientras, el astrónomo Alex Szalay organizó un equipo alternativo para analizar los mismos datos de imágenes entre la Johns Hopkins, la Universidad de Hawai y el Space Telescope Science Institute en Baltimore. A Szalay se unieron analistas experimentados de la Federation of Earth Science Information Partners, agencias federales… Todos trabajando en conjunto, todos bajo un increíble e innato sentido de la urgencia de los acontecimientos, todos, tratando de hacer posible lo imposible.

Cerca de 20 imágenes fueron etiquetadas como “probables” y una fue etiquetada como “muy probable”

La transferencia de los cientos de gigabytes de píxeles en un formato estándar y en piezas más pequeñas para su distribución ya fue difícil. Pero cuando el primer lote llegó a los servidores de Amazon aquello fue una decepción. Les tomó horas y días cruciales eliminar y “limpiar” cada imagen, una tarea titánica que en la mayoría de ocasiones era insensible a los ojos, imposible discernir si eran nubes, islotes o el barco de Gray.

Ocho días después de que Gray desapareciera, los voluntarios de Amazon habían escrutado miles de kilómetros de mar. Cerca de 20 imágenes fueron etiquetadas como “probables” y una fue etiquetada como “muy probable”. Estas imágenes fueron marcadas con comentarios y enviadas a un panel de expertos analistas de satélites convocados por Szalay y gente de Darpa.

Había un nuevo problema antes de enviar aviones a las zonas. Debido a que habían pasado varios días desde la toma de las imágenes, muy probablemente estos objetos ya no estaban en esa ubicación. Los amigos de Jim necesitaban una manera de predecir hacia donde podría haberse desviado un velero de esas características dado el tiempo y las corrientes.

Farallones. Wikimedia Commons

Aquí entró en escena el oceanógrafo Jim Bellingham, antiguo alumno de Gray. Necesitaba herramientas para construir simulaciones y trazar trayectorias de deriva, para ello ensambló un equipo de modeladores del océano de la marina, de la NASA y de la Universidad de Oregon. Para obtener los datos actualizados sobre las corrientes rastrearon las boyas de radio de la Guardia Costera y monitorearon las condiciones costeras. Luego publicaron animaciones en GIF en la web mostrando dónde se encontraría probablemente un objeto visto por un satélite dos días antes.

Pero cuando los aviones estaban preparados para salir en busca de los objetivos más prometedores, el clima volvió a intervenir. Lluvia y fuertes vientos retrasaron los vuelos. Cuando la meteorología dio tregua, los aviones acudieron a todos los lugares sin encontrar una pista que arrojara luz.

El 11 de febrero su esposa Carnes emprendió un viaje con rumbo a los Farallones. Había alquilado un barco pesquero y a un experto en búsqueda marina

Esos días de más antes de enviar los aviones habían desecho la labor de búsqueda más sofisticada y asombrosa de la historia. A pesar del potencial financiero y técnico de los amigos de Jim, ya no quedaban opciones. Se había perdido la esperanza de encontrarlo con vida, lo que imposibilitaba comenzar a crear nuevas herramientas que tardarían días o semanas en encontrar posibles nuevas soluciones.

El 11 de febrero su esposa Carnes emprendió un viaje con rumbo a los Farallones. Había alquilado un barco pesquero y a un experto en búsqueda marina. Lo único que encontraron en su travesía fueron cientos de troncos y madera en el agua, probablemente debido a las tormentas de días anteriores.

Ni Google, ni Amazon, ni Microsoft, ni la NASA, ni el mayor conjunto de expertos científicos pudieron dar con el paradero de una de las mentes más brillantes de nuestro tiempo. En cambio, su desaparición proporcionó una serie de métodos de búsqueda y rescate inauditos en la red que pueden servir en el futuro para salvar otras vidas.

Parece poco probable mantener la esperanza, aunque tratándose del científico, nadie se atreve a verificar su muerte. Ni siquiera la propia Wikipedia, quien comienza su entrada con un escenario improbable, pero no imposible:

Jim Gray, presumiblemente muerto…

Los padres (y madres) somos víctimas pero también culpables de las tareas escolares

Buen artículo aunque con bastantes cosas a puntualizar. En rojo. Obviamente hablo desde mi experiencia en secundaria. Primaria es otra historia.

https://www.lainformacion.com/opinion/treintaymuchos/huelga-deberes-padres-tareas-colegio-madres_0_968304401

• La reacción de los padres (y madres) contra los deberes está justificada, pero no en las formas.
• No hay nada más devastador en la educación de los hijos que desautorizar desde casa a los maestros.

Muchas madres piden reducción de jornada para estar con sus hijos mientras hacen los deberes. GettyImages

Debo confesar que mi criterio como padre respecto a los deberes escolares cambió cuando comencé a sufrirlos. Miento. Cuando comenzó a sufrirlos mi mujer, que es la principal perjudicada. Porque esa es otra: los deberes escolares repercuten principalmente en las mujeres. Creo que en lo que voy a relatar pueden verse reflejados millones de padres.

Partamos de aquello en lo que hay un consenso unánime entre padres y profesores:

• Las horas de colegio deberían bastar para aprender lo necesario en cada edad.  No saben qué cansado estoy de escuchar esto. Es FALSO  y demuestra que quien lo dice no sabe de qué habla. ¿Sería lo ideal? Sí, claro que sí, pero es IMPOSIBLE. Empezando porque la mayoría de asignaturas tienen 2-3 clases de 50 minutos a la semana, y más de la mitad de la clase se dedica en poner orden ya que a la mitad de los chavales les importa muy poco lo que allí se está haciendo y se les obliga a estar allí. Y otra es el reparto de horas entre asignaturas. Hay asignaturas como Matemáticas que hay que practicarlas mucho y que, aunque sean de las asignaturas que más horas tienen, sigue sin ser suficiente. Pero si se pide reducir el número de horas de otras asignaturas enseguida los sindicatos y grupos de esas asignaturas saldrán a defender lo suyo.
• Conviene que los niños y adolescentes realicen actividades físicas para crecer sanamente y prevenir enfermedades. Obviamente.
• Los niños deben dedicar tiempo a estar con sus padres y hermanos: a hablar con ellos, a jugar, a comer… y a pelearse, si es preciso. Está claro.
• Los juegos, los amigos, las aficiones, etc, forman parte de la educación. Nadie dice que no.

Las cuatro consideraciones anteriores, y otras que omito para no resultar prolijo, desembocan en la clave de esta cuestión: el TIEMPO. Y eso, tiempo, es lo que devoran sin compasión los deberes escolares, en perjuicio de padres y, sobre todo -insisto de nuevo-, madres.

No me he encontrado a ningún padre de familia, y he conocido a muchísimos, que no esté de acuerdo en el provecho que obtienen los niños al dedicar un tiempo razonable al estudio. Pero por tiempo razonable entiendo aquel que no te impide desarrollar con normalidad las actividades que mencionaba antes.

Niños condenados a perder

Desde hace tiempo los padres protestan porque una sobrecarga de los deberes escolares está alterando la vida familiar. Algunos de ellos resultan absurdos, amén de irrealizables por los niños, al menos (y aquí parte del problema) sin ayuda externa. No lo creo, a menos que el profesor sea realmente muy malo y no sabe qué está mandando. En general todo lo que se manda ha sido explicado en clase. Otra historia es que el chaval no haya estado atento o que sus compañeros no le hayan dejado estar atentos. Pongo un ejemplo: los deberes que incluyen la utilización de programas informáticos que ni el niño ni sus padres tienen por qué conocer o tener en casa, como editor de vídeos, Power Point o Photoshop. Hay profesores que dan por hecho que todas las familias deben disponer en casa de internet de alta velocidad en casa o impresora a color, como si fueran tan imprescindibles como la electricidad o el suministro de agua. Existen las bibliotecas en el pueblo e incluso en colegios e institutos donde se puede disponer de ello. Lo de la impresora ya es más relativo pero se puede imprimir en muchos sitios, no necesariamente en casa.

En esta tesitura, hay unos niños claramente condenados a perder. Me refiero a aquellos que no disponen de ayuda de un adulto en casa a la hora de realizar las tareas, bien porque sus dos padres llegan tarde de trabajar o bien porque el nivel académico de las consultas excede la preparación intelectual de los progenitores o tutores. Un niño no tiene porqué necesitar ayuda de nadie si ha estado atento en clase y su progresión ha sido la adecuada. En mi casa mis padres jamás pudieron ayudarme (igual que la mayoría de mis compañeros) y salimos adelante.

Los padres con recursos económicos pueden solventar esta carencia contratando a un profesor particular, pero… ¿y los que no los tienen? La enfermedad de las clases particulares. En mis tiempos casi nadie iba a clases particulares. Ahora más de la mitad de los padres lleva a sus hijos a clases particulares en lugar de acostumbrar a sus hijos a sacarse ellos las castañas del fuego. Y sí, es injusto para quien no se lo puede pagar, pero eso ha sucedido siempre: quien puede pagarse el conservatorio, el judo/karate, un colegio de pago, una universidad privada,…

Reducción de jornada… para las madres

Por otro lado, cuando los hijos atraviesan dificultades académicas hay padres que se plantean pedir una reducción de jornada para estar en casa cuando ellos llegan del colegio. ¿Quién suele pedir dichas reducciones en este país? La madre. ¿Cuál es el resultado? El obvio, el que reflejan todas las estadísticas: La maternidad perjudica el desarrollo profesional mucho más que la paternidad.

Creo que la mayoría de los padres podría suscribir lo que he relatado hasta ahora. Hay excepciones, por supuesto, pero solo confirman la regla. Los deberes escolares, que también contienen numerosas ventajas en tanto contribuyen a fomentar la laboriosidad y la disciplina del menor, acarrean otros daños colaterales a las familias no siempre tenidos en cuenta.

Dicho lo cual, y me pesa decirlo, no estoy para nada de acuerdo con la primera huelga de padres que ha anunciado la Confederación de Asociaciones de Padre de Alumnos (CEAPA). Por una sencilla razón: no hay nada más devastador para un menor que ver a los mayores desautorizándose entre ellos.

Ni las madres ni los padres deben cuestionar jamás a un profesor delante de un menor. Cuando ocurre tal cosa, se le hace más daño que si le enseñas que 2+2 son 5. Totalmente de acuerdo. Ahí empiezan muchos de los problemas. Y no digamos el consabido «mi padre dice que tienes que aguantarme», que muchos chavales ya traen aprendido de casa.

Comprendo que CEAPA quiera lanzar un justificado grito de desesperación. Miles de padres ya no saben qué hacer para que el sector educativo afronte un problema real que perjudica su vida cotidiana. Pero esas no son formas.

Los errores de los padres

Además, mentiríamos si eximiéramos de todo culpa a los padres en este espinoso asunto. Los padres debemos enseñar a nuestros hijos que las cosas cuestan un esfuerzo. A veces, muchísimo esfuerzo. Los deberes nos ayudan en ese cometido. Ante la pregunta quejumbrosa de un hijo “¿me ayudas a hacer estos problemas?”, a veces no existe respuesta más educativa que “no, hazlo tú solito”.

Y si se equivoca, que le suspendan. Quizá la próxima vez pondrá más atención en clase. O le pida ayuda a un compañero. O estudie más.

La casuística es variada. No digo que todos los niños que suspendan sean unos vagos. Pero ¿a caso no es verdad que muchos fracasan porque los padres no les hemos enseñado a sufrir adversidades, al menos no de manera creativa, poniendo soluciones prácticas para acabar con el sufrimiento?

Cualquiera que haya tenido atragantada una asignatura sabe la gran satisfacción que se siente cuando, después de mucho trabajar, estudiar y porfiar, consigue aprobar el examen.

Sí, en el colegio y en casa también hay que ayudar a resistir y gestionar los vientos contrarios. Eso evitará más adelante afrontar sin desmoronarse contratiempos tan diversos como desengaños amorosos, injusticias laborales o enfermedades imprevistas.

No atenta contra los derechos humanos hacer que un niño se levante todos los días una hora antes para estudiar matemáticas. Eso muchas veces significará, claro está, que el padre o madre tendrá que levantarse a las ocho menos cinco para despertar al niño. Pero es que nadie dijo que educar sea fácil. Tener un hijo implica esfuerzo. Esfuerzo que debe transmitirse al niño como un imperativo vital: los padres cuidaremos de ti, pero tú, en justicia, estás obligado a esforzarte para ser autónomo.

Si la polémica de los deberes ayuda a cuestionarnos qué estamos haciendo mal padres y profesores con la educación de los hijos, bienvenida sea la polémica. Pero huyamos de las soluciones simples. Cuidemos las formas, que también son importantes, por el bien de los menores.

Sigue @martinalgarra

 

Una última puntualización: en secundaria volvemos al problema de siempre, en muchas clases es muy difícil impartir docencia y hay que dedicar más de la mitad de la clase en poner orden. Especialmente en 1º y 2º de la ESO. Los daños colaterales para los chavales que quieren hacer algo son devastadores. Pero nadie se plantea quitar la obligatoriedad o dirigir a los «malos» alumnos hacia otras opciones. En España eso se considera «segregar».

Yo invito a cualquier padre a que se pase una semana en las clases de 1º y 2º de la ESO de un instituto «normal» y se dará cuenta enseguida de cuál es el problema con la educación que tenemos en este país.

Así ataca el tabaco a nuestro ADN para provocar 17 tipos de cáncer

http://www.agenciasinc.es/Noticias/Asi-ataca-el-tabaco-a-nuestro-ADN-para-provocar-17-tipos-de-cancer

La ciencia demostró hace décadas que el tabaquismo aumenta el riesgo de cáncer. Ahora, un nuevo estudio relaciona por primera vez varias clases de tumores con el daño genético que sufren los órganos y tejidos de los fumadores. Y aporta datos contundentes: consumir un paquete al día durante un año causa 150 mutaciones en las células de los pulmones.

Fumar es un factor de riesgo para al menos 17 tipos de cáncer y está detrás de más de seis millones de muertes cada año. / Ana Penagos

El humo del tabaco contiene más de 7.000 productos químicos, entre ellos, más de 70 conocidos por ser potenciales carcinógenos. La literatura científica insiste desde hace años en que fumar es un factor de riesgo para al menos 17 tipos de cáncer y está detrás de más de seis millones de muertes cada año. Sin embargo, los mecanismos por los cuales se daña el genoma y se producen las mutaciones que desencadenan la enfermedad aún no se entendían completamente.

Ahora, un equipo internacional de investigadores ha demostrado por primera vez que el hábito de fumar aumenta el riesgo de cáncer al causar mutaciones en los tejidos directa e indirectamente expuestos al humo. Los resultados se publican esta semana en la revista Science.

El estudio, liderado por científicos del Laboratorio Nacional de Los Alamos (Nuevo Mexico, EE UU) y del Instituto Wellcome Trust Sanger de Gran Bretaña, identifica los signos de mutación asociados al tabaco tras el análisis del genoma de más de 5.000 tumores humanos de fumadores y no fumadores.

Han demostrado que fumar aumenta el riesgo de cáncer al causar mutaciones en los tejidos expuestos al humo

“La secuenciación de los genomas del cáncer y el examen de las firmas mutacionales nos proporciona nuevas pistas sobre los procesos que causan los tumores. Este estudio puede preparar el camino para nuevas estrategias de prevención”, explica a Sinc Ludmil B. Alexandrov, investigador del laboratorio estadounidense y uno de los autores del trabajo.

Los expertos han medido el daño genético causado en diferentes órganos del cuerpo y han descrito los mecanismos que originan las mutaciones en el ADN.

En su estudio encontraron que los fumadores acumulaban un promedio de 150 mutaciones adicionales en cada célula pulmonar –97 en las células de laringe, 39 en las de faringe, 23 en las de boca, 18 en las de vejiga y 6 en las de hígado– por cada año fumando un paquete de cigarrillos al día.

“Las mutaciones asociadas con el humo del tabaco son más complejas de lo que se pensaba anteriormente”, añade Alexandrov. «El tabaco daña el ADN en los órganos directamente expuestos al humo y acelera un reloj celular mutacional en los órganos que están directa e indirectamente expuestos».

Humo del tabaco y cáncer

El estudio ha identificado las ‘firmas’ de mutación y los mecanismos epigenéticos en las miles de secuencias del genoma analizadas. «El genoma de cada tumor proporciona una especie de registro arqueológico de las exposiciones causantes de las mutaciones que conducen al cáncer”, apunta Mike Stratton, del Instituto Wellcome Trust Sanger.

Mutaciones producidas anualmente en cada célula (de pulmón, laringe, faringe, boca, vejiga e hígado) al fumar un paquete al día. / Broad Institute

Todos los cambios en el ADN –inofensivos o cancerosos– se deben a procesos de mutación endógena o exógena. Cada proceso deja una firma en el código de ADN que queda impresa en los pares de bases del genoma de esa célula, identificadas por las letras A, T, G y C.

El nuevo estudio encontró más de 20 huellas de mutaciones en los 17 tipos de cáncer asociados con el tabaquismo. Sin embargo, solo cinco de estas marcas fueron significativas en los cánceres sufridos por los fumadores.

El genoma de cada cáncer proporciona una especie de registro arqueológico de las exposiciones causantes de las mutaciones

Algunos tipos de cáncer tenían solamente una firma mutacional elevada en fumadores, mientras que otros tenían múltiples. Según los autores, la firma 4 es particularmente destacada en los cánceres de tejidos directamente expuestos al humo, como los pulmones y la laringe, y se produjo con menos frecuencia en los cánceres de tejidos indirectamente expuestos, como la vejiga.

Por su parte, la firma 5 –el signo mutacional más ampliamente difundido– se encontró en todos los tipos de cáncer y desencadenó mutaciones regulares en los fumadores frente a los no fumadores.

“Los órganos que no están en contacto con el humo inhalado no tienen la misma firma mutacional directa que se ve en los pulmones”, afirma Alexandrov. “Sin embargo, existe otra en todas las células cancerosas que acelera la tasa de mutación en las células de fumadores. Cuantas más mutaciones tenga una célula, más probabilidades hay de que una de ellas esté en un gen causante de cáncer y lo provoque”.

Apenas había pequeñas diferencias en los cambios epigenéticos que sufrieron los tumores de fumadores frente a los de no fumadores. “Esto sugiere que son las mutaciones genéticas el principal mecanismo por el cual el tabaquismo aumenta el riesgo de cáncer”, concluyen los autores.

Referencia bibliográfica:

L.B. Alexandrov; Y.S. Ju; K. Haase; P. Van Loo; I. Martincorena; S. Nik-Zainal; P.J. Campbell; M.R. Stratton; T. Shibata. ‘Mutational signatures associated with tobacco smoking in human cancer’. Science, 3 de noviembre de 2016.

http://science.sciencemag.org/cgi/doi/10.1126/science.aag0299

Aliens, el regreso: El logro que se gestó en un aparcamiento

http://www.valenciaplaza.com/aliens-el-regreso-el-logro-que-se-gesto-en-un-aparcamiento

Sigourney Weaver, en un momento de la película

Hace 30 años llegaba a los cines españoles la segunda entrega de la franquicia, la obra maestra de James Cameron, una película que estuvo en el cajón y cuyo turbulento rodaje se puso en marcha tras una conversación en un parking

 

Fue en el cine Serrano de Valencia, el mismo donde hacía siete años se proyectó la primera entrega. Fue un miércoles, 29 de octubre de 1986, hace ahora 30 años. Convertida ya en un éxito, tras sus excelentes resultados en Estados Unidos y Canadá, llegaba a los cines para el puente de Todos los Santos la que para muchas es una de las mejores secuelas de la historia del cine. Aliens (James Cameron 1986) se estrenaba con el reto de mantener el nivel de calidad y éxito de su predecesora, Alien (Ridley Scott, 1979), algo que se presumía difícil. Como diría el propio Cameron, el film de Scott había puesto “el listón muy alto”. Una gran marquesina pintada por Misas presidía el paseo Ruzafa, al cual miraba una Sigourney Weaver aterrorizada sujetando a una niña pequeña mientras contemplaba un peligro inminente que el espectador sólo podía intuir.

La llegada de Aliens rompía un tanto la lógica industrial de esos años. Si bien hoy son moneda común las secuelas, casi todas innecesarias, a principios de los años ochenta era difícil que una productora insistiera con un film repitiendo personajes y situaciones. Secuelas existían, obviamente, pero sobre todo en el cine de serie B. Excepciones hechas como Bond, en las grandes majors no estaba tan extendida la mentalidad de franquicia que hoy impera en Hollywood, que hace que cualquier película que venda más de dos entradas tenga su continuación. Por eso, pese al éxito del film original, dos nominaciones a los Oscars y más de 100 millones recaudados en taquilla, en la Fox, propietaria de los derechos, no existía mucho interés por realizar una continuación. David Giler y Walter Hill, productores y coguionistas de la primera entrega, trabajaban pues sin mucha fe en el proyecto conscientes de que, por ejemplo, Norman Levy, uno de los capitostes de la major, era uno de los mayores escépticos.

Todo dio un giro de 180 grados tras un cambio en la propiedad de la Fox. El momento clave se produjo en un aparcamiento de la productora. Giler se encontró con uno de los nuevos ejecutivos, a quien conocía de antes. Sin mucha convicción, quizás por mera cortesía, el ejecutivo le preguntó qué proyecto estaban preparando. Giler le refirió su idea de la secuela de Alien, en la que estaba trabajando con Walter Hill, un cruce entre la excelente película de su socio La presa (1981) y el clásico Los siete magníficos (John Sturges, 1960), todo en un contexto de cine fantástico. “Suena bien”, le respondió el ejecutivo. A los pocos días Hill y Giler tuvieron una reunión con los nuevos responsables del estudio y les dieron el visto bueno. Comenzaba pues la secuela de Alien. Faltaba director.

Con presteza pero sin atropellarse, Giler y Hill se entrevistaron con James Cameron, quien hasta la fecha no tenía lo que se dice un gran currículum como realizador: sólo había estrenado el bodrio Piraña II: Los vampiros del mar (1981) y todavía estaba pendiente de rodar la que sería su primera obra maestra, Terminator (1984). Tenía fama de guionista habilidoso y se le contrató con la idea de que fuera el responsable del libreto final, si bien Giler tuvo claro desde el principio que le quería también tras las cámaras. Mientras pensaban en cómo convencer a la Fox, dónde cómo es lógico no apostaban por Cameron, le dieron margen de tiempo para que concluyera el rodaje de Terminator que se había retrasado por unos problemas en la agenda de Arnold Schwarzenegger. Desde la Fox le prometieron que si Terminator era un éxito sería el director de la secuela de Alien. Tanto Cameron como su mujer y productora Gale Anne Hurd dieron por buena la promesa. “Cuando comienzas en Hollywood ayuda el ser ingenuo e idealista y creer que la gente dice la verdad”, comentaría años después Hurd.

Verdad sobrevenida o promesa cumplida, el caso es que, con el éxito de Terminator  bajo el brazo, Cameron recibió mando en plaza y se puso al frente de Aliens con una serie de nociones claras. La primera de ellas, que carecía de sentido imitar a la primera entrega, ese mundo opresivo creado por genios como Giger y Moebius. Así que optó por reconvertir la secuela en una película de guerra en la que emplearía con sutilidad iconografía del conflicto de Vietnam, aún tan presente en el subconsciente colectivo. Eran los años en los que Bruce Springsteen versioneaba el clásico ‘War’ de The Temptations, en los que Oliver Stone preparaba Platoon mientras se sucedían toda clase de subproductos de diferente pelaje en torno al conflicto.

No existían dudas al respecto de la protagonista ni del regreso de Ripley. Cameron era un fan de la película y del personaje de Weaver; de hecho en su cine se ha significado por la presencia habitual de personajes femeninos con entidad. Pero había un problema: Weaver, que se hallaba rodando en París La calle de la media luna (Bob Swaim, 1986) con Michael Caine, no tenía ni idea de que existiera una secuela en ciernes. Nadie en esos seis años se había dignado a contarle nada. Ella se veía que tenía frente a sí un guión acabado y un plazo para empezar a rodar: tres meses. En todo ese tiempo, además, Weaver se había convertido una estrella gracias a éxitos como Los cazafantasmas (Ivan Reitman, 1984) por lo que la oferta económica debería ser más tentadora. Desde la Fox instaron a Cameron a reescribir el guión sin el personaje de Ripley, a lo que el canadiense se negó en redondo. Sería precisamente su visión la que convencería a Weaver, quien se avino a participar en la secuela tras superar sus recelos iniciales. Una vez la Fox aceptó sus pretensiones económicas la maquinaria se puso en marcha.

Syd Mead, quien venía de trabajar en películas como Tron (Steven Lisberger, 1982) o Blade Runner (Ridley Scott, 1982) fue el encargado del arte junto a Ron Cobb. El primero diseñó la nave, voluntariamente diferente a la Nostromo de la primera película, mientras que el segundo se ocupó de los vehículos utilitarios. Ambos crearon escuela y se anticiparon varias décadas, algo que se puede comprobar si se repasan algunas películas recientes en las que se imitan las naves y vehículos que se emplearon en Aliens. Una labor en la que se devino fundamental la participación de un profesional británico, el decorador y diseñador de producción Peter Lamont, con experiencia en la saga Bond, quien supo aprovechar material de desguace de la RAF para dar forma a los diseños de Mead y Cobb. Lamont fue imprescindible para llevar la película a buen puerto y se convirtió en uno de los pocos técnicos locales que colaboró.

Porque la relación con los profesionales británicos no resultó precisamente idílica, antes y durante el rodaje, que tuvo lugar en los estudios Pinewood. Muchos de los técnicos eran profundos admiradores de Scott, una gloria del cine inglés, y veían con malos ojos a ese joven canadiense (Cameron entonces tenía 31 años) egocéntrico, malhablado y malcarado a quien consideraban un advenedizo. Especialmente significativos fueron los encontronazos con el ayudante de dirección Derek Cracknell, quien se consideraba más capacitado para dirigir la película que el canadiense. Tal y como recoge la biografía sobre Cameron The Futurist de Rebecca Keegan, el director de Terminator resumía el enfrentamiento con una descripción desoladora: “El equipo de Pinewood era perezoso, insolente y arrogante. Había algunas luces brillantes entre los jóvenes del departamento de arte, pero en su mayor parte, los despreciamos y nos despreciaron”, explicaba.

Uno de los conflictos se produjo por los peculiares horarios de los trabajadores de Pinewood. Si Cameron hubiera sido un poco más cinéfilo y se hubiera leído el diario del rodaje de Farenheit 451 (1966) que redactó François Truffaut, la situación no le habría pillado de improviso. En función de un peculiar convenio, los trabajadores de Pinewood tenían por costumbre parar a las 10 y a las 2 para tomar el té. Eso provocó que Cameron se encontrara en ocasiones el estudio vacío, cuando se suponía que debían estar rodando. Finalmente, tras despedir al director de fotografía y a Cracknell, Cameron reunió a la plantilla y les pidió su colaboración, al tiempo que reclamó que quienes no quisieran estar ahí dieran un paso adelante y se marcharan. Tras dejar de manifiesto los británicos su hartazgo por las jornadas sin fin de Cameron, éste accedió a mostrarse más sensible a los horarios y ellos más predispuestos a arrimar el hombro.

Con los actores fue bastante más fácil. La selección fue exhaustiva y en ella eligieron a intérpretes con los que sentían una especial química. La mayoría de los que encarnaban a soldados participaron en un adiestramiento militar para dar más verismo a sus personajes; sólo faltaron Weaver (que estaba terminando de rodar La calle de la media luna), Paul Reiser y Michael Biehn, quien fue llamado a última hora, con el rodaje ya comenzado, para sustituir a James Remar. Este entrenamiento, como ha sucedido en otras películas, contribuyó a forjar unos lazos de unión entre el reparto. Asimismo Cameron se mostró abierto a las propuestas que algunos de ellos le lanzaron, como Lance Henriksen, quien le sugirió la secuencia del cuchillo y la mano para presentar a su personaje. La escena, apenas un minuto de duración, es una de las más recordadas de la película.

La película cumplió su calendario, al final, pese a todo, y con un presupuesto de 18 millones de dólares estuvo a punto para la temporada de verano de 1986 en los cines estadounidenses y canadienses. A España, ya se ha dicho, no llegó hasta otoño. El argumento sedujo por ser una progresión lógica del original. En el satélite en el que fue encontrado el primer Alien se había instalado una colonia de humanos. Al perder el contacto con la colonia, la Corporación propietaria de la misma decide enviar una unidad de Infantería del Ejército y un ejecutivo para explorar la situación, comitiva a la que se incorpora a Ripley como asesora.

Cuando llegan allí descubren que los aliens se han hecho con el control de la colonia matando a todos los humanos. Lo que sigue a continuación es una sucesión de escenas de tensión, a cada cual más emocionante, que evidencian la maestría de Cameron como director de cine de aventuras. Impactante, emotiva, las peleas se suceden como en una guerra interminable, de sorpresa en sorpresa hasta la batalla final. La pasional interpretación de todo el reparto, con una Weaver en estado de gracia, contribuye a implicarse en la historia, con hallazgos como los personajes del androide Bishop que interpreta Henriksen, la dura Vásquez que encarnó la debutante Jenette Goldstein o el de un jovencísimo Bill Paxton.

Aliens, que en España llegó con el apellido El regreso, recaudó en todo el mundo 131 millones de dólares y ganó dos Oscars: el de mejores Efectos de Sonido y el de Efectos Visuales. Estuvo nominada a otros cinco, entre los que sobresalió la candidatura a Weaver como mejor actriz. Si es raro que una actriz sea nominada por una comedia, que lo sea por un film de ciencia ficción es casi milagroso. Desde el principio fue alabada por la crítica (¿quién dijo que a los críticos sólo les gusta el cine aburrido?) y en la página de referencia IMDB se encuentra en el puesto 65 de las mejores películas de la historia. Fue tal su éxito que se hizo inevitable la puesta en marcha de una saga que no ha vuelto a rayar a esta altura desde entonces, pese a que por ella han pasado talentos de la talla de David Fincher o Jean-Pierre Jeunet, y que ha retomado el propio Scott con una nueva saga, ampliando aún más el universo de los xenomorfos.

Este verano en el marco de la Comic-Con de San Diego se celebró un reencuentro del reparto con Cameron y Hurd, con motivo del 30 aniversario de la película. Asimismo, al director se le ha entrevistado por esta onomástica en diferentes medios. En una de estas entrevistas el canadiense recordaba cómo antes de rodar todo el mundo le recomendaba que no hiciera la película porque todo lo bueno de su secuela se asociaría a Scott y todo lo malo a él. Lo importante es que él tenía una visión, era propia, y funcionó. Tanto que hasta sido elogiada por el propio Scott. En la edición ilustrada del guión de Aliens se recogía la valoración del padre de la criatura. “No hay ninguna duda de que Cameron hizo un excelente film. Es realmente un logro”, aseguraba el autor de Blade Runner. Un logro que, curiosamente, comenzó como una pregunta de cortesía en un parking. Los grandes ríos tienen nacimientos pequeños.

¿MAGIA O ROBO? O COMO UNA PATATA PASA DE 0,12 €/kg (PRODUCTOR) A 1,25 €/kg (CONSUMIDOR)

http://www.masquemaquina.com/2016/11/magia-o-robo-o-como-una-patata-pasa-de.html?m=1

Recogiendo patatas

EN REALIDAD YO NO ENTIENDO NADA

Así es, yo no entiendo nada. No soy ningún experto en mercados agrarios, pero como productor y también como consumidor me «chirría» que vayas al «super» y encuentres como una patata, variedad Monalisa, la oferta una gran superficie a 1,25 €/kg pero esa misma patata se la han pagado al productor a 0,12 €/kg. ¿O yo estoy loco, que puede ser, o es que en realidad hay muchos «locos», posiblemente «piratas» o hablando claro, sinvergüenzas?

¿Cómo es posible que un producto que no se manipula, que como mucho se lava y se envasa en red o plástico agujereado se multiplique por 10 desde su parcela a la tienda?

¿Y la naranja qué?: He iniciado el tema con la patata como podía haberla iniciado con la naranja o la mandarina o decenas de productos que no requieren ningún tipo de manipulación: pimiento, melones y sandías, ajos y cebollas, tomates, almendra, judías, lentejas y garbanzos…

Parcelas de cebollas abandonadas sin recoger (las pagan a menos de 5 céntimos y en tienda a 0,65 o 1 €/kg…), campos de melones pisoteados por el propio agricultor, ídem con tomates, ajos…

Vídeo

¿Quién controla todo esto? Legalidad, ética y pecado

Yo tengo que reconocer que me pierdo en este intrincado laberinto de intereses. Ya lo he dicho, no soy ningún experto y ni tan siquiera un estudioso del tema, pero «me chirría«.
Siguiendo con la patata, puedo llegar a entender que hace un par de años se pagase a 7 o 9 céntimos y este año sobre 0,30; 0,45 e incluso 0,60 €/kg en función de variedades y calidades, pero me vuelve a «chirriar» que en una misma campaña tras esos 0,30 €/kg en apenas unos días se desplome a 0,12 €/kg (y ojo porque en las ventas por contrato previo apenas se ha rondado toda la campaña sobre esos 12 o 13 céntimos)
Seré demasiado obtuso, pero no puedo entenderlo y tengo tendencia a ver «fantasmas» o lo que es lo mismo, entiendo que hay suficiente poder para manipular los mercados, para meter miedo a los productores para que vendan, casi a cualquier precio, sus producciones (sobre todo teniendo en cuenta que hace unas semanas España se ha visto inmersa en unas lluvias que añadidas a la falta de capacidad de almacenamiento de los productores y unos cuantos rumores sobre cierre de demanda se precipite en la manipulación de precios en origen) No puedo creer que haya tantas fluctuaciones en la demanda mundial e insisto que más bien creo en intereses y codicia que en la propia ley de oferta y demanda.

¿Esto es legal? Posiblemente lo sea. Así es la economía en la “aldea global”. Aquí todo vale, pero ¿es ético? En cualquier caso seguro que es pecado.

Algunos números: Solamente revisando el completo informe semanal del Magrama(26 de octubre del 2016) los «chirríos» son constantes:

• La clementina a 0,253 €/kg
• La naranja a 0,173 €/kg
• La ya nombrada patata a 0,217 €/kg
• El tomate liso a 0,61 €/kg…

DE CUENTAS

Sigo con la patata y voy a hacer mis propios números. Son números «muy gordos» pero alguien tiene que mojarse y si me equivoco en los números, repito que son «gordos», que alguien me corrija.

• Patata en origen colocada en sacas: 0,12 €/kg = 20 pts/kg
• Transporte hasta almacenista (100 km): 4-5 pts/kg
• Manipulación y envasado: 6-9 pts/kg

Coste mayorista: suma costes anteriores 30 a 34 pts/kg

• Margen comercial almacenista (15 %): 34 a 39 pts/kg
• Transporte hasta mayorista (80 km): 3 – 4 pts/kg
• Margen comercial mayorista (15 %): 43 a 50 pts/kg = Coste a vendedor

Coste vendedor: 43 a 50 pts/kg

• Margen comercial frutería (30 %): 56 a 65 pts/kg es decir 0,34 a 0,39 €/kg

Coste a consumidor final: 56 a 65 pts/kg o 0,34 a 0,39 €/kg

Entonces ¿quién se queda con lo que falta hasta la oferta de 1,25 €/kg? ¿Dónde están los 85 o 90 céntimos que faltan?

Además este “burdo” no coincide exactamente con los precios publicados por Mercasa (precios a mayoristas) pues Mercasa marca, última semana, un precio a mayorista entre 0,29 €/kg (patata común) y 0,38 €/kg (patata calidad) pero se acerca.

«Magia» o ¿como pasar de 0,05 a 0,65 €/kg? (foto 2/11/2016)

También Mercasa ofrece la evolución desde origen a destino y da unas cifras de 0,28 €/kg (origen) mayorista 0,43 €/kg y consumidor 1,06 €/kg Aquí ya hay diferencias en un producto tan sensible en precio como es la patata y donde unos pocos céntimos marca la diferencia entre una campaña aceptable a un desastre para el productor. Un productor con un rendimiento de 40000 a 50000 kg/ha significa que 0,05 €/kg le supone más de 2000 €/ha a su producción.

¿Pero quien gana? ¿La pequeña tienda, los mayoristas, quién?

No creo que sea la pequeña tienda porque sé de buena tinta que una pequeña tienda no compra la patata al precio que yo digo en la tabla de 43-50 pts/kg si no bastante más. La pregunta entonces es ¿cuál es el margen del mayorista? ¿a cómo compran las grandes cadenas de distribución? ¿Está aquí el “eslabón perdido? ¿son los que tienen capacidad para manipular un mercado, almacenar producto y sacarlo cuando quieren los que de verdad ganan?

Cadena de valor desequilibrada: Mi opinión es que el mal está en los intermediarios, en una cadena de valor que no tiene ningún equilibrio, que son personas que no han visto el campo ni en pintura y que sólo les preocupa obtener beneficios para sus empresas y para si mismos.
No importa el origen de la patata, si los franceses empiezan a sacar sus patatas en octubre a los españoles que las cogen en ese mes que les den morcilla pero sin embargo cuando Murcia empieza a recoger su patata temprana tampoco la pagan mucho más cara porque tienen sus reservas en las cámaras… Siempre ganancias para los mismos, «chirridos«.

Naranja (1,1 €/kg) y tomate (1,29 €/kg) (Foto 2/11/2016)

¿Y LA ADMINISTRACIÓN, ADEMÁS DE COBRAR Y LUCIR PALMITO NO DEBEN HACER ALGO MÁS?

Pues quizá lo hacen pero…: La Administración no puede estar “a verlas venir”. Unas instituciones macromayoradas encabezadas por Ministerios como Agricultura y Alimentación, Sanidad (antes también Consumo…) Economía y Competitividad… y de ahí a las diversas Consejerías de las numerosas Comunidades Autónomas, Institutos y cientos de organismos que seguro que en su interior hacen algo pero pardiez si desde fuera sabemos lo que hacen por la ciudadanía dentro de sus bonitas y cómodas paredes. Supongo que entre sus bien nutridas filas de técnicos funcionarios habrá sapiencia, tiempo y ganas suficientes para investigar un mercado tan sencillo de seguir como qué ocurre y cómo evoluciona el precio de una patata, de un tomate y de un pepino, desde la tierra hasta la mesa.

Y que se hace algo estoy seguro pero repito, «la mujer del Cesar además de ser honrada debe parecerlo»

Me hago eco de una noticia del diario El País en referencia al expediente sancionador que actualmente el Ministerio de Agricultura tiene con Dia y Eroski, la causa es por un pacto de compras que puede infringir la Ley de la Cadena Alimentaria. Sin embargo la Comisión Nacional de los Mercados y la Competencia (CNMC) es partidaria del archivo del expediente que en su día presentó Promarca y la Federación de Industrias de Alimentación y Bebidas. Este es un buen ejemplo de dos políticas totalmente enfrentadas en el tablero de la gran distribución alimentaria.

POSIBLES SOLUCIONES

Unión de productores

No me estoy refiriendo a las cooperativas que en mayor o menos medida funcionan sino en la unión de cooperativas de diferentes regiones.

En el caso particular de la patata, un producto que en realidad se está recogiendo más de 6 meses en España pues se empieza a primeros de mayo en Murcia y Cartagena y luego se pasa a Andalucía para seguir en Toledo, Albacete y así ir subiendo hasta llegar a Ávila, Salamanca, Valladolid… ¿no se podrían unir los productores para tener una entrega al mercado escalonada?

Sin peajes

Naranja: del árbol a la mesa

Del productor al consumidor: No sé si esto puede ser una solución. Al final estas iniciativas funcionan pero a pequeña escala pero dar así salida a una producción tan elevada como la de patatas, melones, tomates…

Sería lo ideal pues así el consumidor sabría lo que conlleva la producción, se abaratarían los precios y se llevaría un producto seguramente más sano a las mesas pero en realidad lo veo utópico, ajeno a la realidad.

De todas formas abogo por que se analicen estas soluciones. Ahora hay armas importantes, contundentes, las que no había hace unas decenas de años. Están las web´s, las redes de comunicación pueden convertirse en redes de abastecimiento.
Mirad el artículo de La Huerta Digital que puede ilustrar nuevas formas de comercio como la iniciativa de Agroboca.

¡Gran Oferta! Patatas en la gran superficie (foto 2/11/2016)

Redes sociales ¿sirven para algo?

Pues deberían porque si no se convierten en «gallinero de cacareo» Hoy los agricultores han encontrado en las redes sociales un buen sistema para comunicarnos nuestras experiencias. Grupos tan numerosos como el de Agricultura Española o el de Amantes de los tractores ambos con más de 30.000 miembros pero también muchos otros deberían tener poder para denunciar los abusos.

Veremos si las redes sociales contribuyen a encauzar la «justicia» para el productor

Creo que esto si lo sabemos encauzar es bueno. Si no, una vez más, seguiremos siendo, con perdón, borregos y cada vez que vayamos a un súper nos taparemos los oídos para no escuchar los «chirridos» del campo 😒

Las 10 empresas que controlan el mercado de la alimentación procesada